Cyber-Physical Systems as General Distributed Parameter Systems:Three Types of Fractional Order Models and Emerging Research Opportunities

Fudong Ge,YangQuan Chen,Senior Member,IEEE,and Chunhai Kou

Cyber-Physical Systems as General Distributed Parameter Systems:Three Types of Fractional Order Models and Emerging Research Opportunities

Fudong Ge,YangQuan Chen,Senior Member,IEEE,and Chunhai Kou

—Cyber-physical systems(CPSs)are man-made complex systems coupled with natural processes that,as a whole, should be described by distributed parameter systems(DPSs)in general forms.This paper presents three such general models for generalized DPSs that can be used to characterize complex CPSs.These three different types of fractional operators based DPS models are:fractional Laplacian operator,fractional power of operator or fractional derivative.This research investigation is motivated by many fractional order models describing natural,physical,and anomalous phenomena,such as sub-diffusion process or super-diffusion process.The relationships among these three different operators are explored and explained.Several potential future research opportunities are then articulated followed by some conclusions and remarks.

Index Terms—Cyber-physical systems(CPSs),generalized distributed parameter systems(DPSs),fractional Laplacian operator,fractional power of operator,fractional derivative.

I.INTRODUCTION

I T is well known that the cyber-physical systems(CPSs) with integrated computational and physical processes can be regarded as a new generation of control systems and can interact with humans through many new modalities[1].The objective of CPS is to develop new science and engineering methods in which cyber and physical designs are compatible, synergistic,and integrated at all scales.Besides,as we all know,the distributed parameter systems(DPSs)can be used to well characterize those cyber-physical process[2-3]and the actions and measurements of the system studied are better described by utilizing the actuators and sensors,which was first introduced by El Jai and Pritchard in[4]and mainly focused on the locations,number and spatial distributions of the actuators and sensors.

Moreover,in the past several decades,fractional calculus has shown great potential in science and engineering appli-cations and some phenomena such as self-similarity,nonstationary,non-Gaussian process and short or long memory process are all closely related to fractional calculus[5-7].It is now widely believed that,using fractional calculus in modeling can better capture the complex dynamics of natural and man-made systems,and fractional order controls can offer better performance not achievable before using integer order controls[8-9].

Fudong Ge is with the College of Information Science and Technology, Donghua University,Shanghai 201620,China(e-mail:gefd2011@gmail.com).

YangQuan Chen is with the Mechatronics,Embedded Systems and Automation Lab,University of California,Merced,CA 95343,USA(e-mail: ychen53@ucmerced.edu).

Chunhai Kou is with the Department of Applied Mathematics,Donghua University,Shanghai 201620,China(e-mail:kouchunhai@dhu.edu.cn).

Motivated by the above arguments,in this paper,let Ω be an open bounded subset of Rnwith smooth boundary∂Ω and we consider the following fractional DPSs:

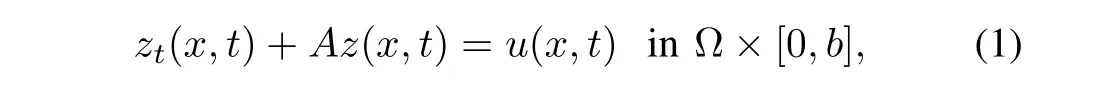
where b＞0 is a given constant,u is the control input depending on the number and the structure of actuators and A may be a fractional Laplacian operator,a fractional power of operator or a fractional derivative.

The contribution of this present paper is to analyze the relationship among the fractional Laplacian operator,fractional power of operator and fractional derivative and try to explore the opportunities and research challenges related to the fractional order DPSs emerging at the same time.To the best of our knowledge,no result is available on this topic.We hope that the results here could provide some insights into the control theory of this field and be used in real-life applications.

The rest of the paper is organized as follows.The relationship among fractional Laplacian operator,fractional power of operator and fractional derivative are explored in Section II.In Section III,the emerging research opportunities of the fractional order DPSs with those three operators are discussed.Several conclusions and remarks of this paper are given in the last section.

II.THREE DIFFERENT TYPES OF OPERATORS

In this section,we shall introduce some basic relationships among the fractional Laplacian operator,fractional power of operator and fractional derivative.For further information,we refer the readers to papers from[10]to[36]in the reference section of the present paper and the references cited therein.

A.Fractional Laplacian Operator and Fractional Power of Operator

This subsection is devoted to the difference between the fractional Laplacian operator and fractional power of operator. For more details,please see[10-15]and their cited references.

Let us denote(-△)α/2the nonlocal operator(also called the fractional Laplacian operator)defined pointwise by the following Cauchy principal value integral

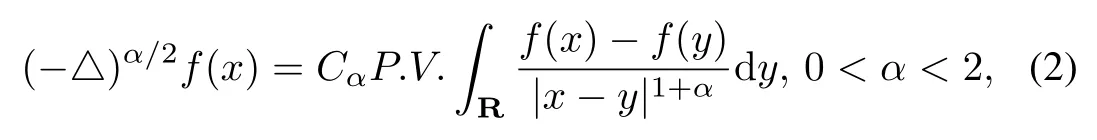
Theorem 1[21].Suppose that(-△)α/2is defined in L2(0,l) for α∈(0,2).Then,the eigenvalues of the following spectral problem

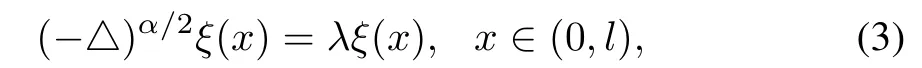
where ξ∈L2(0,l)is extended to all R by 0 is

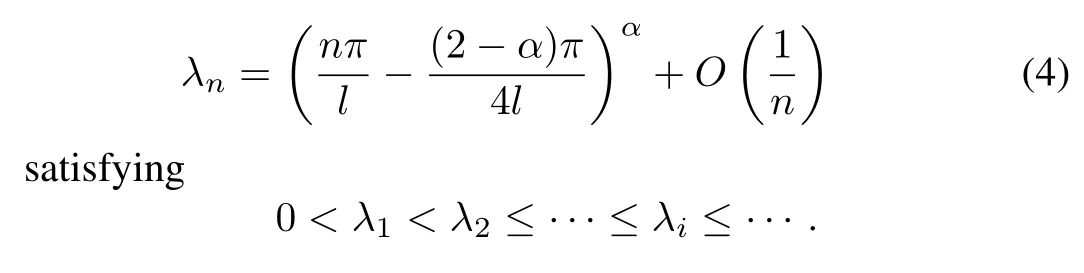
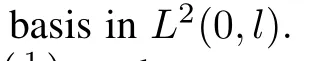
However,for a positive operator A on bounded domain [0,l],suppose 0＜λ1≤λ2≤···≤λn≤···are the eigenvalues of A,{ξ1,ξ2,...,ξn,...}are the corresponding eigenfunctions and ξn(i=1,2,...)form an orthonormal basis of L2(0,l).Let(·,·)be the inner product of L2(0,l). We define the fractional power of operator A as follows:

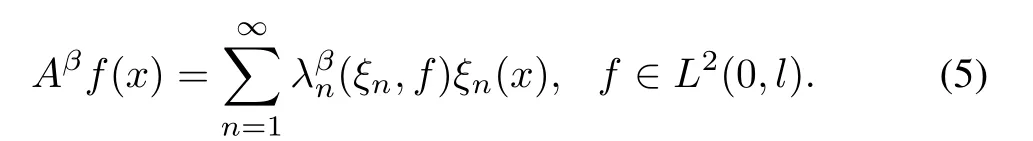
Moreover,the work spaces of the two operators(fractional Laplacian operator and fractional power of operator A)are different.Before stating our main results,we first introduce two Banach spaces,which are specified in[11,13,14,16].

For Ω⊆Rnis a bounded domain,s∈(0,1)and p∈[1,∞),we define the classical Sobolev space Ws,p(Ω) as follows[13]:

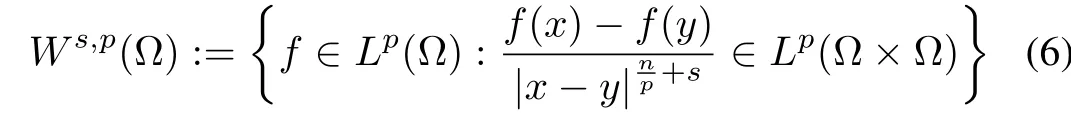
endowed with the natural norm

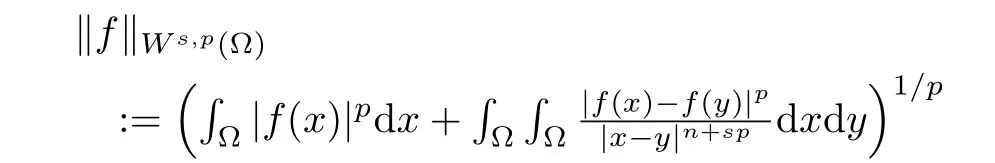
is an intermediary Banach space between Lp(Ω)and W1,p(Ω). When a non-integer s＞1,let s=m+σ with m∈N and σ∈(0,1).In this case,let Dβf with|β|=m be the distributed derivative of f,then the classical Sobolev space Ws,p(Ω)defined by

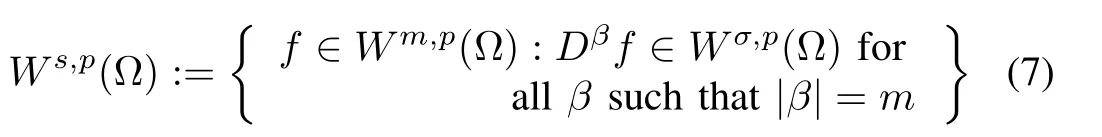
with respect to the norm

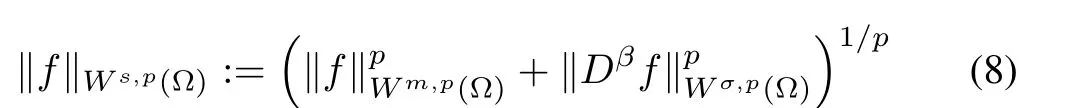
is a Banach space.Clearly,if s=m is an integer,the space Ws,p(Ω)coincides with the Sobolev space Wm,p(Ω).

with the norm

Besides,it is well known that

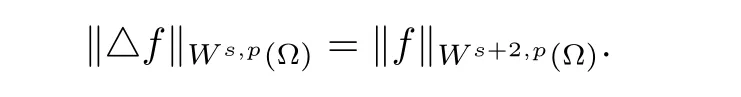
But for the fractional Laplacian operator(-△)α,

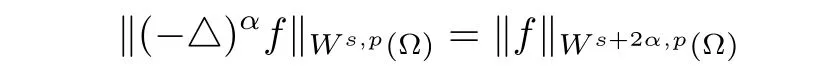
will not hold.By using Fourier transform,we have

Finally,by[15],let X be a rotationally invariant stable process of index α∈(0,1).Its symbol is given by η(f)=-|f|αfor all f∈Rn.It is instructive to accept that η is the symbol for a legitimate differential operator;then,using the usual correspondence fj→-i∂jfor 1≤j≤n,we would write

In fact,it is very useful to interpret η(Ω)as a fractional power of the operator-△.However,for the fractional Laplacian operator,it can be defined as the generator of α-stable L´e vy processes.More precisely,if Xtis the isotropic α-stable L´e vy processes starting at zero and f is a smooth function,then

This also indicates that the fractional power of operator-△and the fractional Laplacian operator are different.

B.Fractional Laplacian Operator and Fractional Derivative

This subsection is focused on the relationship between the fractional Laplacian operator and the Riesz fractional derivative.

Definition 1[23].The Riesz fractional operator for n-1＜α≤n on a finite interval 0≤x≤l is defined as

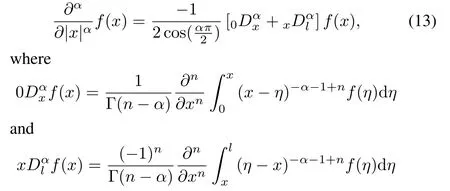
are the left-sided and right-sided Riemann-Liouville fractional derivative,respectively.

Moreover,according to[24],the fractional Laplacian is the operator with symbol|x|α.In other words,the following formula holds:

where F and F-1denote the Fourier transform and inverse Fourier transform of f(x),respectively.We refer the readers to[25]for a detailed proof of the equivalence between the two definitions(1)and(2)of fractional Laplacian operator.

Lemma 1[27].For a function f(x)defined on the finite domain[0,l],and f(0)=f(l)=0,the following equality holds:

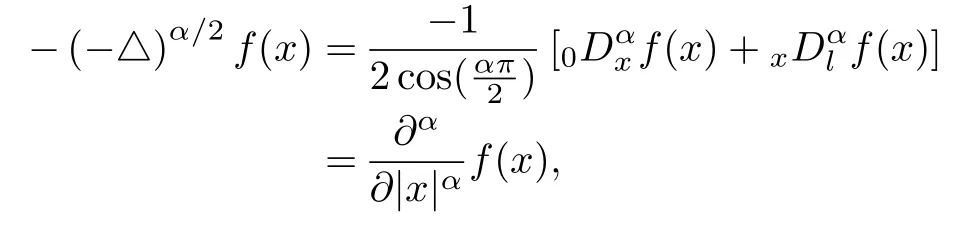
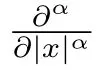
For more information on the analytical solution of the generalized multi-term time and space fractional partial differential equations with Dirichlet nonhomogeneous boundary conditions,we refer the readers to[27].For more information on the numerical solution of fractional partial differential equation with Riesz space fractional derivatives on a finite domain,consult[28-29]. C.Fractional Derivative and Fractional Power of Operator

In this part,we first show the following definition of the positive operator.

Definition 2[30].The operator A is said to be positive if its spectrum σ(A)lies in the interior of the sector of angle φ∈(0,π),symmetric with respect to the real axis,and if on the edges of this sector,S1={ρeiφ:0≤ρ＜∞}and S2={ρe-iφ:0≤ρ＜∞},and outside it the resolvent (λI-A)-1is subject to the bound

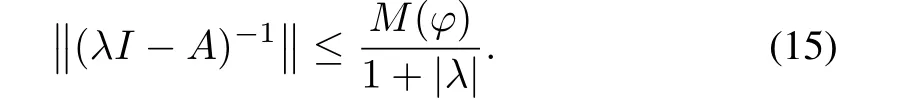
Moreover,for a positive operator A,any α＞0,one can define the negative fractional power of operator A by the following formula[31]:

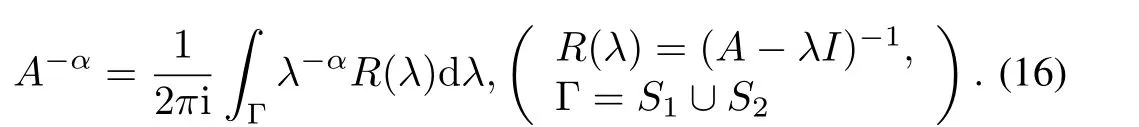
It is then quite easy to see that A-αis a bounded operator, which is an entire function of α,satisfying A-α=A-nif α is an integer n,and A-(α+β)=A-αA-βfor all α,β∈C. Using(16),we have

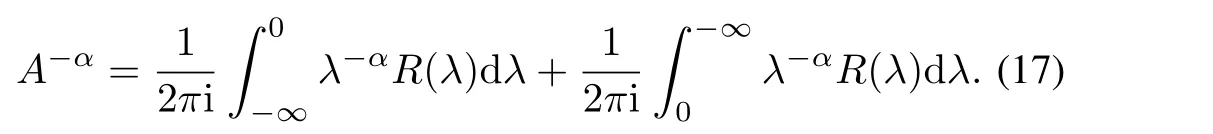
Then taking the integration along the lower and upper sides of the cut respectively:λ=s e-πiand λ=s eπi,it follows that

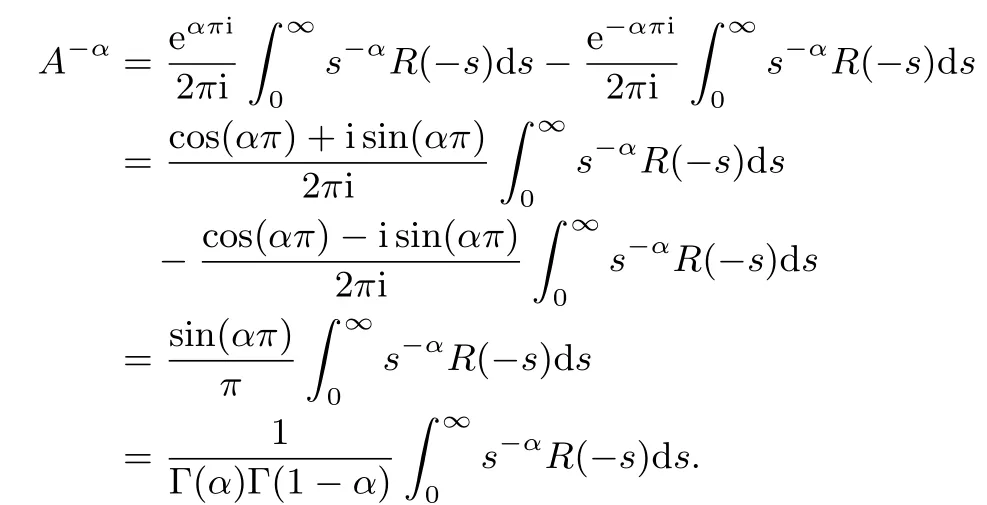
Moreover,for any α∈(n-1,n),we get that

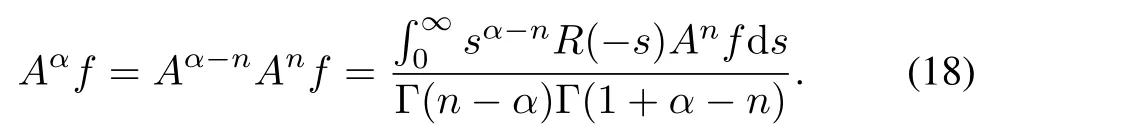
and for more properties on the fractional power of a positive operator,please see[32-35]and the references cited therein.

Now we are ready to state the following results on the connection of fractional derivative and integral with fractional power of positive operator.

Theorem 2.Let the absolutely space

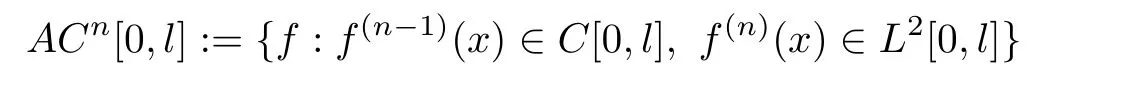
and let A be the operator defined by the formula Af(x)= f′(x)with the domain

Then A is a positive operator in the Banach space ACn[0,l] andfor all f(x)∈D(A).

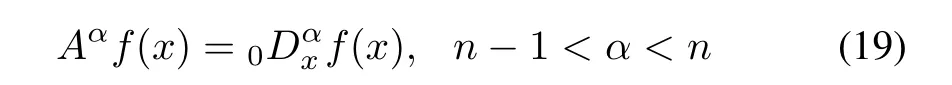
Proof.By[36],the operator A+sI(s≥0)has a bounded inverse and the resolvent of A is given by

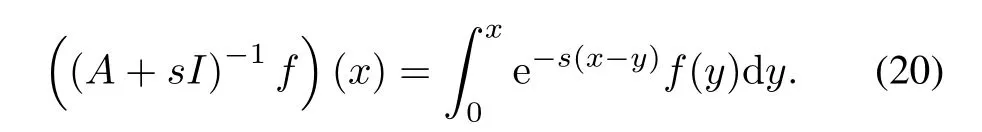
Then the operator A is a positive operator in ACn[0,l]and (17)gives

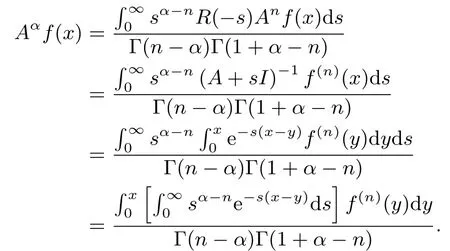
Let s(x-y)=λ,we get that

III.THE EMERGING RESEARCH OPPORT UNITIES

Recent advances in modeling and control of fractional diffusion systems,fractional reaction-diffusion systems and fractional reaction-diffusion-advection systems have been reviewed in the framework of CPSs.The fractional order DPSs have now been found wide applications for describing many physical phenomena,such as sub-diffusion or super-diffusion processes.At the same time,to our best knowledge,many problems are still open calling for research cooperation of multi-disciplines such as mathematical modelling,engineering applications,and information sciences.

First,it is worth noting that in the more recent monograph[37],the theory of pseudo-differential operators with singular symbols,and the connections between them and those three types of operators are explored.See[38-40]for more knowledge on pseudo-differential operator.Moreover, we claim that those equivalences between fractional Laplacian operator and fractional derivative,fractional order of operator and fractional derivative discussed in this paper can introduce new mathematical vehicles to study fractional order generalized DPSs.For example,when we study a fractional DPSs with Riesz fractional derivative,by Lemma 1 in Section II-B,the spectral representation methods can be used to characterize the solution of the dynamic system.Then we can study the existence of solutions,stability,controllability and observability of the system under consideration.

Potential topics such as modeling the sub-diffusion or superdiffusion processes with consideration of the networked mobile actuators and mobile sensors,the communication among the actuators and sensors,collocated or non-collocated actuators and sensors,their robustness and optimality problems are all interesting and worthy much more efforts in future.Another interesting and important topic concerns the time-space fractional DPSs where the traditional first order derivative is replaced by a fractional order derivative with respect to the time t.

Furthermore,in the case of diffusion systems,it is worth mentioning that,in general,not all the states can be reached in the whole domain of interest[4,41-42]and it would be more challenging in nature since the dynamics of the reallife control problem is always hybrid continuous and discrete. Due to the spatial-temporal sampling and discrete nature of decision and control,the notions of regional analysis should be introduced,i.e.,we can consider the regional stability, regional controllability,regional observability etc.of the system under consideration.In addition,as stated in[43],from an application point of view,some plain questions such as“How many actuators/sensors are sufficient and how to best configure them for a fractional DPSs control process?”,“Given the desirable zone shape,is it possible to control or contain the fractional diffusion process within the given zone?”,if not,“How to quantify the controllability/observability of the actuators/sensors”and etc.might be asked,which in fact raises some important theoretical challenges and open new opportunities for further research.

IV.CONCLUSIO N

This paper is concerned with the fractional order DPSs with three different operators:fractional Laplacian operator, fractional power of operator and fractional derivative.The relationship among the three operators and the emerging research opportunities are introduced.We hope that the results here could provided some insight into the control theory analysis of fractional order DPSs in particular and CPSs in general. The results presented here can also be extended to complex fractional order DPSs and various open questions are still pending.For instance,the problem of regional optimal control of fractional order DPSs with more complicated sensing and actuation configurations are of great interest.

REFERENCES

[1]Lee E A.Cyber physical systems:design challenges.In:Proceedings of the 11th IEEE International Symposium on Object Oriented Real-Time Distributed Computing(ISORC).Orlando,FL:IEEE,2008.363-369

[2]Song Z,Chen Y Q,Sastry C R,Tas N C.OptimalObservationforCyberphysical Systems:a Fisher-information-matrix-based Approach.London: Springer,2009.

[3]Tricaud C,Chen Y Q.Optimal Mobile Sensing and Actuation Policies in Cyber-physical Systems.London:Springer,2012.

[4]El Jai A,Pritchard A J.Sensors and Controls in the Analysis of Distributed Systems.New York:Halsted Press,1988.

[5]Podlubny I.Fractional Differential Equations,Vol.198.New York: Academic Press,1999.

[6]Kilbas A A,Srivastava H M,Trujillo J J.Theory and Applications of Fractional Differential Equations.Amsterdam,Netherlands:Elsevier Science,2006.

[7]Klimek M.On Solutions of Linear Fractional Differential Equations of a Variational Type.Czestochowa:Publishing Office of Czestochowa University of Technology,2009.

[8]Torvik P J,Bagley R L.On the appearance of the fractional derivative in the behavior of real materials.Journal of Applied Mechanics,1984, 51(2):294-298

[9]Mandelbrot B B.The Fractal Geometry of Nature,Vol.173.San Francisco:W.H.Freeman,1983.

[10]Lv G Y,Duan J Q,He J C.Nonlocal elliptic equations involving measures.Journal of Mathematical Analysis and Applications,2015, 432(2):1106-1118

[11]Lv G Y,Duan J Q.Martingale and weak solutions for a stochastic nonlocal Burgers equation on bounded intervals.arXiv preprint arXiv: 1410.7691[math.P R],October 28,2014.

[12]He J C,Duan J Q,Gao H J.Global solutions for a nonlocal Ginzberg-Landau equation and a nonlocal Fokker-Plank equation.arXiv preprint arXiv:1312.5836[math.A P],December 20,2013.

[13]Di Nezza E,Palatucci G,Valdinoci E.Hitchhiker’s guide to the fractional Sobolev spaces.Bulletin des Sciences Math´ematiques,2012,136(5): 521-573

[14]Du Q,Gunzburger M,Lehoucq R B,Zhou K.A nonlocal vector calculus,nonlocal volume-constrained problems,and nonlocal balance laws.Mathematical Models and Methods in Applied Sciences,2013, 23(3):493-540

[15]Applebaum D.L´evy Processes and Stochastic Calculus(Second edition). Cambridge:Cambridge University Press,2009.

[16]Du Q,Gunzburger M,Lehoucq R B,Zhou K.Analysis and approximation of nonlocal diffusion problems with volume constraints.SIAM Review,2012,54(4):667-696

[17]Choi W,Kim S,Lee K A.Asymptotic behavior of solutions for nonlinear elliptic problems with the fractional Laplacian.Journal of Functional Analysis,2014,266(11):6531-6598

[18]Barrios B,Colorado E,De Pablo A,S´anchez U.On some critical problems for the fractional Laplacian operator.Journal of Differential Equations,2012,252(11):6133-6162

[19]Micu S,Zuazua E.On the controllability of a fractional order parabolic equation.SIAM Journal on Control and Optimization,2006,44(6): 1950-1972

[20]Fattorini H O,Russell D L.Exact controllability theorems for linear parabolic equations in one space dimension.Archive for Rational Mechanics and Analysis,1971,43(4):272-292

[21]Kwa´snicki M.Eigenvalues of the fractional Laplace operator in the interval.Journal of Functional Analysis,2012,262(5):2379-2402

[22]Ros-Oton X,Serra J.The dirichlet problem for the fractional Laplacian:regularity up to the boundary.Journal de Math´ematiques Pures et Appliqu´ees,2014,101(3):275-302

[23]Gorenflo R,Mainardi F.Random walk models for space-fractional diffusion processes.Fractional Calculus and Applied Analysis,1998, 1(2):167-191

[24]Samko S G,Kilbas A A,Marichev O I.Fractional Integrals and Derivatives:Theory and Applications.New York:Gordon and Breach Science Publishers,1993.

[25]Landkof N S,Doohovskoy A P.Foundations of Modern Potential Theory (Grundlehren der mathematischen Wissenschaften).New York:Springer, 1972.

[26]Luchko Y,Gorenflo R.An operational method for solving fractional differential equations with the Caputo derivatives.Acta Mathematica Vietnamica,1999,24(2):207-233

[27]Jiang H,Liu F,Turner I,Burrage K.Analytical solutions for the multiterm time-space Caputo-Riesz fractional advection-diffusion equations on a finite domain.Journal of Mathematical Analysis and Applications, 2012,389(2):1117-1127

[28]Yang Q,Liu F,Turner I.Numerical methods for fractional partial differential equations with Riesz space fractional derivatives.Applied Mathematical Modelling,2010,34(1):200-218

[29]Liu F,Anh V,Turner I.Numerical solution of the space fractional Fokker-Planck equation.Journal of Computational and Applied Mathematics,2004,166(1):209-219

[30]Ashyralyev A.A note on fractional derivatives and fractional powers of operators.Journal of Mathematical Analysis and Applications,2009, 357(1):232-236

[31]Komatsu H.Fractional powers of operators.Pacific Journal of Mathematics,1966,19(2):285-346

[32]Komatsu H.Fractional powers of operators,II.Interpolation spaces. Pacific Journal of Mathematics,1967,21(1):89-111

[33]Komatsu H.Fractional powers of operators,III.Negative powers.Journal of the Mathematical Society of Japan,1969,21(2):205-220

[34]Carracedo C M,Alix M S.TheTheoryofFractionalPowersofOperators. Amsterdam:Elsevier,2001.

[35]Balakrishnan A V.Fractional powers of closed operators and the semigroups generated by them.Pacific Journal of Mathematics,1960, 10(2):419-439

[36]Faris W G.Methods of applied mathematics second semester lecture notes[Online],available:http://math.arizona.edu/faris/methbweb/methlecb.pdf,January 2,2015.

[37]Umarov S.Introduction to Fractional and Pseudo-differential Equations with Singular Symbols,Vol.41.Switzerland:Springer,2015.

[38]Hahn M,Kobayashi K,Umarov S.SDEs driven by a time-changed L´evy process and their associated time-fractional order pseudo-differential equations.Journal of Theoretical Probability,2012,25(1):262-279

[39]Umarov S.Algebra of pseudo-differential operators with variable analytic symbols and propriety of the corresponding equations.Differential Equations,1991,27(6):753-759

[40]Hahn M,Umarov S.Fractional Fokker-Planck-Kolmogorov type equations and their associated stochastic differential equations.Fractional Calculus and Applied Analysis,2011,14(1):56-79

[41]AfifiL,Chafia A,El Jai A.Regionally efficient and strategic actuators. International Journal of Systems Science,2002,33(1):1-12

[42]Ge F D,Chen Y Q,Kou C H.Regional controllability of anomalous diffusion generated by the time fractional diffusion equations.In:Proceedings of the 2015 ASME International Design Engineering Technical Conferences(ASME IDETC/CIE 2015),Boston,2015,DETC2015-46697.See also:arXiv preprint arXiv:1508.00047

[43]Chen Y Q,Moore K L,Song Z.Diffusion boundary determination and zone control via mobile actuator-sensor networks(MAS-net):challenges and opportunities.In:Proceedinds of SPIE,Intelligent Computing: Theory and Applications II.Orlando,FL:SPIE,2004,5421:102-113

Fudong Ge Ph.D.candidate in the College of Information Science and Technology of Donghua University,China.He joined the MESA Lab of the University of California,Merced in October, 2014 as an exchange Ph.D.student hosted by Prof. YangQuan Chen.His research interests include existence,stability of solutions for fractional differential equations,continuous time random walks and anomalous diffusion systems,distributed measurement and distributed control problems in general distributed parameter systems or cyber-physical systems in general form.

YangQuan Chen earned his Ph.D.degree in advanced control and instrumentation from Nanyang Technological University,Singapore,in 1998.Dr. Chen was on the Faculty of Electrical and Computer Engineering at Utah State University before he joined the School of Engineering,University of California,Merced in 2012 where he teaches“Mechatronics”for juniors and“Fractional Order Mechanics”for graduates.His current research interests include mechatronics for sustainability,cognitive process control and hybrid lighting control, multi-UAV based cooperative multi-spectral“personal remote sensing”and applications,applied fractional calculus in controls,signal processing and energy informatics,distributed measurement and distributed control of distributed parameter systems using mobile actuator and sensor networks.Corresponding author of this paper.

Chunhai Kou received his Ph.D.degree from Shanghai Jiao Tong University,Shanghai,China, in 2002.He joined the Department of Science, Donghua University in 2004 where he teaches“Stability Analysis of Nonlinear Differential Equations”,“Theory and Applications of Fractional Differential Equations”and“Mathematical Analysis”.His current research interests include stability analysis of differential equations based on the Lyapunov theory,basic theory of differential inclusions,applied fractional calculus in controls,existence,stability of solutions for the fractional differential equations.

t

August 25,2015,accepted September 7,2015.This work was supported by Chinese Universities Scientific Fund(CUSF-DHD-2014061)and Natural Science Foundation of Shanghai(15ZR1400800). Recommended by Associate Editor Antonio Visioli.

:Fudong Ge,YangQuan Chen,Chunhai Kou.Cyber-physical systems as general distributed parameter systems:three types of fractional order models and emerging research opportunities.IEEE/CAA Journal of Automatica Sinica,2015,2(4):353-357