Three-dimensional adaptive finite-time guidance law for intercepting maneuvering targets
Yujie SI,Shenmin SONG
Center for Control Theory and Guidance Technology,Harbin Institute of Technology,Harbin 150001,China
Three-dimensional adaptive finite-time guidance law for intercepting maneuvering targets
Yujie SI,Shenmin SONG*
Center for Control Theory and Guidance Technology,Harbin Institute of Technology,Harbin 150001,China
Adaptive control; Finite-time guidance law; Impact angle; Sliding mode control; Three-dimensional guidance law
To intercept maneuvering targets at desired impact angles,a three-dimensional terminal guidance problem is investigated in this study.Because of a short terminal guidance time,a finitetime impact angle control guidance law is developed using the fast nonsingular terminal sliding mode control theory.However,the guidance law requires the upper bound of lumped uncertainty including target acceleration,which may not be accurately obtained.Therefore,by adopting a novel reaching law,an adaptive sliding mode guidance law is provided to release the drawback.At the same time,this method can accelerate the convergence rate and weaken the chattering phenomenon to a certain extent.In addition,another novel adaptive guidance law is also derived;this ensures systems asymptotic and finite-time stability without the knowledge of perturbations bounds.Numerical simulations have demonstrated that all the three guidance laws have effective performances and outperform the traditional terminal guidance laws.
1.Introduction
To improve the impact lethality of missiles,guidance laws with terminal impact angle constraints are necessary in modern warfare.1In the past decades,various methods have been developed in this field.2–7In Ref.2,unlike the conventional proportional navigation guidance law(PNGL),a new guidance law with a supplementary time-varying bias was developedto intercept targets at the desired impact angle.In Ref.3,for the problem of trajectory shaping against a stationary target,the biased pure PNGL(BPPNGL)was given.The control problem was solved indirectly by using the integrated form of the BPPNGL.In Ref.4,to intercept ground targets,a guidance law was developed for air-launched missiles using the nonlinear suboptimal method,and terminal impact angle constraints were accurately satisfied by applying this guidance law.In Refs.5,6,guidance laws were proposed against stationary targets using the optimal control method.In Ref.7,to obtain the expected interception angle for intercepting a slowmoving or stationary target,an interception angle control guidance law was formulated using a PNG-based method.
During the developments of the PNGL,the optimal method,and other traditional methods,a sliding mode control(SMC)method was proposed to provide a new way for designing guidance laws.Because the SMC method has good robustness to external disturbances and uncertainties of systematic parameters,it has been generally used in designing guidance laws in the recent decades.In Ref.8,guidance laws were developed using the traditional SMC.In Ref.9,an adaptive nonlinear guidance law was proposed by using the SMC method so that a missile can accurately hit a target at the desired impact angle.Thus,it can be concluded that the SMC method can only guarantee the asymptotic convergence of a system.
Because the terminal guidance phase lasts for only several seconds,the finite-time control theory has been extensively studied.Moreover,the finite-time stabilization of dynamic systems can contribute to both high accuracy and good performance.Notably,the terminal SMC(TSMC)is a relatively good method to solve this problem.In Refs.10,11, finite-time sliding mode guidance laws with impact angle constraints were proposed.Guidance laws have been developed using the nonsingular TSMC(NTSMC)theory in Refs.12,13.Using the NTSMC method, finite-time guidance laws with the desired impact angle were proposed.However,they were derived against stationary or constant-velocity targets.To intercept targets with impact angle constraints,guidance laws were proposed without exhibiting any singularity in Ref.14.In Ref.15,using the NTSMC theory,a strictly convergent guidance law was developed for missiles with impact angle constraints.In Ref.16,an optimal pulsed guidance law with a time-varying weighted quadratic cost function that enables the imposing of a predetermined intercept angle was developed.However,all the guidance laws in the above literature were developed in a two-dimensional(2D)scene,which would significantly reduce the guidance precision.
In practice,all the engagement scenarios of a missile intercepting targets are three-dimensional(3D)scenarios.However,most of the existing studies mainly concentrated on decoupling the 3D engagement into two mutually orthogonal 2D engagements,and their guidance laws were designed separately.This will definitely affect the guidance accuracy.Many studies also designed guidance laws in a 3D engagement.3D sliding mode guidance laws have been reported in Refs.17–19.However,these guidance laws were designed without considering impact angle constraints.In Refs.20–22,some novel guidance laws with impact angle constraints were proposed using SMC for 3D engagements.However,those guidance laws were designed without considering target maneuvering.
Along with high complexity of a battle field,rapid combat rhythm,and high-tech weaponry,an interceptor should also have the ability to intercept maneuvering targets.Therefore,it is necessary to devise 3D finite-time guidance laws against maneuvering targets with impact angle constraints.In Refs.19,23,24,to intercept maneuvering targets,novel 3D finite-time convergence guidance laws have been developed using the NTSMC theory.However,all of them did not consider the impact angle constraints problem.
Now,a few studies have designed 3D guidance laws simultaneously by considering the problems of finite-time convergence,terminal impact angle constraints,and maneuvering targets.Based on the summary of the abovementioned problems,the main objective of the paper is to construct finitetime guidance laws with impact angle constraints in a 3D engagement.The main contributions are as follows.(1)Based on the fast NTSMC method,a novel TSM guidance law is derived.Unlike the existing NTSMC methods that decouple a 3D engagement into two mutually orthogonal 2D engagements,12–15the guidance law is derived by using coupled 3D dynamic systems.Furthermore,the guidance law can also be applied to intercept maneuvering targets.(2)By introducing a novel reaching law designed in this study,an adaptive 3D nonsingular guidance law is developed.Compared to the existing 3D TSMC methods,20–22the guidance law can intercept maneuvering targets without the knowledge of target information.Compared to the existing 3D TSMC guidance laws against maneuvering targets,19,23,24this guidance law can not only successfully intercept a maneuvering target,but also intercept it from the desired angles.Moreover,the guidance law can accelerate the convergence rate and reduce the unwanted chattering level as well.(3)Another novel reaching law is derived.The adaptive guidance law designed based on this law ensures that the line-of-sight(LOS)angle and angular rate are asymptotically and finite-timestablewith unknown bounded disturbances.
The structure of this paper is as follows.In Section 2,nondecoupling 3D engagement dynamic systems are established.In Section 3,based on the fast NTSMC method,a novel guidance law is designed.Furthermore,two adaptive fast nonsingular terminal sliding-mode guidance laws are also derived by adopting novel reaching laws.Simulations are performed in Section 4.Conclusions are provided in Section 5.
2.Problem statement
Fig.1 shows the 3D homing guidance geometry.OIXIYIZIrepresents the inertial reference frame,and its origin is the missile launching point.OMXMYMZMand OTXTYTZTrepresent the missile and target velocity coordinate systems,respectively.R denotes the LOS distance.In this geometry,the missile velocity is denoted by Vm,and its direction is de fined by θmand φmwith respect to the LOS frame.Moreover,it is assumed that the missile flies at a constant speed in this study.The target flies at a constant speed Vt,and its direction is denoted by θtand φt. θLand φLdenote the LOS angles.Then,the 3D engagement dynamic systems can be expressed as follows:25


Fig.1 Geometry in a 3D space.


In the study,it is assumed that the signals R,˙R,θL,φL,˙θL,˙φL,θm,and φmcan be measured.Let θLfand φLfbe the desired LOS angles.Let x1and x2be the LOS angle errors,which are de fined by x1= θL-θLfand x2= φL-φLf.
To facilitate the design,the following lemmas and assumptions are considered for further application.
Lemma 126.Consider the nonlinear systemAssume the existence of a continuous and positive de finite function V(x),

where μ,λ > 0,and 0< α < 1 are constants.x(t0)=x0,in which t0is the initial time.Then,the time of system states arriving at the equilibrium point T satis fies the following inequality:

That is,the system states are finite-time convergent.
Lemma 227.Consider the system˙x=f(x,t),x∈Rn.If V(x)is a continuous and positive definite function(de fined on U∈Rn),andis negative semidefinite on U∈Rnfor τ∈ (0,1)and ζ∈ R+,then an area U0∈ Rnexists so that any V(x)starting from U0∈Rncan reach V(x)≡0 in finite time.Moreover,if Tris the time needed to reach V(x)≡0,thenwhere V(x0)is the initial value of V(x).
Lemma 328.For bi∈ R,i=1,2,···,n,if q is a real number and 0<q<1,then the following inequality can be satisfied:

Assumption 1.Assume that the target accelerations,aytand azt,are bounded and satisfy|ayt|≤ a1,|azt|≤ a2,for all t≥0,where a1and a2are the upper bounds of the target accelerations.
Assumption 229.Assume that the missile intercepting the target by impact(‘hit-to-kill”)occurs whenbut belongs to the interval[Rmin,Rmax]=[0.1,0.25]m.
In this study,guidance laws with impact angle constraints are designed by using nonlinear engagement dynamics,as shown in Eqs.(1)–(7).Moreover,guidance laws are also developed for intercepting maneuvering targets.Second-order dynamic systems between the control input and the LOS angles can be obtained by differentiating Eqs.(2)and(3)as

In the notational form,the dynamics,expressed using Eqs.(11)and(12),can also be rewritten as
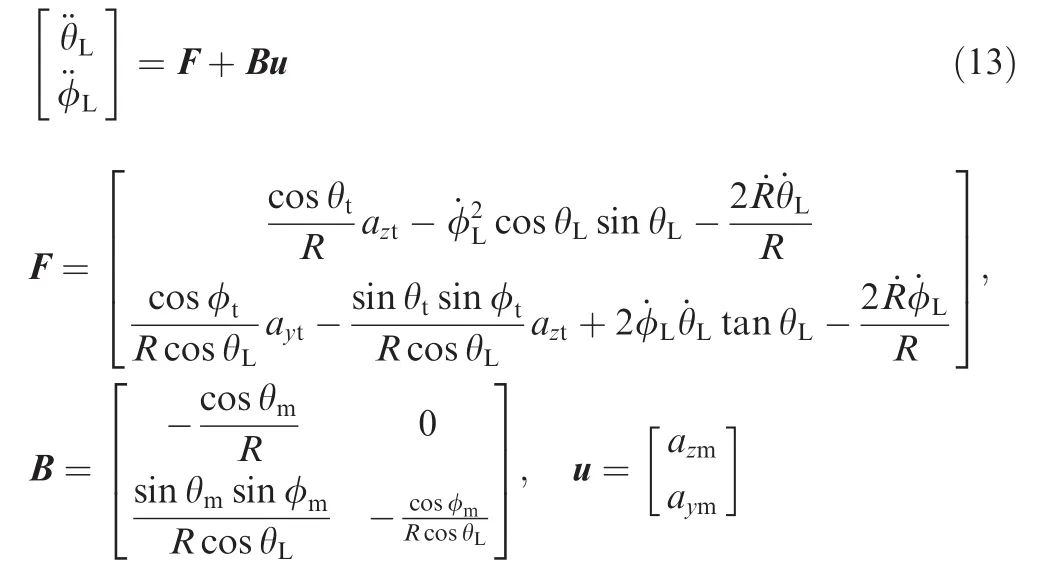
Note that because u is multiplied by matrix B,the LOS angles can be controlled only if R ≠ 0,and the angles θmand φmsatisfy θm,φm≠ ± (π/2).Because the missile intercepting the target by impact occurs when R=R0≠0 with Assumption 2,R≥R0during the terminal guidance phase.Hence,acceleration u can be used to control under the assumptions of θm≠ ± (π/2)and φm≠ ± (π/2).
3.Design of guidance laws
3.1.Design of a fast nonsingular guidance law



where r,η,β,and φ are positive constants that need to be determined,and 0<r<1.The derivative of S can be expressed as

where

The fast nonsingular terminal sliding mode guidance law(FNTSMGL)of a missile intercepting maneuvering targets with impact angle constraints can be expressed using the following equation with Assumptions 1 and 2:

Theorem 1.Considering the systems in Eqs.(1)–(7),if the external disturbance M is bounded,then‖M‖≤m.If Eq.(14)is selected as the sliding mode surface and Eq.(25)is selected as the guidance law,the following conclusions can be satisfied:
(i)The sliding manifold S converges to zero in finite time.
(ii)The LOS tracking error angles θL- θLfand φL- φLfconverge to the regions |θL-θLf|< η and|φL- φLf|< η in finite time,where η is a small positive constant.

Proof.Consider the Lyapunov function candidate as

The time derivative of V1along with Eqs.(1)–(7)results in

According to Lemma 1,inequality Eq.(28)indicates that the finite-time convergence of the sliding mode surface is available.Thus,conclusion(i)is proven.
Case I.If|xi|≥ η(i=1,2),consider another Lyapunov function candidate as

When S=0,the derivation of V2can be written as

Case II.If|xi|< η(i=1,2)and because

we can obtain

Remark 1.By adding the proportional term x to the sliding mode surface in Eq.(14),the guidance laws designed in this study can signi ficantly accelerate the convergence rate towards the manifold compared to the traditional terminal sliding mode guidance laws according to Ref.30.
Remark 2.From Theorem 1,the guidance law in Eq.(25)ensures that the LOS angular ratesandconverge to a small region,which is de fined using Eq.(26).From Eq.(26),we can conclude that η1is related to φ, η, β,and r.When η =0.05 and φ =0.1,the curves of η1are illustrated in Fig.2.Fig.2 shows that the value of η1decreases with a decrease in β and decreases with an increase in r.Therefore,a reasonable convergence region of the LOS angular rates can be obtained by adjusting the parameters.
Now,we have proposed a 3D finite-time convergent guidance law.In Theorem 1,it is assumed that‖M‖≤ m.However,the upper bound of M including ayt,azt, θt,and φtcannot be measured or estimated accurately.In addition,the exponential reaching law is used in the guidance law of Eq.(25).The exponential reaching law has the advantage of a fast convergence rate,but has the disadvantage in suppressing chattering.To solve these problems,new reaching laws will be introduced in Section 3.2.
3.2.Design of adaptive fast nonsingular guidance laws
In the sliding mode method,the control performance can be improved by selecting an appropriate reaching law that can accelerate the convergence rate and weaken the chattering phenomenon.Among the traditional reaching laws,the constant reaching law not only suffers from a slow convergence speed,but also causes a large chattering phenomenon to the systems.31The exponential reaching law significantly accelerates the convergence rate because of the presence of a constant term.32The power reaching law can weaken the chattering phenomenon to a certain extent.33Specific forms are shown below.
Gao34proposed the concept of reaching law and designed the power reaching law as

where h and α are positive coefficients.
The exponential reaching law was proposed in Ref.35,and the specific function is


Fig.2 Curves of η1and r with different values of β.
where k are positive coefficients.This type of reaching law was adopted in u1.It has the merit of a fast convergence rate,but it has the shortcoming of suppressing chattering.
To overcome the drawbacks of the exponential reaching law and simultaneously maintain its advantages,we introduce a new reaching law by combining an integral adaptation term with an exponential term as

where k>0,α>0,γ0>0,γ1>0,1>γ2>0,and p>0.-(αy+N(s))sign(s)can suppress chattering,and-ks can accelerate convergence.
By selecting the novel reaching law in Eq.(32)in this section,we can obtain

where γ0and γ1are positive constants;1 > γ2> 0 and α>1.

Then,a new finite-time adaptive guidance law for a missile intercepting maneuvering targets with impact angle constraints can be de fined as:

Theorem 2.Considering the systems in Eqs.(1)–(7),suppose that the external disturbance M is bounded.When Eq.(14)is selected as the sliding mode surface and Eq.(34)as the guidance law,the following conclusions are satis fied.
(i)The sliding manifold S converges to zero in finite time.
(ii)The LOS tracking error angles θL- θLfand φL- φLfconverge to the regions |θL-θLf|< η and|φL- φLf|< η in finite time,where η is a small positive constant.


The Lyapunov function candidate can be expressed as
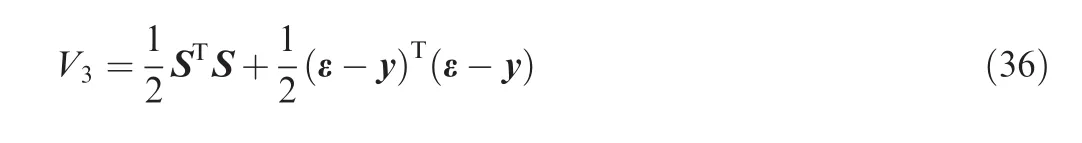
The time derivative of V3along with Eqs.(1)–(7)results in



where 0<μ<1.The time derivative of V4along with Eqs.(1)–(7)results in

where k,k1, α, γ0, γ00,and γ1are positive constants,1 ≥ γ2> 0,and α> 1.
Remark 3.The integration term y in the guidance law of Eq.(34)can compensate a perturbation with unknown bounds and force the sliding variable to converge to the sliding surface in finite time.The exponential term N(S)is a sufficiently high gain.When the state is far away from the sliding surface,the time of the compensating phase can be very short,i.e.,this term can accelerate the system’s response to perturbations.When S→0,the integration term gradually slows down until it stops growing.Moreover,the value of the exponential term decreases rapidly until it disappears at the sliding surface.On reaching the sliding surface,the overall gain can be reduced.In other word,this method can reduce the unwanted chattering level.As a result,when γ2is closer to 1,the reaching law has a better performance.Notably,γ2cannot be equal to 1;otherwise,the inequality Eq.(38)may not satisfy Lemma 1.
We have designed a guidance law that can deal with external disturbances.However,there is still a problem:the guidance laws in Eqs.(25)and(34)cannot guarantee that the states of the systems converge to zero.To deal with this problem,based on Theorem 2 and inspired by Refs.36,37,another new reaching law is introduced by adding-k1ϖsign(s)to the reaching law in Eq.(32)as

where k>0,k1>0,α>0,γ0>0,γ1>0,1≥γ2>0,and p > 0.-(αy+N(s))sign(s)can suppress chattering,and-ks can accelerate convergence.Moreover,-k1ϖsign(s)ensures that the systems are asymptotically and finite-time stable.
By selecting the novel reaching law in Eq.(39),we can obtain

Then,a new finite-time adaptive guidance law for a missile intercepting maneuvering targets with impact angle constraints can be de fined as

Theorem 3.Considering the systems in Eqs.(1)–(7),suppose that the external disturbance M is bounded.Both the LOS angular rate and LOS angle error can converge to zero while being asymptotically and finite-time stable,if Eq.(14)is selected as the sliding mode surface and Eq.(41)as the guidance law.
Proof.Consider the Lyapunov function candidate as

The time derivative of V5along with Eqs.(1)–(7)results in



where 0<μ<1.The time derivative of V4along with Eqs.(1)–(7)results in


Then

Because V6(t)≥0 and V6(t)is a monotone decreasing function,we have

where

Because V6(t)≡0,the sliding modes S and x converge to 0 when t≥t*.Then,according to Eq.(14),converges to 0 in finite time.In addition,ensures that the system is asymptotically and finite-time stable.Therefore,the conclusions of Theorem 3 can be easily obtained.□
Remark 4.In Theorem 3,the guidance law u3ensures that the LOS angular rate and LOS angle error converge to zero while being asymptotically and finite-time stable.Compared to u2,γ2can be equal to 1 by adopting the newly designed reaching law in Eq.(39).Moreover,the time of system states arriving at the equilibrium point can be accurately calculated using Theorem 3,but can only be estimated using Theorems 1 and 2.

Remark 6.Note that the guidance laws proposed in this paper are discontinuous because of the presence of a signum function,leading to the undesired chattering phenomenon.To weaken this phenomenon,a continuous saturation function sat(s)is given as Eq.(48).This function is used to approximate the signum function,i.e.,Eq.(48)can be used to replace the signum function.

4.Simulation results
In this part,numerical simulations are carried out to verify the performance of the designed guidance laws.
To illustrate the effectiveness of the designed guidance laws,the two cases shown below were selected for different target accelerations.
Case 1.ayt=azt=19.6cos(2t)m/s2.
Case 2.azt=19.6 m/s2and ayt=19.6 m/s2.
To analyze the superiority of the proposed guidance laws,the PNGL and NTSMGL22were compared.The PNGL can be expressed as


Table 1 Initial conditions for missile and target.
The nonsingular terminal sliding mode surface is given as

where β>0 is a constant.p>0 and q>0 are odd constants,following the condition of

The corresponding NTSMGL is given as

Two sets of initial engagement parameters are considered,and the details are given in Table 1.
The capacity of dynamic actuators is limited in practice;therefore,the maximum lateral accelerations are assumed to be limited as

where aMmax=25g,i.e.,the maximum allowable value of the missile acceleration is assumed to be 25g,and g is the acceleration of gravity(g=9.8 m/s2).

4.1.Simulation results under u1

For Case 1,When ayt=azt=19.6cos(2t)m/s2and the initial engagement parameters are considered as Dataset 1 in Table 1,the simulation results under u1are shown in Fig.3.Fig.3(a)shows the relative movement trajectories under three different guidance laws,and the missile can intercept the target successfully for Case 1,even though the flight paths of the missile are different.Fig.3(b)clearly shows that both u1and u0ensure the convergences of θLand φLto the desired values.However,the PNGL cannot guarantee the convergence of the LOS angles.In addition,clearly the LOS angles under u1have a faster convergence speed and better performance than those under u0.Fig.3(c)shows the curves of˙θLand˙φL.Similarly,still a good convergence performance was observed under u1.As shown in Fig.3(d),under the three different guidance laws,accelerations of the missile are in reasonable scales.For u1and u0,an acceleration saturation phenomenon exists,which lasts for about 4 s.Further,clearly the accelerations of the missile produced under the PNGL are smaller than those under u1or u0.However,a large acceleration of the missile under u1and u0ensures that the LOS angles and angular rates have preferable convergence performances.

Fig.3 Responses under u1with Case 1.
For Case 2,Fig.4 shows the simulation results under u1with azt=ayt=19.6 m/s2,and the initial engagement parameters are considered as Dataset 2 in Table 1.Fig.4(a)clearly shows that the target is maneuvering at a new way,and the missile can still intercept it successfully under three different guidance laws.Fig.4(b)shows the curves of θLand φL.The LOS angles θLand φLconverge to the desired values under both u1and u0.Fig.4(c)shows the curves of˙θLand˙φL.Figs.4(b)and(c)clearly show that the dynamic systems have the best convergence performances under u1.Fig.4(d)shows the missile acceleration pro files.The missile acceleration curves shown in Fig.4(d)converge to a constant within 5 s.
In conclusion,the control strategy u1also performs very well when the target is maneuvering in different ways.Furthermore,in Ref.22,the designed guidance law was simulated and verified only for nonmaneuvering targets.In this paper,simulation experiments were carried out for targets with different accelerations.The simulation results show that the performance of guidance laws obtained in this study is obviously better than that of the guidance law designed in Ref.22.This also validates that the guidance laws designed in this study have a faster convergence speed and stronger robustness than the PNGL and the guidance law reported in Ref.22.
To further verify the effectiveness of the guidance laws,simulation results for different desired impact angles,but with the same initial LOS angles,are presented.The initial conditions are given as Dataset 1 in Table 1.Let the desired impact angles θLfbe 5°,15°,25°,35°,45°,and 60°.Let the desired impact angles φLfbe-25°,-15°,-5°,5°,15°,and 30°.Fig.5 shows the trajectories of the missile and the target and the curves of the LOS angle θL.Fig.5(a)shows that the missile can intercept the target successfully for different desired impact angles.Fig.5(b)shows that when φLf=5°and θLf=5°,15°,25°,35°,45°,and 60°,θLcan converge to different desired angles precisely.However,the convergence time of θLis significantly affected by different desired impact angles.Using the same parameters,the greater the difference between the desired and initial angles is,the slower the convergence rate of θLis.That is because the value of the adaptive term increases with time.Therefore,it requires much more time to compensate the large errors between the initial and desired values.As shown in Fig.5(b),the optimal desired LOS angles of the proposed guidance law u1approach θLf=[5°,60°].Fig.6 shows the responses under u1with Dataset 1 when θLf=35°and φLf=-25°,-15°,-5°,5°,15°,and 30°. φLalso converges to different desired angles precisely,even though the convergence time is different.In addition,the optimal desired LOS angles of the proposed guidance law u1approach φLf=[-25°,30°].

Fig.4 Responses under u1with Case 2.

Fig.5 Responses under u1with Dataset 1 when φL=5° and θL=5°,15°,25°,35°,45°,60°.

Fig.6 Responses under u1with Dataset 1 when θL=35° and φL=-25°,-15°,-5°,5°,15°,30°.
4.2.Simulation results under guidance law u2
Similar to Section 4.1,the PNGL in Eq.(50)and the guidance law u0are still selected for comparison.
To validate u2,the selected parameters are η=0.025,r=0.2, β=0.7,k=1, α=2, γ0=4.849, γ00=5.259,γ1=0.1,γ2=0.99,and p=0.1.The parameter in the saturation function is selected as h=0.04.
For Case 1,Fig.7 shows the simulation results under the guidance law u2with ayt=azt=19.6cos(2t)m/s2,and the initial engagement parameters are considered as Dataset 1 in Table 1.Fig.7(a)shows the relative movement trajectories under u2,u0,and the PNGL.Clearly,the missile can intercept the target successfully under u2.Fig.7(b)–(c)show the curves of the LOS angles and angular rates.Both the guidance laws u0or u2ensure the convergence of the LOS angles to the expected values,and the LOS angular rates converge to zero.Under u2,u0,and the PNGL,Fig.7(d)shows the curves of the missile accelerations,which are within reasonable bounds.Fig.7(e)shows the adaptive value curves.Clearly,the dynamic systems under u2have a faster convergence speed and better performance than those under u0or the PNGL.
For Case 2,Fig.8 shows the simulation results under the second guidance law u2with azt=ayt=19.6 m/s2,and the initial engagement parameters are considered as Dataset 2 in Table 1.Fig.8(a)shows the relative movement trajectories under three different guidance laws.Fig.8(b)–(c)show the curves of θL,φL,˙θL,and˙φL.Fig.8(d)shows the missile acceleration pro files.Fig.8(e)shows the adaptive value curves.The comparison results are the same as those described in the previous section.Therefore,the remaining analyses are omitted here.
According to the above analyses,u2has a better performance than the PNGL and the NTSMGL.
Fig.9 shows the responses under u2with Dataset 1 when φLf=5°and θLf=5°,15°,25°,35°,45°,and 60°.Fig.10 shows the responses under u2with Dataset 1 when θLf=35°and φLf=-25°,-15°,-5°,5°,15°,and 30°.The situations are similar to those shown in Figs.5 and 6,thus not repeated here.
To further prove the superiority of the chattering elimination of u2,the sliding mode guidance law u1is selected for comparison.Notably,the signum function is no longer replaced by a saturation function in this part.
Fig.11(a)and(b)show the comparison results of u1and u2including the curves of sliding mode surfaces.The sliding mode surface pro files shows a severe chattering phenomenon under u1,i.e.,the undesired chattering is reduced effectively by the guidance law u2.The comparison results between u1and u2show that on one hand,the integration term y in u2can compensate a perturbation with unknown bounds and force the sliding variable to converge to the sliding surface in finite time.On the other hand,the magnitude of y can be adjusted according to the value of the sliding mode surface,reducing the unwanted chattering level.This is sufficient to validate the good performance and superiority of u2.
4.3.Simulation results under guidance law u3
Similar to Sections 4.1 and 4.2,the PNGL and u0are selected for comparison to verify the effectiveness of u3.


Fig.7 Responses under u2with Case 1.

Fig.8 Responses under u2with Case 2.

Fig.9 Responses under u2with Dataset 1 when φLf=5° and θLf=5°,15°,25°,35°,45°,60°.

Fig.10 Responses under u2with Dataset 1 when θLf=35° and φLf=-25°,-15°,-5°,5°,15°,30°.

Fig.11 Comparison between u1and u2with azt=ayt=20g.
For Case 1,Fig.12 shows the simulation results under u3for Case 1,and the initial engagement parameters are considered as Dataset 1 in Table 1.Fig.12(a)shows the relative movement trajectories under u3,u0,and the PNGL,and the missile can hit the target successfully under the three different guidance laws.The relative movement curves under u3are similar to those under u0,but are quite different from those under the PNGL.Fig.12(b)and(c)show the curves of the LOS angles and angular rates.Clearly,the guidance systems have a faster convergence speed under u3than those under u0and the PNGL.Fig.12(d)shows the curves of the missile accelerations under the three different guidance laws,which are within reasonable bounds.Fig.12(e)shows the adaptive value curves.
From the above analysis,clearly the dynamic systems under u3have a faster convergence speed and better performance than those under u0or the PNGL.

Fig.12 Responses under u3with Case 1.

Fig.13 Responses under u3with Case 2.

Fig.14 Comparison between u1and u3with azt=ayt=20g.

Fig.15 Effect of gain on the performance of the guidance law.
For Case 2,Fig.13 shows the simulation results under u3with azt=ayt=19.6 m/s2,and the initial engagement parameters are considered as Dataset 2 in Table 1.Fig.13(a)shows the relative movement trajectories under three different guidance laws.Figs.13(b)and 13(c)show the curves of θL, φL,˙θL,and˙φL.Fig.13(d)shows the missile acceleration pro files.Fig.13(e)shows the adaptive value curves.The comparison results are the same as described in the previous sections.Therefore,the remaining analyses are omitted here.
According to the above analysis,the proposed adaptive guidance law u3has a better performance than those of the PNGL and the NTSMGL.
The simulation results of different desired impact angles under u3are similar to those shown in Figs.5 and 6,thus not repeated here.
Similar to Section 4.2,u1is still selected to compare with u3.Notably,the signum function is no longer replaced by the saturation function in this part.Fig.14(a)and(b)show the comparison results between u1and u3.The sliding mode surface pro files show a severe chattering phenomenon under u1.In u3,weakening of undesired chattering was caused by adding-Q(αy+N(S))to the reaching law.This is sufficient to demonstrate the good performance and superiority of u3.
In addition,the effect of k1on the value of the guidance law is analyzed by simulation,and the results are shown in Fig.15.Fig.15(a)shows that k1is too large and would cause an overshoot phenomenon.Fig.15(b)shows that the duration of the saturation phenomenon would increase with an increase in the numerical value of k1.Therefore,a route to obtain a better convergence performance is by adjusting k1.
Table 2 shows the precise data including interception time,miss distance,and LOS angle error.Although the guidance laws are different,the interception time is similar.Further,the comparison between different guidance laws under the two cases shows that the miss distances and LOS angle errors derived under u1,u2,and u3are much less than those derived under u0and the PNGL.Therefore,u1,u2,and u3ensure the guidance accuracy and success rate of the interception.Moreover,a high guidance precision can be achieved even in the case of an existing strong target maneuver.

Table 2 Interception time,miss distance,and LOS angle error.
5.Conclusions
For a missile intercepting maneuvering targets in a 3D engagement scene,this study has proposed three novel fastconvergence guidance laws with terminal impact angle constraints.To intercept maneuvering targets,all the three guidance laws designed in this study ensure that the LOS angular rate and LOS angle are finite-time stabilized.The details can be summarized as follows:
(1)The first guidance law is developed based on the fast nonsingular terminal sliding mode control theory.This guidance law can compensate target acceleration with a known upper bound.
(2)Based on the newly designed reaching law,an adaptive sliding mode guidance law is provided.Besides,this guidance law can deal with the problem of target acceleration with unknown bounds.
(3)Based on another newly designed reaching law,the third guidance law is developed.This guidance law can ensure that the LOS angular rate and LOS angle are asymptotically and finite-time stable.
(4)The theoretical proofs and simulation results have verified that the three guidance laws designed in this study are effective and superior.
Acknowledgements
This study was co-supported by the National Natural Science Foundation of China(No.61333003)and the China Aerospace Science and Technology Innovation Foundation(No.JZ20160008).
1.Lee CH,Kim TH,Tahk MJ.Design of impact angle control guidance laws via high-performance sliding mode control.Proc IMechE Part G:J Aerospace Eng 2013;227(2):235–53.
2.Kim BS,Lee JG,Han HS.Biased PNG law for impact with angular constraint.IEEE Trans Aerosp Electron Syst 1998;34(1):277–88.
3.Erer KS,Merttopc¸uoglu O.Indirect impact-angle-control against stationary targets using biased pure proportional navigation.J Guid Control Dynam 2012;35(2):700–4.
4.Oza HB,Padhi R.Impact-angle-constrained suboptimal model predictive static programming guidance of air-to-ground missiles.J Guid Control Dynam 2012;35(1):153–64.
5.Lee YI,Kim SH,Tahk MJ.Optimality of linear time-varying guidance for impact angle control.IEEE Trans Aerosp Electron Syst 2012;48(4):2802–17.
6.Lee YI,Kim SH,Lee JI.Analytic solutions of generalized impactangle-control guidance law for first-order lag system.J Guid Control Dynam 2012;36(1):96–112.
7.Lee CH,Kim TH,Tahk MJ.Interception angle control guidance using proportional navigation with error feedback.J Guid Control Dynam 2013;36(5):1556–61.
8.Utkin VI,Chang HC.Sliding mode control on electro-mechanical systems.Math Probl Eng 2002;8(4–5):451–73.
9.Hou M,Duan G.Integrated guidance and control of homing missiles against ground fixed targets.Chin J Aeronaut 2008;21(2):162–8.
10.Zhang Y,Sun M,Chen Z.Finite-time convergent guidance law with impact angle constraint based on sliding-mode control.Nonlinear Dynam 2012;70(1):619–25.
11.Xiong S,Wang W,Liu X.Guidance law against maneuvering targets with intercept angle constraint.ISA Trans 2014;53(4):1332–42.
12.Kumar SR,Rao S,Ghose D.Non-singular terminal sliding mode guidance and control with terminal angle constraints for nonmaneuvering targets.In:Proceedings of the 12th ieee workshop on variable structure systems;2012 Jan 12–14;Mumbai,India.New York:IEEE;2012.p.291–6.
13.Song QZ,Meng XY.Design and simulation of guidance law with angular constraint based on non-singular terminal sliding mode.In:Proceedings of the 2012 international conference on solid state devices and materials science;2012 Apr 1–2;Macao,China.London:Elsevier;2012.p.1197–204.
14.Kumar SR,Rao S,Ghose D.Nonsingular terminal sliding mode guidance with impact angle constraints.J Guid Control Dynam 2014;37(4):1114–30.
15.Zhao J,Zhou J.Strictly convergent nonsingular terminal sliding mode guidance law with impact angle constraints.Optik-Int J Light Electron Opt 2016;127(22):10971–80.
16.Liu JH,Shan JY,Liu Q.Optimal pulsed guidance law with terminal impact angle constraint.Proc IMechE Part G:J Aerospace Eng(in press).DOI:10.1177/0954410016664918.
17.Sun S,Zhou D,Hou W.A guidance law with finite time convergence accounting for autopilot lag.Aerosp Sci Technol 2013;25(1):132–7.
18.Cho N,Kim Y,Park S.Three-dimensional nonlinear differential geometric path-following guidance law.J Guid Control Dynam 2015;38(12):2366–85.
19.Song J,Song S.Three-dimensional guidance law based on adaptive integral sliding mode control.Chin J Aeronaut 2016;29(1):202–14.
20.He S,Wang W,Wang J.Three-dimensional impact angle guidance laws based on model predictive control and sliding mode disturbance observer.J Dyn Syst Meas Contr 2016;138(8):1–11.
21.Wang W,Xiong S,Wang S,Song S,Lai C.Three dimensional impact angle constrained integrated guidance and control for missiles with input saturation and actuator failure.Aerosp Sci Technol 2016;53:169–87.
22.Kumar SR,Ghose D.Three-dimensional impact angle guidance with coupled engagement dynamics.Proc IMechE Part G:J Aerospace Eng 2017;231(4):621–41.
23.Li G,Ji H.A three-dimensional robust nonlinear terminal guidance law with ISS finite-time convergence.Int J Control 2016;89(5):938–49.
24.Li G,Ji H.A three-dimensional terminal guidance law based on finite-time input-to-state stability.In:Proceedings of the 34th Chinese control conference;2015 July 28–30;Hangzhou.Piscataway(NJ):IEEE;2015.p.5174–81.
25.Song SH,Ha IJ.A Lyapunov-like approach to performance analysis of 3-dimensional pure PNG laws.IEEE Trans Aerosp Electron Syst 1994;30(1):238–48.
26.Yu SH,Yu XH,Man ZH.Continuous finite-time control for robotic manipulators with terminal sliding mode.Automatica 2005;41(11):1957–64.
27.Pukdeboon C,Siricharuanun P.Nonsingular terminal sliding mode based finite-time control for spacecraft attitude tracking.Int J Control Autom 2014;12(3):530–40.
28.Hardy H,Littlewood JE,Polya G.Inequalities.2nd ed.Cambridge:Cambridge University Press;1952.p.31–2.
29.Shtessel YB,Shkolnikov IA,Levant A.Guidance and control of missile interceptor using second-order sliding modes.IEEE Trans Aerosp Electron Syst 2009;45(1):110–24.
30.Yang L,Yang J.Fast nonsingular terminal sliding-mode control for nonlinear dynamical systems.Int J Robust Nonlin 2011;21(16):1865–79.
31.Mehta A,Bandyopadhyay B.In frequency shaped and observerbased discrete-time sliding mode control.Springer Briefs Appl Sci Technol 2015;37(5):9–25.
32.Xie WF.Sliding-mode-observer-based adaptive control for servo actuator with friction.IEEE Trans Ind Electron2007;54(3):1517–27.
33.Niu Y,Hu DW,Wang Z.Improved sliding mode control for discrete-time systems via reaching law.IET Control Theory A 2010;4(11):2245–51.
34.Gao WB.Theory and design method of variable structure control.2nd ed.Beijing:Science Press;1996.p.27–8.
35.Fallaha CJ.Sliding-mode robot control with exponential reaching law.IEEE Trans Ind Electron 2011;58(2):600–10.
36.Huo M,Huo X,Karimi HR.Finite-time control for attitude tracking maneuver of rigid satellite.Abstr Appl Anal 2014;2014(2014):1–15.
37.Jin XZ,He YG,Wang D.Adaptive finite-time synchronization of a class of pinned and adjustable complex networks.Nonlinear Dynam 2016;85(3):1–11.
3 November 2016;revised 7 December 2016;accepted 3 March 2017
Available online 7 June 2017
Ⓒ2017 Chinese Society of Aeronautics and Astronautics.Production and hosting by Elsevier Ltd.This is an open access article under the CCBY-NC-ND license(http://creativecommons.org/licenses/by-nc-nd/4.0/).
*Corresponding author.Tel.:+86 451 8642224 8214.
E-mail address:songshenmin@hit.edu.cn(S.SONG).
Peer review under responsibility of Editorial Committee of CJA.
 CHINESE JOURNAL OF AERONAUTICS2017年6期
CHINESE JOURNAL OF AERONAUTICS2017年6期
- CHINESE JOURNAL OF AERONAUTICS的其它文章
- A general method for closed-loop inverse simulation of helicopter maneuver flight
- Numerical simulation of a cabin ventilation subsystem in a space station oriented real-time system
- Parametric analyses on dynamic stall control of rotor airfoil via synthetic jet
- Effect of particle size and oxygen content on ignition and combustion of aluminum particles
- Effects of axial gap and nozzle distribution on aerodynamic forces of a supersonic partial-admission turbine
- Effect of a transverse plasma jet on a shock wave induced by a ramp
