A NOTE ON MEASURE-THEORETICEQUICONTINUITY AND RIGIDITY*

Chiyi LUO(罗炽逸)Yun ZHAO (赵云)*

School of Mathematical Sciences and Center for Dynamical Systems and Differential Equations Soochow University，Suzhou 215006，China E-mail: 20194207005@stu.suda.edu.cn; zhaoyun@suda.edu.cn

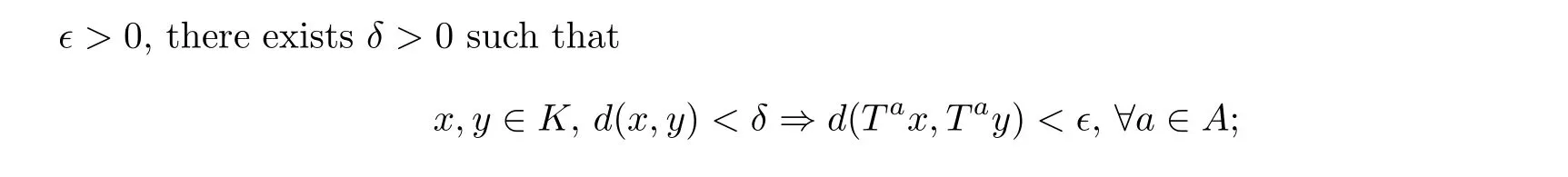
(ii) a function f ∈L2(µ) is called µ-A-equicontinuous if, for any τ > 0, there exists a compact subset K ⊂X with µ(K)>1-τ such that {f ◦Ta:a ∈A} is equicontinuous on K,that is, for any ∊>0, there exists δ >0 such that

Given a TDS (X,T), a T-invariant measure µ and f ∈L2(µ), Cai [1] proved the following results which gave the relations between measure-theoretic equicontinuity and rigidity of a TDS(see[4,6-8]and references therein for more descriptions of the relations between the topological equicontinuity and rigidity of a TDS):

Theorem 1.2 ([1, Corollary 3.9]) Let (X,T) be a TDS and µ be a T-invariant measure.Then (X,B,µ,T) is rigid if and only if there exists a subsequence A = {ai: i ∈N} of N such that (X,T) is µ-A-equicontinuous.

Theorem 1.3 ([1, Corollary 3.11]) Let (X,T) be a TDS, µ be a T-invariant measure,and f ∈L2(µ). Then f is rigid if and only if there exists a subsequence A={ai: i ∈N} of N such that f is µ-A-equicontinuous.

The notion of µ-mean equicontinuity was introduced in [3], where it was proved that if an MPS (X,B,µ,T) is ergodic, then it has discrete spectrum if and only if it is µ-mean equicontinuous. Later, Huang et al. [5] introduced a similar notion called µ-equicontinuity in the mean and proved that these two notions are equivalent; moreover, they proved that a general MPS (X,B,µ,T) has discrete spectrum if and only if it is µ-mean equicontinuous (see [10] for another proof). Following this idea, Cai [1] introduced the notion of measure-theoretic mean equicontinuity along some subsequence A of N.

Definition 1.4 Given a TDS (X,T) and a subsequence A={ai:i ∈N}⊂N, let µ be a T-invariant measure. Then,

(i) (X,T)is calledµ-A-equicontinuous in the mean if,for each τ >0,there exists a compact subset K ⊂X with µ(K)>1-τ such that for any ∊>0, there exists δ >0 such that

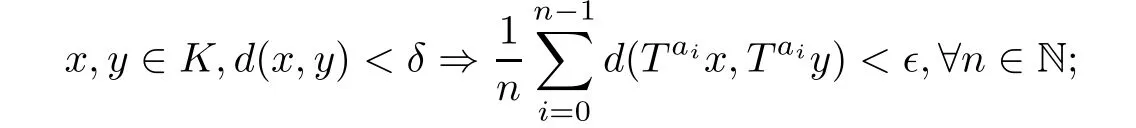
(ii) a function f ∈L2(µ) is calledµ-A-equicontinuous in the mean if, for each τ >0, there exists a compact subset K ⊂X with µ(K)>1-τ such that for any ∊>0, there exists δ >0 such that

In a similar fashion, we say that a TDS (X,T) is µ-A-mean equicontinuous if, for each τ >0, there exists a compact subset K ⊂X with µ(K)>1-τ such that

and that a function f ∈L2(µ) is µ-A-mean equicontinuous if, for each τ > 0, there exists a compact subset K ⊂X with µ(K)>1-τ such that

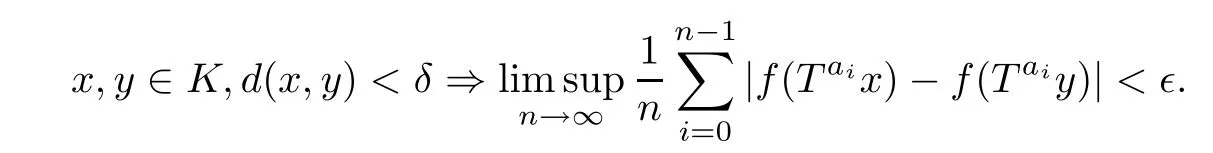
In fact,the notions ofµ-A-mean equicontinuous andµ-A-equicontinuous in the mean are equivalent with respect to a TDS or a function f;see Theorem 4.2 and Theorem 4.8 in[1]for detailed proofs.

In [1], Cai obtained the following relations between measure-theoretic mean equicontinuity and rigidity:

Theorem 1.5 ([1, Theorem 4.5]) Let (X,T) be a TDS and µ be a T-invariant measure.If there exists a subsequence A = {ai: i ∈N} of N with D(A) > 0 such that (X,T) isµ-A-equicontinuous in the mean, then (X,B,µ,T) is rigid.

Theorem 1.6 ([1, Theorem 4.9]) Let (X,T) be a TDS, µ be a T-invariant measure and f ∈L2(µ). If there exists a subsequence A = {ai: i ∈N} of N with D(A) > 0 such that f isµ-A-equicontinuous in the mean, then f is rigid.

In [1], Cai asked the question of whether or not the condition D(A) > 0 in the above two theorems is necessary. In fact, this is the main obstacle to giving the equivalence between measure-theoretic mean equicontinuity and rigidity. In this paper, we show that the condition D(A)>0 is redundant. Furthermore, we obtain the following results:

Theorem 1.7 Let (X,T) be a TDS and let µ be a T-invariant measure. Then the following statements are equivalent:

1. (X,B,µ,T) is rigid, where B is the σ-algebra on X;

2. there exists a subsequence A={ai:i ∈N} of N such that (X,T)is µ-A-equicontinuous in the mean.

Theorem 1.8 Let (X,T) be a TDS, let µ be a T-invariant measure and let f ∈L2(µ).Then the following statements are equivalent:

1. f is rigid;

2. there exists a subsequence A = {ai: i ∈N} of N such that f is µ-A-equicontinuous in the mean.

In the next section, we will give detailed proofs of these two theorems.

2 Proofs of the Main Theorems

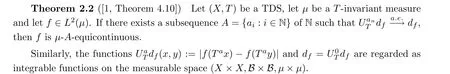
Proof of Theorem 1.7 If (X,B,µ,T) is rigid, by Theorem 1.2, there exists a subsequence A of N such that (X,T) is µ-A-equicontinuous, that is, for any τ > 0, there exists a compact subset K ⊂X with µ(K) > 1-τ such that {Ta: a ∈A} is equicontinuous on K,that is, for any ∊>0, there exists δ >0 such that

Therefore, the TDS (X,T) is µ-A-equicontinuous in the mean.

To prove the other direction, we assume, without loss of generality, that d(x,y)≤1 for all x,y ∈X. It suffices to prove that there exists a subsequence B ={bi:i ∈N} of N such that

Consequently, for every (x,y)∈Xi×Xj, we have that