Exact Solutions and Finite Time Stability of Linear Conformable Fractional Systems with Pure Delay
Ahmed M.Elshenhab,Xingtao Wang,Fatemah Mofarreh and Omar Bazighifan
1School of Mathematics,Harbin Institute of Technology,Harbin,150001,China
2Department of Mathematics,Faculty of Science,Mansoura University,Mansoura,35516,Egypt
3Mathematical Science Department,Faculty of Science,Princess Nourah Bint Abdulrahman University,Riyadh,11546,Saudi Arabia
4Section of Mathematics,International Telematic University Uninettuno,Roma,00186,Italy
ABSTRACT We study nonhomogeneous systems of linear conformable fractional differential equations with pure delay.By using new conformable delayed matrix functions and the method of variation,we obtain a representation of their solutions.As an application,we derive a finite time stability result using the representation of solutions and a norm estimation of the conformable delayed matrix functions.The obtained results are new,and they extend and improve some existing ones.Finally,an example is presented to illustrate the validity of our theoretical results.
KEYWORDS Representation of solutions;conformable fractional derivative;conformable delayed matrix function;conformable fractional delay differential equations;finite time stability
1 Introduction
In recent years,particularly in 2014,Khalil et al.[1]introduced a new definition of the fractional derivative called the conformable fractional derivative that extends the classical limit definition of the derivative of a function.The conformable fractional derivative has main advantages compared with other previous definitions.It can,for example,be used to solve the differential equations and systems exactly and numerically easily and efficiently,it satisfies the product rule and quotient rule,it has results similar to known theorems in classical calculus,and applications for conformable differential equations in a variety of fields have been extensively studied,see [2–10] and the references therein.On the other hand,in 2003,Khusainov et al.[11]represented the solutions of linear delay differential equations by constructing a new concept of a delayed exponential matrix function.In 2008,Khusainov et al.[12] adopted this approach to represent the solutions of an oscillating system with pure delay by establishing a delayed matrix sine and a delayed matrix cosine.This pioneering research yielded plenty of novel results on the representation of solutions,which are applied in the stability analysis and control problems of time-delay systems;see for example[13–28]and the references therein.Thereafter,in 2021,Xiao et al.[29]obtained the exact solutions of linear conformable fractional delay differential equations of orderα∈(0,1]by constructing a new conformable delayed exponential matrix function.
However,to the best of our knowledge,no study exists dealing with the representation and stability of solutions of conformable fractional delay differential systems of orderα∈(1,2].
Motivated by these papers,we consider the explicit formula of solutions of linear conformable fractional differential equations with pure delay
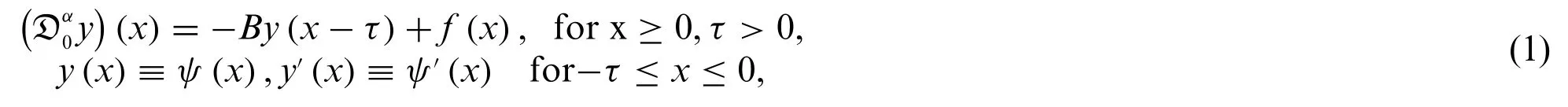
by constructing new conformable delayed matrix functions.Moreover,the representation of solutions of Eq.(1) is used to obtain a finite time stability result onW= [0,L],L >0,whereis called the conformable fractional derivative of orderα∈(1,2] with lower index zero,y(x)∈Rn,ψ∈C2([-τ,0],Rn),B∈Rn×nis a constant nonzero matrix andf∈C([0,∞),Rn)is a given function.
The paper is organized as follows: In Section 2,we present some basic definitions concerning conformable fractional derivative and finite time stability,and construct new conformable delayed matrix functions and derive their properties for use when we discuss the representation of solutions and finite time stability.In Section 3,by using the new conformable delayed matrix functions,we give the explicit formula of solutions of Eq.(1).In Section 4,as an application,we derive a finite time stability result using the representation of solutions.Finally,we give an example to illustrate the main results.
2 Preliminaries
Throughout the paper,we denote the vector norm and matrix norm,respectively,as‖y‖=and‖B‖ =yandbare the elements of the vectoryand the matrixB,respectively.iijDenoteC(W,Rn)the Banach space of vector-value continuous function fromW→Rnendowed with the norm ‖y‖C= maxx∈W‖y(x)‖ for a norm ‖·‖ on Rn.We introduce a spaceC1(W,Rn)={y∈C(W,Rn):y′∈C(W,Rn)}.Furthermore,we see‖ψ‖C=maxυ∈[-τ,0]‖ψ(υ)‖.
We recall some basic definitions of conformable fractional derivative,fractional exponential function,and finite time stability.
Definition 2.1.([2,Definition 2.2]).Letf:[a,∞)→Rnbe a differentiable function atx.Then the conformable fractional derivative forfof orderα=(1,2]is given by

if the limit exists.
Remark 2.1.As a consequence of Definition 2.1,we can show that
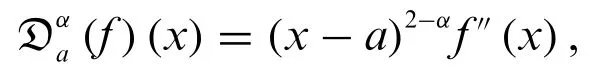
whereα=(1,2],andfis 2-differentiable atx >a.
Definition 2.2.([2]).We define the fractional exponential function as follows:
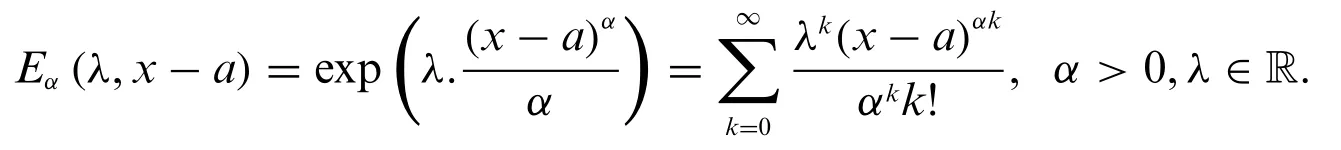
Definition 2.3.([30]).The system in Eq.(1)is finite time stable with respect to{0,W,τ,δ,β},δ <βif and only ifη <δimplies‖y(x)‖<βfor allx∈W,whereη=maxandδ,βare real positive numbers.
Next,we construct new conformable delayed matrix functions that are the fundamental solution matrices of Eq.(1).
Definition 2.4.The conformable delayed matrix functionsHτ,α(Bxα)andMτ,α(Bxα)are defined as
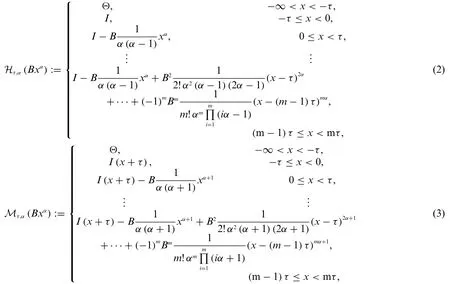
respectively,wherem=0,1,2,...,Iis then×nidentity matrix andΘis then×nnull matrix.
Lemma 2.1.The following rule is true:
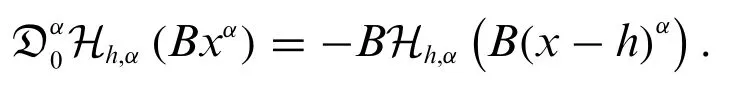
Proof.First,whenx∈(-∞,-τ),we obtainHτ,α(Bxα)=Hτ,α(B(x-τ)α)=Θ,and we can see that Lemma 2.1 holds.Following that,set(m-1)τ≤x <mτ,m=0,1,2,...,we get
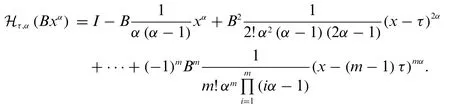
Applying Remark 2.1,we get

This completes the proof.
In the same way that we proved Lemma 2.1,we can derive the next result.
Lemma 2.2.The following rule is true:
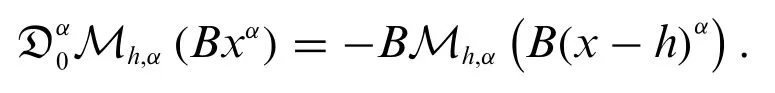
To conclude this section,we provide a norm estimation of the conformable delayed matrix functions,which is used while discussing finite time stability.
Lemma 2.3.For anyx∈[(m-1)τ,mτ],m=0,1,2,...,we have

Proof.Taking the norm of Eq.(2),we get
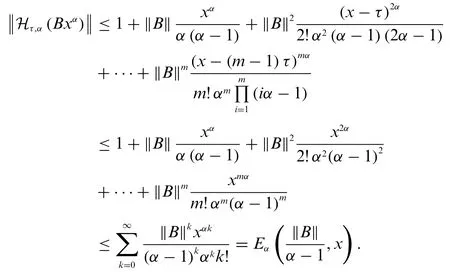
This completes the proof.
Lemma 2.4.For anyx∈[(m-1)τ,mτ],m=0,1,2,...,we have
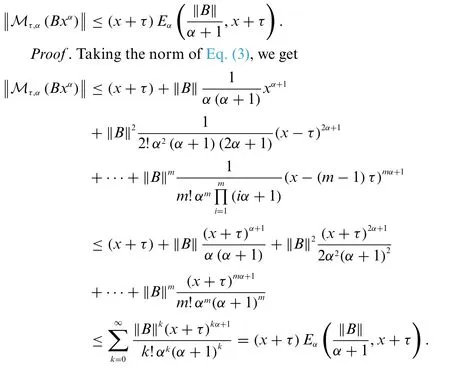
This completes the proof.
3 Exact Solutions for Linear Conformable Fractional Delay Systems
In this section,we give the exact solutions of Eq.(1)via the conformable delayed matrix functions and the method of variation of constants.To do this,we consider the homogeneous system of linear conformable fractional delay differential equations
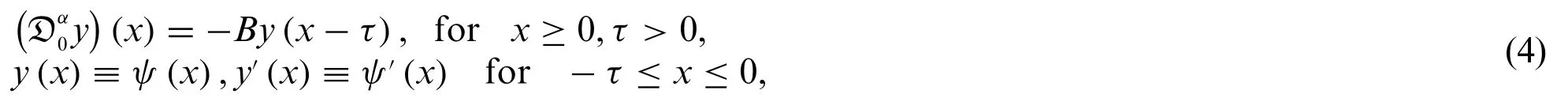
and the linear inhomogeneous conformable fractional delay system

Theorem 3.1.The solutiony(x)of Eq.(4)has the representation
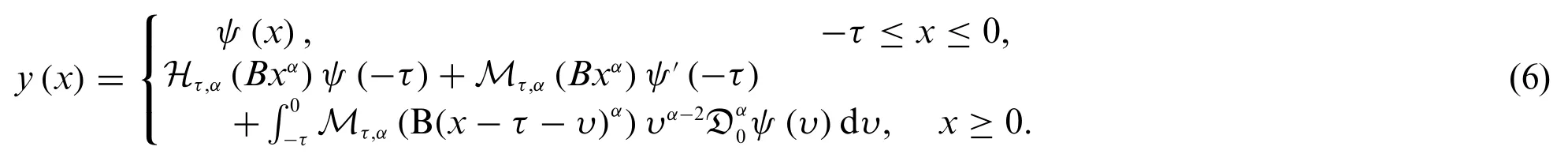
Proof.We seek for a solution of Eq.(4)in the form
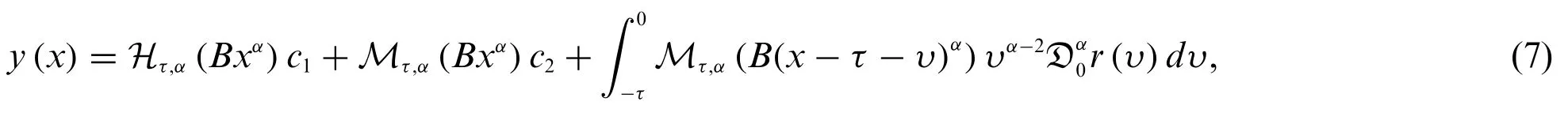
or
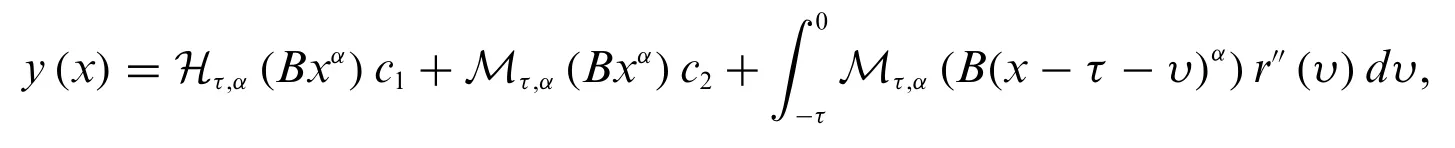
wherec1andc2are unknown constants vectors on Rn,andr(x)is an unkown twice continuously differentible vector function.From Lemmas 2.1 and 2.2,we deduce thatHτ,α(Bxα)andMτ,α(Bxα)are solutions of Eq.(4).We notice that Eq.(6)is a solution of Eq.(4)due to the linearity of solutions for arbitraryc1,c2andr(x).Now we find the constantsc1andc2,and the vector functionr(x)so that the initial conditionsy(x)≡ψ(x),y(x)≡ψ′(x)for -τ≤x≤0,are satisfied.That is,the following relations hold for-τ≤x≤0:
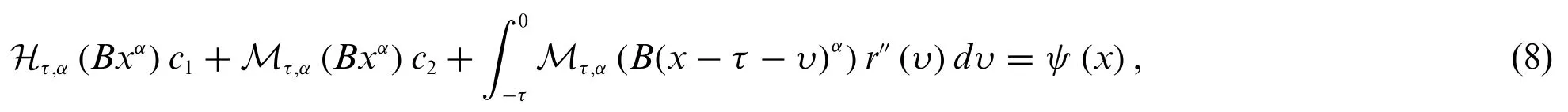
and

Consider Eq.(8).If-τ≤x <0,then
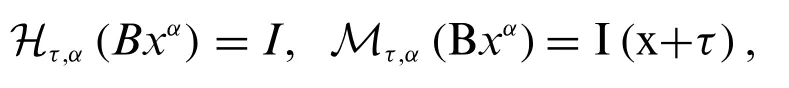
and
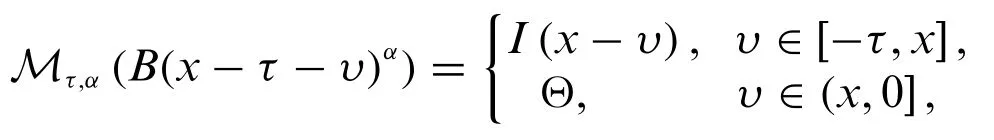
which implies that

and
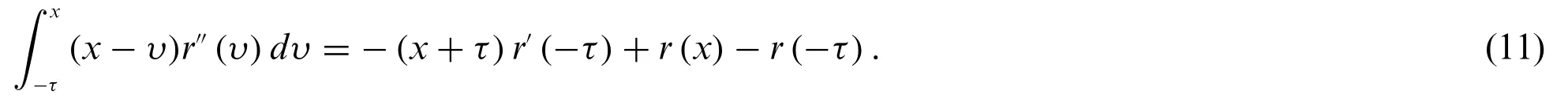
Substituting Eq.(11)into Eq.(10),we get
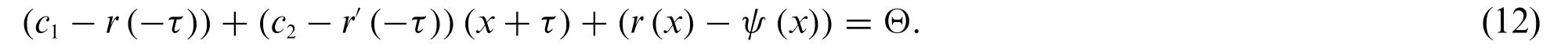
Differentiating Eq.(12)with respect tox,we have

As a result,we find that the equalities obtained Eqs.(12)and(13)are true if

Substituting Eq.(14)into Eq.(7),we obtain Eq.(6).This finishes the proof.
Theorem 3.2.The particular solutiony0(x)of Eq.(5)has the representation

Proof.We try to find a particular solutiony0(x)of Eq.(5)in the form

by applying the method of variation of constants,whereξ(υ),0<υ≤x,is an unknown function.Taking the conformable derivative of Eq.(16),we get

Substituting Eqs.(16)and(17)into Eq.(5),and noting that
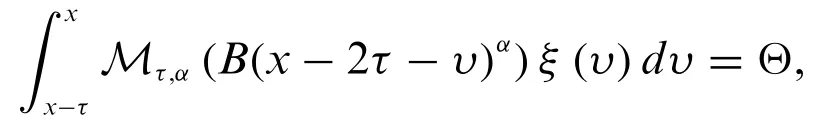
We havex2-αξ(x)=f (x).Substitutingξ(x)=xα-2f (x)into Eq.(16),we obtain Eq.(15).This completes the proof.
Corollary 3.1.The solutiony(x)of Eq.(1)can be represented as

Remark 3.1.Letα=2 in Eq.(1).Then Corollary 3.1 coincides with Corollary 1 in[13].
Remark 3.2.Letα= 2,B=B2in Eq.(1)such that the matrixBis a nonsingularn×nmatrix.Then

where cosτ(Bx)and sinτ(Bx)are called the delayed matrix of cosine and sine type,respectively,defined in[12].Therefore,Corollary 3.1 coincides with Theorems 1 and 2 in[12].
4 Finite Time Stability of Linear Conformable Fractional Delay Systems
In this section,we establish some sufficient conditions for the finite time stability results of Eq.(1)by using a norm estimation of the conformable delayed matrix functions and the formula of general solutions of Eq.(1).
Theorem 4.1.The system Eq.(1)is finite time stable with respect to{0,W,τ,δ,β},δ <βif
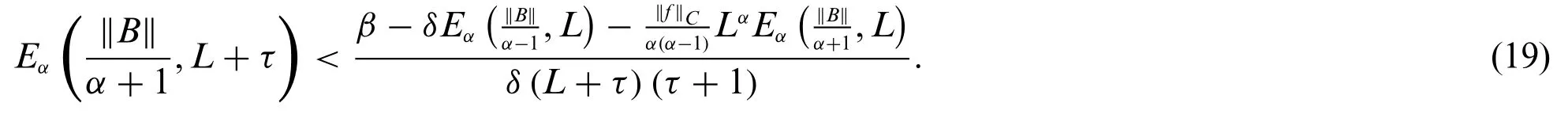
Proof.By using Definition 2.3,and Theorems 3.1 and 3.2,we haveη <δand
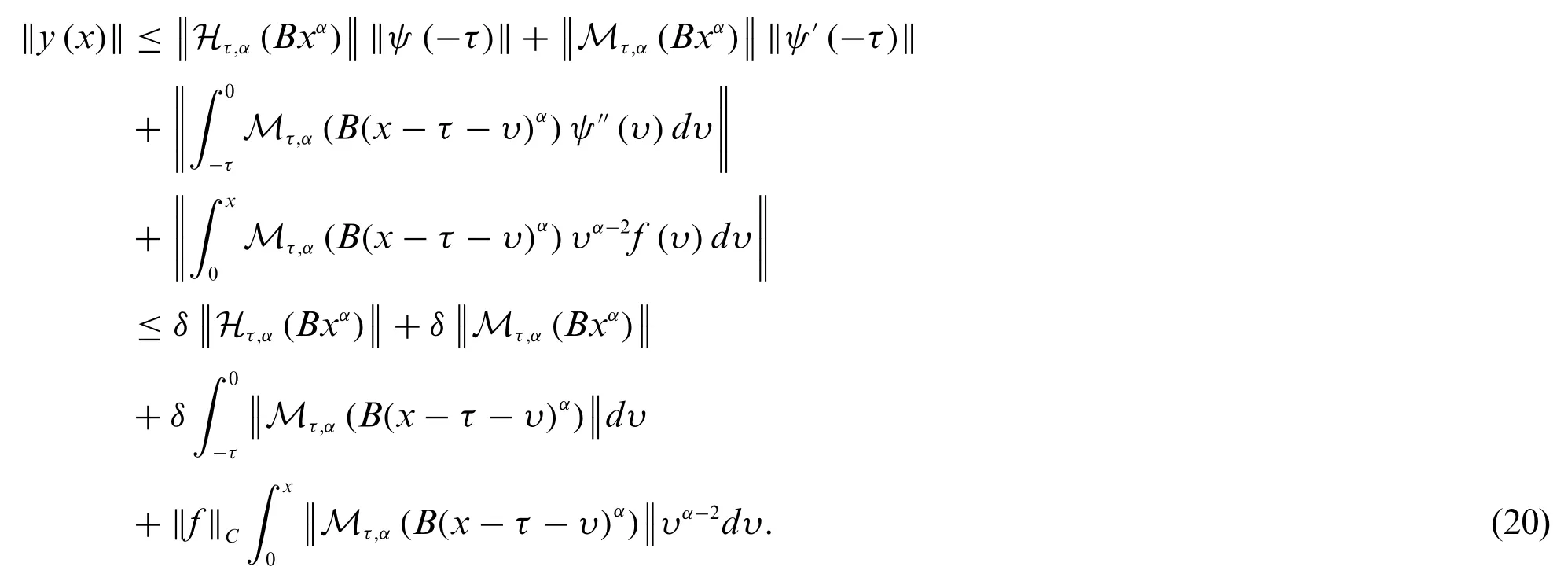
Note thatMτ,α(Bxα)=Θifx∈(-∞,-τ).For-τ≤υ≤0,we get
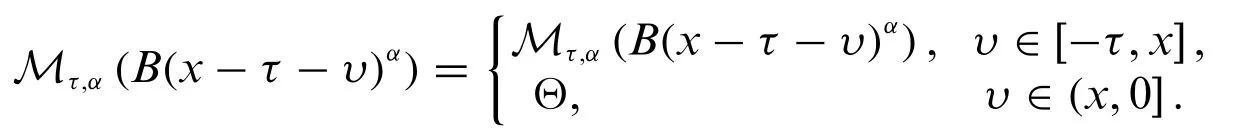
Thus
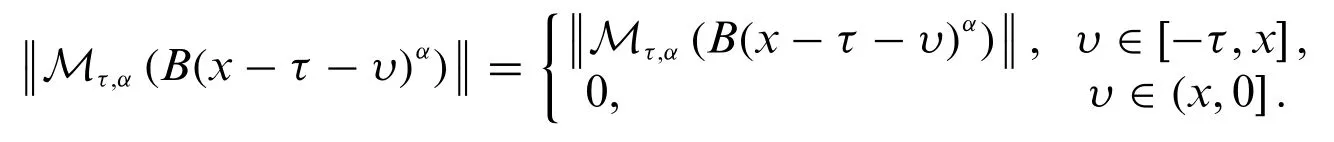
Therefore,from Lemma 2.4,we have
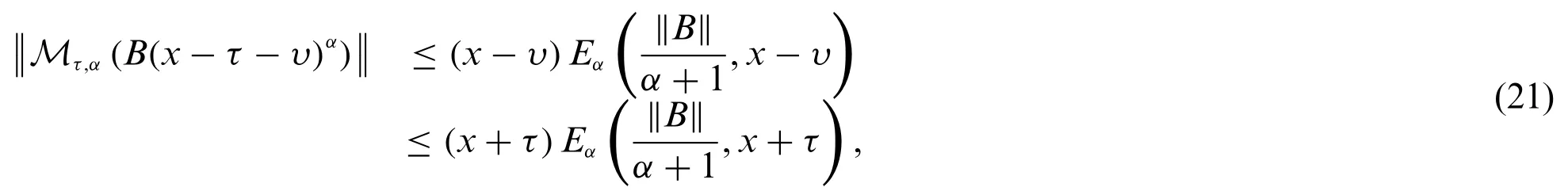
for-τ≤υ≤0,x∈W,and sinceEαis increasing function whenx≥υ.From Eq.(21),we get
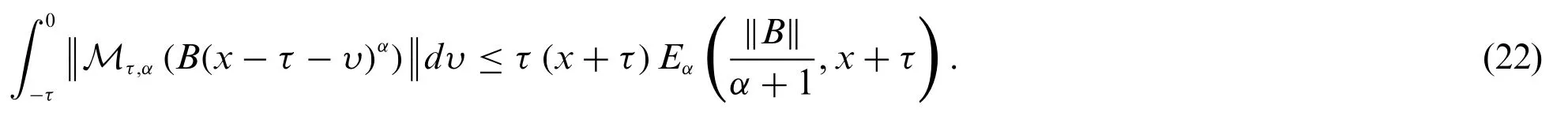
From Lemma 2.4,we have

From Eqs.(20),(22)and(23),we get
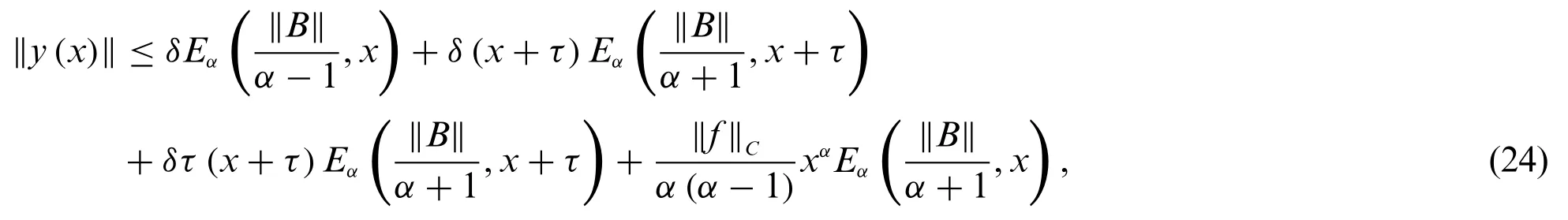
for allx∈W.Combining Eq.(19)with Eq.(24),we obtain‖y(x)‖<βfor allx∈W.This completes the proof.
Corollary 4.1.Letα=2 in Eq.(1).Then the system
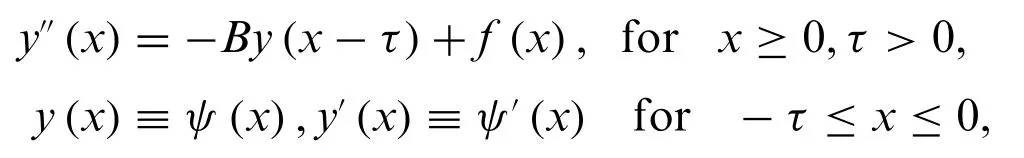
is finite time stable with respect to{0,W,τ,δ,β},δ <βif
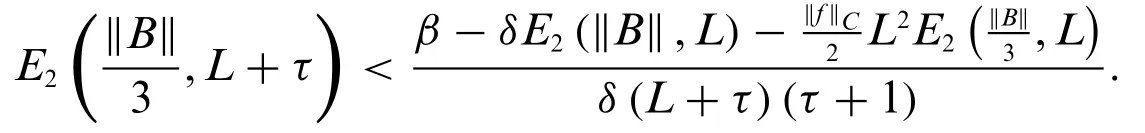
Remark 4.1.Letα= 2,B=B2in Eq.(1)such that the matrixBis a nonsingularn×nmatrix.Then the representation of solution Eq.(18)coincides with the conclusion of Theorems 1 and 2 in[12],which leads to the same of the finite time stability results in[27].
5 An Example
Consider the conformable delay differential equations
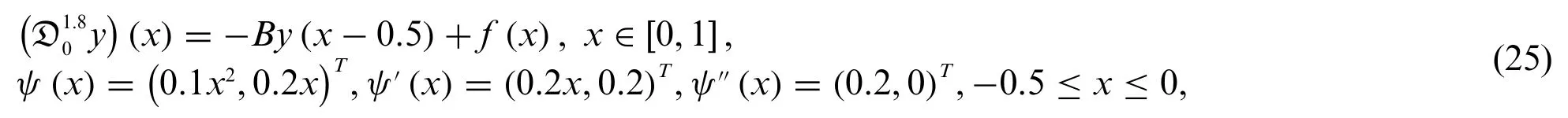
where
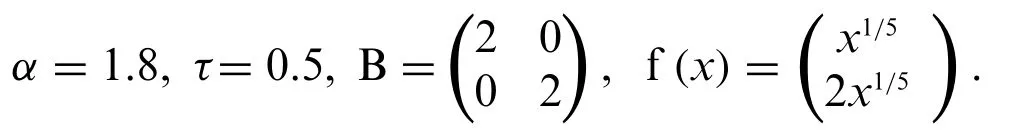
From Theorems 3.1 and 3.2,for all 0 ≤x≤1,and through a basic calculation,we can obtain
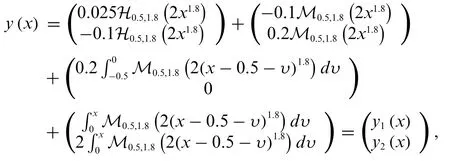
which implies that
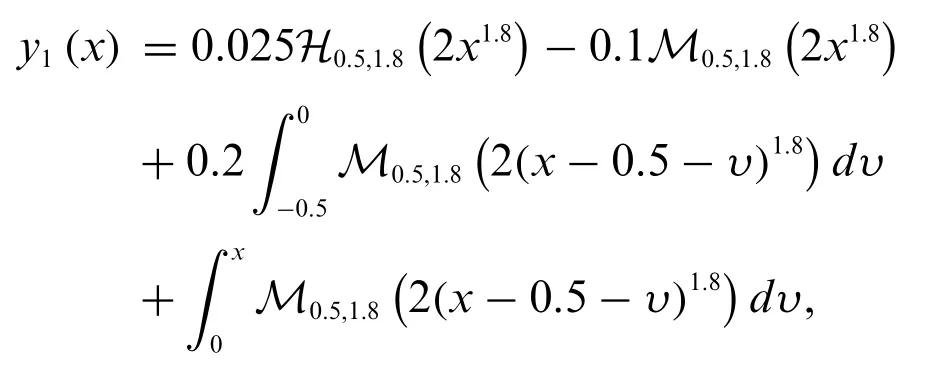
and
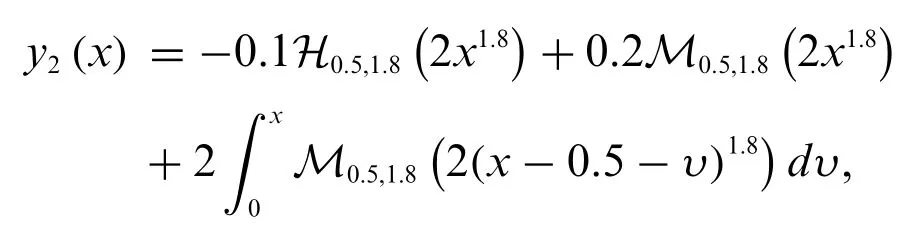
where
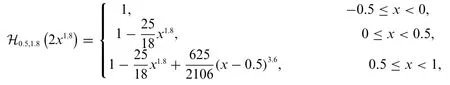
and
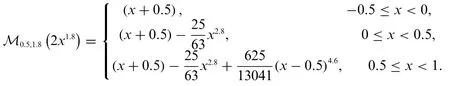
Thus the explicit solutions of Eq.(25)are
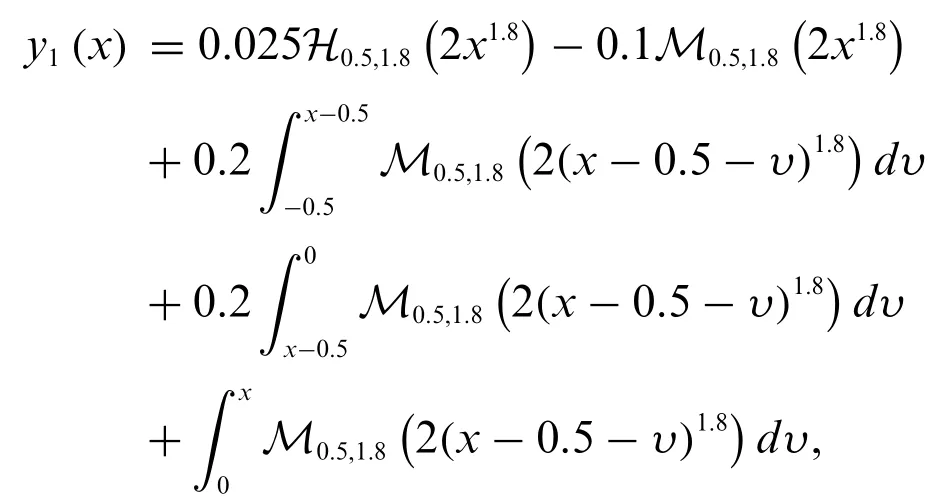
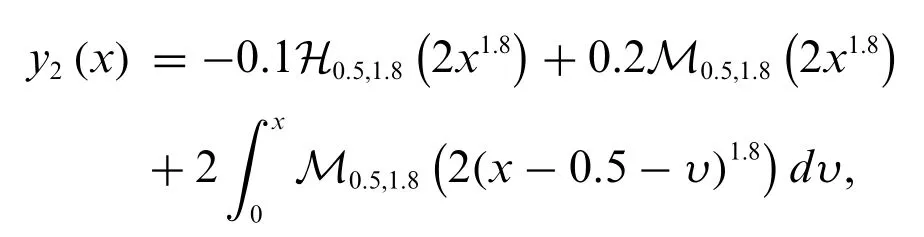
where 0 ≤x≤0.5,which implies that

and
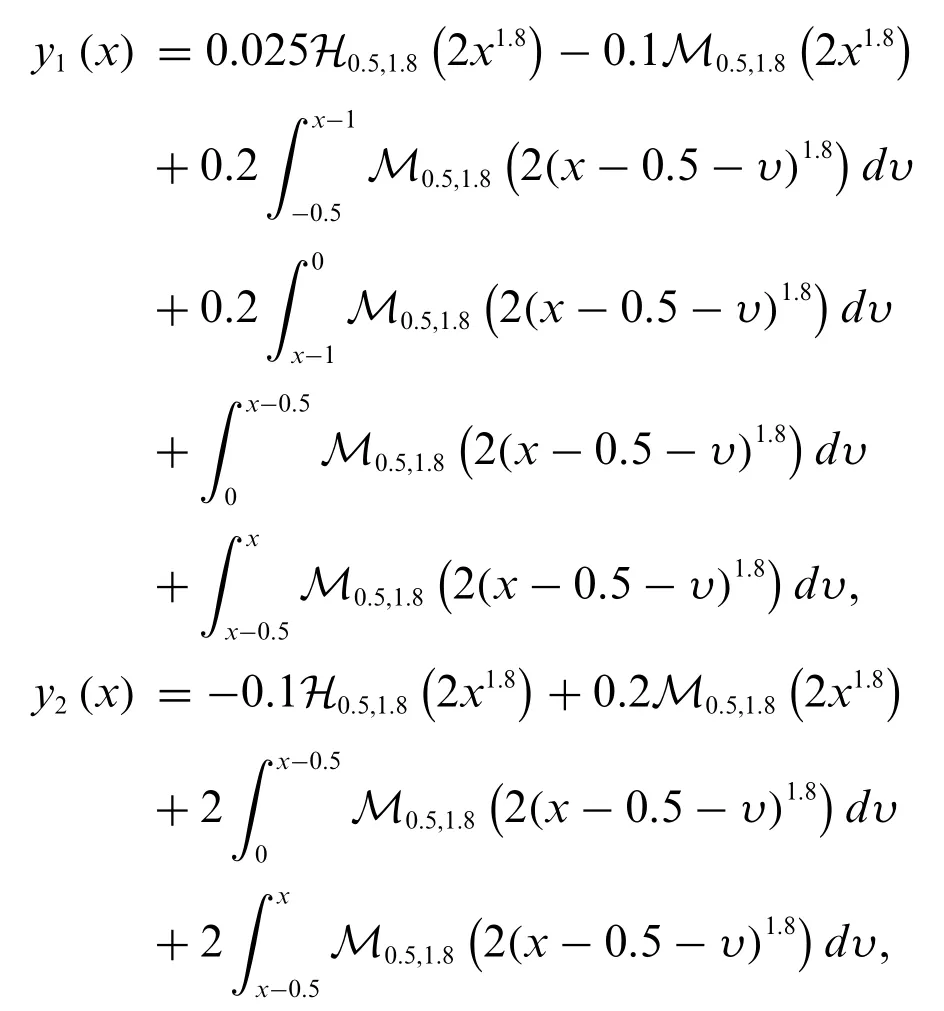
where 0.5 ≤x≤1,which implies that

By calculating we obtainη= max= 0.3,‖B‖ = 2,‖f‖C= 3,Eα=4.0104,= 1.4871,then we setδ= 0.31>0.3 =η.Fig.1 shows the statey(x)and the norm‖y(x)‖of Eq.(25).Now Theorem 4.1 implies that‖y(x)‖ ≤5.930254,we just takeβ=5.9303,which implies that‖y(x)‖<βand Eq.(25)is finite time stable.
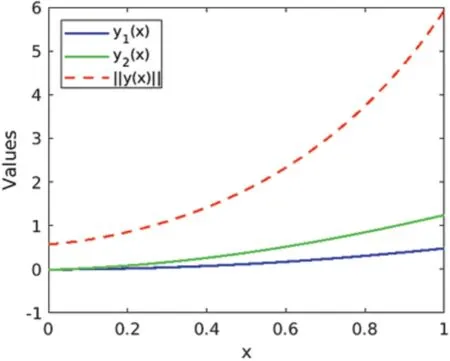
Figure 1:The state y(x)and||y(x)||of Eq.(25)
6 Conclusion
In this work,using new conformable delayed matrix functions,we derived explicit solutions of linear conformable fractional delay systems of orderα∈(1,2],which extend and improve the corresponding and existing ones in[12,13]in the case ofα=2 without any restrictions on the matrix coefficient of the linear part,by removing the condition thatBis a nonsingular matrix and replacing the matrix coefficient of the linear partB2in[12]by an arbitrary,not necessarily squared,matrix.In addition,using the formula of general solutions and a norm estimation of the conformable delayed matrix functions,we established some sufficient conditions for the finite time stability results,which extend and improve the existing ones in[27]in the case ofα= 2.Ultimately,an illustrative example was given to show the validity of the proposed results.
Following the topic of this paper,we outline some possible next research directions.The first direction will include applying the results of this paper on control problems for conformable fractional delay systems of orderα∈(1,2].The second direction is to consider the explicit solutions of linear conformable fractional delay systems of the form
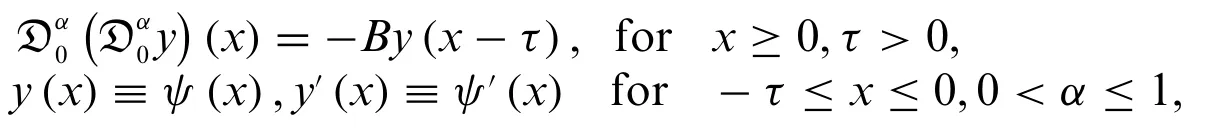
which lead to new results on stability and control problems.Depending on these results and delayed arguments,we will try to prove a generalized Lyapunov-type inequality for the conformable and sequential conformable boundary value problems
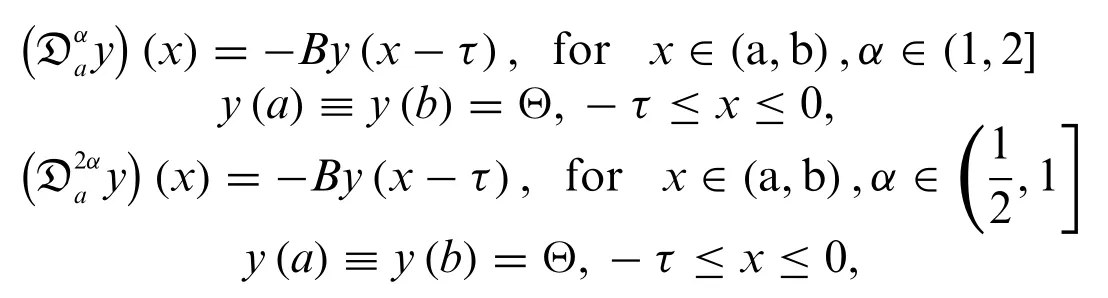
and
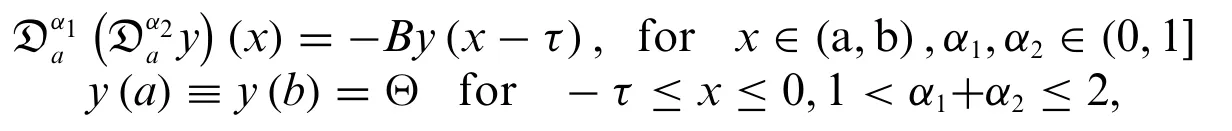
which leads to new results on the conformable Sturm-Liouville eigenvalue problem.
Acknowledgement:The authors would like to thank Princess Nourah bint Abdulrahman University Researchers Supporting Project No.(PNURSP2022R27),Princess Nourah bint Abdulrahman University,Riyadh,Saudi Arabia.
Funding Statement:Princess Nourah bint Abdulrahman University Researchers Supporting Project No.(PNURSP2022R27),Princess Nourah bint Abdulrahman University,Riyadh,Saudi Arabia.
Conflicts of Interest:The authors declare that they have no conflicts of interest to report regarding the present study.
 Computer Modeling In Engineering&Sciences2023年2期
Computer Modeling In Engineering&Sciences2023年2期
- Computer Modeling In Engineering&Sciences的其它文章
- Detecting Icing on the Blades of a Wind Turbine Using a Deep Neural Network
- Optimizing Big Data Retrieval and Job Scheduling Using Deep Learning Approaches
- Image Representations of Numerical Simulations for Training Neural Networks
- Structural Damage Identification Using Ensemble Deep Convolutional Neural Network Models
- Self-Triggered Consensus Filtering over Asynchronous Communication Sensor Networks
- State Estimation Moving Window Gradient Iterative Algorithm for Bilinear Systems Using the Continuous Mixed p-norm Technique
