Bounds on Fractional-Based Metric Dimension of Petersen Networks

Dalal Awadh Alrowaili,Mohsin Raza and Muhammad Javaid,＊

1Mathematics Department,College of Science,Jouf University,P.O.Box:2014,Sakaka,Saudi Arabia

2Department of Mathematics,School of Science,University of Management and Technology,Lahore,Pakistan

ABSTRACT The problem of investigating the minimum set of landmarks consisting of auto-machines(Robots)in a connected network is studied with the concept of location number or metric dimension of this network.In this paper,we study the latest type of metric dimension called as local fractional metric dimension(LFMD)and find its upper bounds for generalized Petersen networks GP(n,3),where n ≥7.For n ≥9.The limiting values of LFMD for GP(n,3)are also obtained as 1(bounded)if n approaches to infinity.

KEYWORDS Metric dimension;local fractional metric dimension;Petersen network;local resolving neighborhoods

1 Introduction

The idea of metric dimension (MD) was firstly introduced by Melter et al.[1].It has various applications in different areas such as the navigation system,image processing and drug discoveries.A network consists of nodes that are represented by vertices and connections between different vertices are denoted by edges.With the help of edges, an agent can change its position from one vertex to another.Some vertices are referred to as landmarks from which an agent can easily find its location in the network.The set with the minimum number of landmarks is known as the metric basis,and the cardinality of the aforesaid set is known as MD[2,3].

Moreover,the concept of MD in integer programming problem(IPP)was studied by Chartrand et al.[4].Later on, Oellermann et al.[5,6] produced more refined results of (IPP) through MD.Fehr et al.[7] also derived various results for different graphs which are used to solve relaxation problems by using MD.For more results on MD,see[8,9].

The idea of fractional metric dimension (FMD) for different networks flourished through the work of Arumugam et al.[10].Moreover,different networks are studied with the help of FMD such as hierarchical,Cartesian,corona,comb and lexicographic products[11-14].Yi[15]and Liu et al.[16]calculated FMD for permutation and generalized Jahangir networks.Moreover, the sharps bounds of FMD for all the connected networks are studied in [17].The idea of local fractional metric dimension(LFMD)came through the work of Aisyah et al.in which they computed the LFMD for the corona product of networks [18].Later on, Liu et al.[19] discussed the LFMD for a particular class of planar networks called by circular ladders and rotationally symmetric networks.Recently,Javaid et al.calculated the bounds of LFMD of connected and prism related networks in[20,21].

In this paper,we find local resolving neighborhood sets(LRNs)of generalized Petersen network GP(n,3)forn≥5.After that,we calculated the sharp bounds of the local fractional metric dimension with the help of LRNs.The organization of paper is:Section 1 describes the introduction,Section 2 presents the preliminaries,Section 3 includes the local fractional metric dimensions of the Generalized Petersen network and Section 4 presents the discussion and conclusion.

2 Preliminaries

Mathematically, a networkNconsists of vertices setV(N )and edges set E(N )with propertyE(N )⊆V(N )×V(N ).In the present study,only the simple networks without any loop or parallel edges are considered.The distance between two vertices is considered as the length(number of edges)of the shortest path existing between them.For more basic notions,we refer to[22,23].

For any connected graph,y∈V(N )can resolve pair{v,w} ∈V(N,ifd(y,v)/=d(y,w).LetTbe a set which is subset ofV(N )known as resolving set ofNif all pair of vertices inNare resolved by some vertices ofT.The cardinality of resolving set is denoted by |T|.The set having minimum cardinality among all the resolving sets ofNis called as metric dimension(MD).

Forvw∈E(N ),the local resolving neighborhood(LRN)setLR(vw)ofvwis defined asLR(vw)={x∈V(N ):d(x,v)/=d(x,w)}.A local resolving function (LRF) is a real valued functionφ:V(N )→[0,1] such thatφ(LR(vw))≥1 for eachLR(vw) ofN, whereAn LRFgis called minimal if there exists an other functionφ:V(N )→[0,1]such thatφ≤gandφ(u)/=g(u)for at least oneu∈V,that is not a LRF ofN.Ifthen LFMD ofNis defined as

dimlf(N )=min{|g|:gis a minimal LRF ofN}.For more detail,see[1,10,19].

Forn≥7,let GP(n,3)be a generalized Petersen network with vertex setV(GP(n,3))= {xi,yj:1 ≤i,j≤n}and edge setE(GP(n,3))={xixi+1:1 ≤i≤n-1}∪{xiyi:1 ≤i≤n}∪{xnx1}∪{yiyi+3:1 ≤i≤n-3}∪{yn-2y1,yn-1y2,yny3},where|V(GP(n,3))|=2n,|E(GP(n,3))|=3n(see Fig.1).

Now we present the following important result which will be frequently used in the main results.

Theorem 1:(see[15])LetN (VN,E(N ))be a connected network andLR(c)be a local resolving neighborhood for somec∈E(N ).If|LR(c)∩Y|≥αfor allc∈E(N ),then,,where,α=min{|LR(c)|:c∈E(N )},Y=∪{LR(c):|LR(c)|=α}.

Theorem 2:(see[15])For a connected network N,dimlf(N)=1 if N is bipartite.

Theorem 3:(see[20])LetN (VN,E(N ))be a connected network andLR(c)be a local resolving neighborhood set.Then,, where,γ=max{|LR(c)| :c∈E(N )} and 2 ≤γ≤|V(N )|.

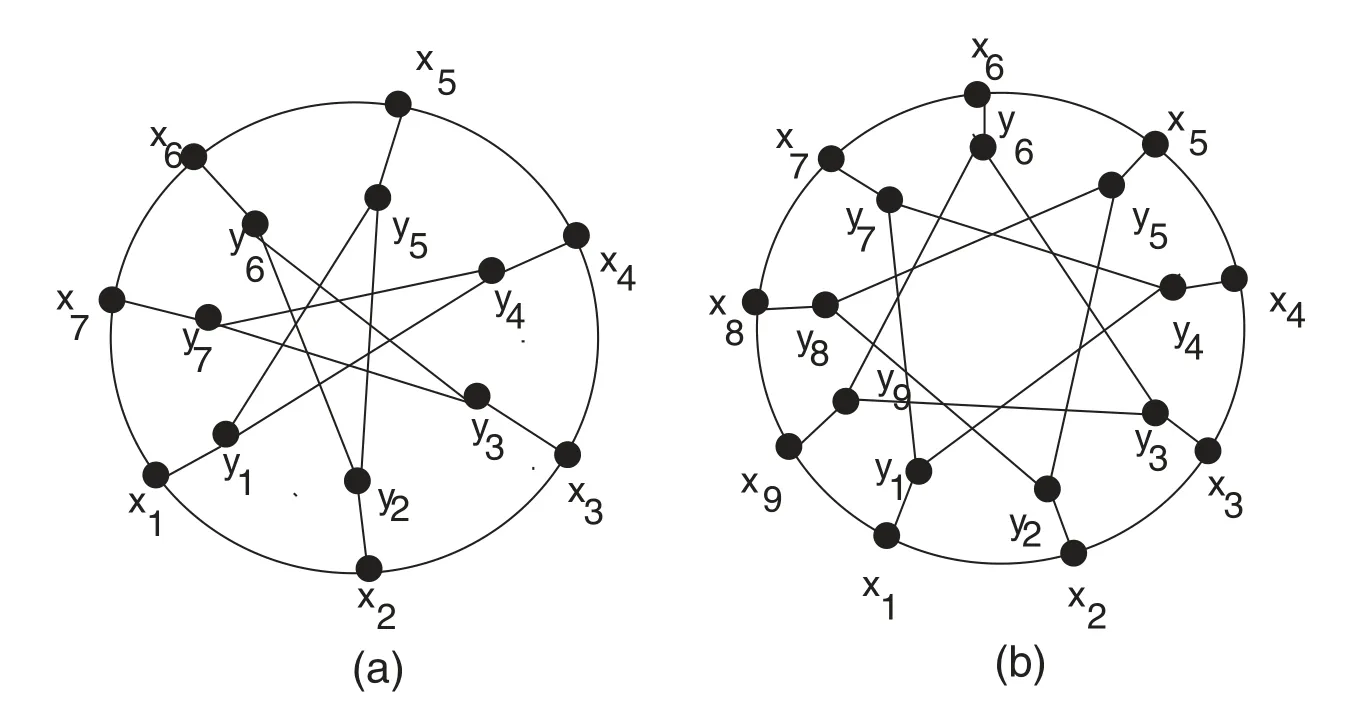
Figure 1:Petersen graphs(a)GP(6,3)and(b)GP(9,3)

3 Local Fractional Metric Dimension Generalized Petersen Network

This section deals with the main findings of the present studies.

Theorem 4.The LFMD of generalized Petersen network GP(n,3)forn= 7 is

Proof:The LRNs of GP(n,3)forn=7 are given by:

LR1=LR(x1x2)=V(GP(7,3))-{x5,y5},LR2=LR(x2x3)=V(GP(7,3))-{x6,y6},

LR3=LR(x3x4)=V(GP(7,3))-{x7,y7},LR4=LR(x4x5)=V(GP(7,3))-{x1,y1},

LR5=LR(x5x6)=V(GP(7,3))-{x2,y2},LR6=LR(x6x7)=V(GP(7,3))-{x3,y3},

LR7=LR(x7x1)=V(GP(7,3))-{x4,y4},LR8=LR(y1y4)=V(GP(7,3))-{y6},

LR9=LR(y2y5)=V(GP(7,3))-{y7},LR10=LR(y3y6)=V(GP(7,3))-{y1},

LR11=LR(y4y7)=V(GP(7,3))-{y2},LR12=LR(y5y1)=V(GP(7,3))-{y3},

LR13=LR(y6y2)=V(GP(7,3))-{y4},LR14=LR(y7y3)=V(GP(7,3))-{y5},

LR(x1y1)=V(GP(7,3))-{y2,y3,y6,y7}=LR(e1),

LR(x2y2)=V(GP(7,3))-{y3,y4,y7,y1}=LR(e2),

LR(x3y3)=V(GP(7,3))-{y4,y5,y1,y2}=LR(e3),

LR(x4y4)=V(GP(7,3))-{y5,y6,y2,y3}=LR(e4),

LR(x5y5)=V(GP(7,3))-{y6,y7,y3,y4}=LR(e5),

LR(x6y6)=V(GP(7,3))-{y7,y1,y4,y5}=LR(e6),

LR(x7y7)=V(GP(7,3))-{y1,y2,y5,y6}=LR(e7).

For 1 ≤m≤14 and 1 ≤j≤7 LRN are |LR(ej)| = 10＜|LRm|.Furthermore,Moreover,1 ≤j≤7,LR(ej)are pairwise nonempty.There exist a minimal LRFψ:V(GP(7,3))→[0,1]is defined asfor eachandψ(y)=0 for the vertices of GP(7,3)which are not inTherefore,by theorem 1,Since|V(GP(7,3))| =γ= 13,then by Theorem 3 we have(GP(7,3))(as GP(7,3)is not bipartite network).Therefore,

Lemma 1:Let GP(n,3)be Generalized Petersen network for,n≡3(mod6)andn≥9.Then,for 1 ≤i≤n-3, 1 ≤j≤n|LR(ej)| = |LR(ej=yiyi+3)| = 2n-6 = |LR(yn-2y1)| = |LR(yn-1y2)| =|LR(yny3)|.Moreover,

Proof:For,n≥9 andn≡3(mod6)the local resolving neighborhood of generalized Petersen network GP(n,3),for 1 ≤i≤n-3,1 ≤j≤n,p,

with |LR(ej)| = 2n- 6 and

Lemma 2:Let GP(n,3)be generalized Petersen network withn≡3(mod6)andn≥9,then,for 1 ≤i≤n,1 ≤j≤n.(a)|LR(ej)|＜|LR(xixi+1)|and

(b) |LR(ej)|＜|LR(xiyi)|and

Proof:(a)The local resolving neighborhood for 1 ≤i≤n,1 ≤j≤n,p,

with |LR(xixi+1)| = 2n-2＞2n-6 = |LR(ej)|, Therefore,

(b)The local resolving neighborhood for 1 ≤i≤n,1 ≤j≤n,

with|LR(xiyi)|=2n ＞2n-6=|LR(ej)|,Therefore,

Theorem 5:Let GP(n,3)withn≡ 3(mod6)be a generalized Petersen network, where|V(GP(n,3))|=2nandn≥9.Then,1 ≤dimlf (GP(n,3))≤

Proof:

Case 1:The LRNs of GP(n,3)forn=9 are given by:

LR1=LR(x1x2)=V(GP(9,3))-{x6,y6},LR2=LR(x2x3)=V(GP(9,3))-{x7,y7},

LR3=LR(x3x4)=V(GP(9,3))-{x8,y8},LR4=LR(x4x5)=V(GP(9,3))-{x9,y9},

LR5=LR(x5x6)=V(GP(9,3))-{x1,y1},LR6=LR(x6x7)=V(GP(9,3))-{x2,y2},

LR7=LR(x7x8)=V(GP(9,3))-{x3,y3},LR8=LR(x8x9)=V(GP(9,3))-{x4,y4},

LR9=LR(x9x1)=V(GP(9,3))-{x5,y5},LR10=LR(x1y1)=V(GP(9,3)),

LR11=LR(x2y2)=V(GP(9,3)),LR12=LR(x3y3)=V(GP(9,3)),

LR13=LR(x4y4)=V(GP(9,3)),LR14=LR(x5y5)=V(GP(9,3)),

LR15=LR(x6y6)=V(GP(9,3)),LR16=LR(x7y7)=V(GP(9,3)),

LR17=LR(x8y8)=V(GP(9,3)),LR18=LR(x9y9)=V(GP(9,3)),

LR(y1y4)=V(GP(9,3))-{x6,x7,x8,y6,y7,y8}=LR(e1),

LR(y2y5)=V(GP(9,3))-{x7,x8,x9,y7,y8,y9}=LR(e2),

LR(y3y6)=V(GP(9,3))-{x8,x9,x1,y8,y9,y1}=LR(e3),

LR(y4y7)=V(GP(9,3))-{x9,x1,x2,y9,y1,y2}=LR(e4),

LR(y5y8)=V(GP(9,3))-{x1,x2,x3,y1,y2,y3}=LR(e5),

LR(y6y9)=V(GP(9,3))-{x2,x3,x4,y2,y3,y4}=LR(e6),

LR(y7y1)=V(GP(9,3))-{x3,x4,x5,y3,y4,y5}=LR(e7),

LR(y8y2)=V(GP(9,3))-{x4,x5,x6,y4,y5,y6}=LR(e8),

LR(y9y3)=V(GP(9,3))-{x5,x6,x7,y5,y6,y7}=LR(e9).

For 1 ≤m≤18 and 1 ≤j≤9 LRN are |LR(ej)| = 12＜|LRm|.Furthermore,V(GP(9,3)),andMoreover,1 ≤j≤9,LR(ej)are pairwise nonempty.There exist a minimal LRFψ:V(GP(9,3))→[0,1]is defined asfor eachandψ(y)=0 for the vertices of GP(9,3)which are not inTherefore,by Theorem 1,Since |V(GP(9,3))| =γ= 18, then by Theorem 3≤dimlf (GP(9,3))implies 1 ≤dimlf(GP(9,3))(as GP(9,3)is not bipartite network).Therefore,1 ≤dimlf (GP(9,3))≤.

Case 2:The LRNs of GP(n,3)forn=15 are given by:

LR1=LR(x1x2)=V(GP(15,3))-{x9,y9},LR2=LR(x2x3)=V(GP(15,3))-{x10,y10},

LR3=LR(x3x4)=V(GP(15,3))-{x11,y11},LR4=LR(x4x5)=V(GP(15,3))-{x12,y12},

LR5=LR(x5x6)=V(GP(15,3))-{x13,y13},LR6=LR(x6x7)=V(GP(15,3))-{x14,y14},

LR7=LR(x7x8)=V(GP(15,3))-{x15,y15},LR8=LR(x8x9)=V(GP(15,3))-{x1,y1},

LR9=LR(x9x10)=V(GP(15,3))-{x2,y2},LR10=LR(x10x11)=V(GP(15,3))-{x3,y3},

LR11=LR(x11x12)=V(GP(15,3))-{x4,y4},LR12=LR(x12x13)=V(GP(15,3))-{x5,y5},

LR13=LR(x13x14)=V(GP(15,3))-{x6,y6},LR14=LR(x14x15)=V(GP(15,3))-{x7,y7},

LR15=LR(x15x1)=V(GP(15,3))-{x8,y8},LR16=LR(x1y1)=V(GP(15,3)),

LR17=LR(x2y2)=V(GP(15,3)),LR18=LR(x3y3)=V(GP(15,3)),

LR19=LR(x4y4)=V(GP(15,3)),LR20=LR(x5y5)=V(GP(15,3)),

LR21=LR(x6y6)=V(GP(15,3)),LR22=LR(x7y7)=V(GP(15,3)),

LR23=LR(x8y8)=V(GP(15,3)),LR24=LR(x9y9)=V(GP(15,3)),

LR25=LR(x10y10)=V(GP(15,3)),LR26=LR(x11y11)=V(GP(15,3)),

LR27=LR(x12y12)=V(GP(15,3)),LR28=LR(x13y13)=V(GP(15,3)),

LR29=LR(x14y14)=V(GP(15,3)),LR30=LR(x15y15)=V(GP(15,3)),

LR(y1y4)=V(GP(15,3))-{x9,x10,x11,y9,y10,y11}=LR(e1),

LR(y2y5)=V(GP(15,3))-{x10,x11,x12,y10,y11,y12}=LR(e2),

LR(y3y6)=V(GP(15,3))-{x11,x12,x13,y11,y12,y13}=LR(e3),

LR(y4y7)=V(GP(15,3))-{x12,x13,x14,y12,y13,y14}=LR(e4),

LR(y5y8)=V(GP(15,3))-{x13,x14,x15,y13,y14,y15}=LR(e5),

LR(y6y9)=V(GP(15,3))-{x14,x15,x1,y14,y15,y1}=LR(e6),

LR(y7y10)=V(GP(15,3))-{x15,x1,x2,y15,y1,y2}=LR(e7),

LR(y8y11)=V(GP(15,3))-{x1,x2,x3,y1,y2,y3}=LR(e8),

LR(y9y12)=V(GP(15,3))-{x2,x3,x4,y2,y3,y4}=LR(e9),

LR(y10y13)=V(GP(15,3))-{x3,x4,x5,y3,y4,y5}=LR(e10),

LR(y11y14)=V(GP(15,3))-{x4,x5,x6,y4,y5,y6}=LR(e11),

LR(y12y15)=V(GP(15,3))-{x5,x6,x7,y5,y6,y7}=LR(e12),

LR(y13y1)=V(GP(15,3))-{x6,x7,x8,y6,y7,y8}=LR(e13),

LR(y14y2)=V(GP(15,3))-{x7,x8,x9,y7,y8,y9}=LR(e14),

LR(y15y3)=V(GP(15,3))-{x8,x9,x10,y8,y9,y10}=LR(e15).

For 1 ≤m≤30 and 1 ≤j≤15 LRN are |LR(ej)| = 24＜|LRm|.Furthermore,V(GP(15,3)),Moreover,1 ≤j≤15,LR(ej)are pairwise nonempty.There exist a minimal LRFψ:V(GP(15,3))→[0,1]is defined asfor eachyandψ(y)=0 for the vertices of GP(15,3)which are not inTherefore,by Theorem 1,Since |V(GP(15,3))| =γ= 30, then by Theorem 3 we have≤dimlf (GP(15,3))implies 1 ≤dimlf(GP(15,3)).As GP(15,3)is not bipartite network therefore,1 ≤dimlf (GP(15,3))≤.

Case 3:For 1 ≤i≤n, 1 ≤j≤nandn≥19,LR(ej)=LR(yiyi+3),LR(xixi+1),LR(xiyi).By Lemmas 1,2,we have(i)|LR(xixi+1)|,The intersection of LRS having minimum cardinality is not empty.Therefore, there exist a mimimal local resolvingψ＇:V(GP(n,3))→[0,1]such that |ψ＇|＜|ψ|, where the minimal LRFψ:V(GP(n,3))→ [0,1] is defined asφ(v)=

Lemma 3:Let GP(n,3)be Generalized Petersen network for,n≡3(mod6)andn≥11.Then,for 1 ≤i≤n-3, 1 ≤j≤n|LR(ej)| = |LR(ej=yiyi+3)| = 2n-6 = |LR(yn-2y1)| = |LR(yn-1y2)| =|LR(yny3)|.Moreover,

Proof:For,n≥11 andn≡3(mod6)the local resolving neighborhood of generalized Petersen network GP(n,3),for 1 ≤i≤n-3,1 ≤j≤n,

with |LR(ej)| = 2n- 6 andand we have

Lemma 4:Let GP(n,3)be generalized Petersen network withn≡3(mod6)andn≥11,then,for 1 ≤i≤n,1 ≤j≤n.

(a) |LR(ej)|＜|LR(xixi+1)|and

(b) |LR(ej)|＜|LR(xiyi)|and

Proof:(a)The local resolving neighborhood for 1 ≤i≤n,1 ≤j≤n,p,

with |LR(xixi+1)| = 2n-2＞2n-6 = |LR(ej)|, Therefore,

(b)The local resolving neighborhood for 1 ≤i≤n,1 ≤j≤n,

with|LR(xiyi)|=2n ＞2n-6=|LR(ej)|,Therefore,

Theorem 6:Let GP(n,3)n≡5(mod6)be a generalized Petersen network,where|V(GP(n,2))|=2nandn≥11.Then,1 ≤dimlf (GP(n,3))≤

Proof:

The LRNs of GP(n,3)forn=11 are given by:

LR1=LR(x1x2)=V(GP(11,3))-{x7,y7},LR2=LR(x2x3)=V(GP(11,3))-{x8,y8},

LR3=LR(x3x4)=V(GP(11,3))-{x9,y9},LR4=LR(x4x5)=V(GP(11,3))-{x10,y10},

LR5=LR(x5x6)=V(GP(11,3))-{x11,y11},LR6=LR(x6x7)=V(GP(11,3))-{x1,y1},

LR7=LR(x7x8)=V(GP(11,3))-{x2,y2},LR8=LR(x8x9)=V(GP(11,3))-{x3,y3},

LR9=LR(x9x10)=V(GP(11,3))-{x4,y4},LR10=LR(x10x11)=V(GP(11,3))-{x5,y5},

LR11=LR(x11x1)=V(GP(11,3))-{x6,y6},LR12=LR(x1y1)=V(GP(11,3)),

LR13=LR(x2y2)=V(GP(11,3)),LR14=LR(x3y3)=V(GP(11,3)),

LR15=LR(x4y4)=V(GP(11,3)),LR16=LR(x5y5)=V(GP(11,3)),

LR17=LR(x6y6)=V(GP(11,3)),LR18=LR(x7y7)=V(GP(11,3)),

LR19=LR(x8y8)=V(GP(11,3)),LR20=LR(x9y9)=V(GP(11,3)),

LR21=LR(x10y10)=V(GP(11,3)),LR22=LR(x11y11)=V(GP(11,3)),

LR(y1y4)=V(GP(11,3))-{x6,x8,x10,y2,y3,y8}=LR(e1),

LR(y2y5)=V(GP(11,3))-{x7,x9,x11,y3,y4,y9}=LR(e2),

LR(y3y6)=V(GP(11,3))-{x8,x10,x1,y4,y5,y10}=LR(e3),

LR(y4y7)=V(GP(11,3))-{x9,x11,x2,y5,y6,y11}=LR(e4),

LR(y5y8)=V(GP(11,3))-{x10,x1,x3,y6,y7,y1}=LR(e5),

LR(y6y9)=V(GP(11,3))-{x11,x2,x4,y7,y8,y2}=LR(e6),

LR(y7y10)=V(GP(11,3))-{x1,x3,x5,y8,y9,y3}=LR(e7),

LR(y8y11)=V(GP(11,3))-{x2,x4,x6,y9,y10,y4}=LR(e8),

LR(y9y1)=V(GP(11,3))-{x3,x5,x7,y10,y11,y5}=LR(e9),

LR(y10y2)=V(GP(11,3))-{x4,x6,x8,y11,y1,y6}=LR(e10),

LR(y11y3)=V(GP(11,3))-{x5,x7,x9,y1,y2,y7}=LR(e11).

For 1 ≤m≤22 and 1 ≤j≤11 LRN are |LR(ej)| = 16＜|LRm|.Furthermore,V(GP(11,2)),Moreover, 1 ≤j≤11,LR(ej)are pairwise nonempty.There exist a minimal LRFψ:V(GP(14,2))→[0,1] is defined asfor eachandψ(y)=0 for the vertices of GP(11,3)which are not inTherefore, by Theorem 1,.Since |V(GP(11,3))| =γ= 22, then by Theorem 3 we have(GP(11,3))implies 1 ≤dimlf(GP(11,3)).As GP(11,3)is not bipartite network therefore,1 ≤dimlf (GP(11,3))≤

Case 2:The LRNs of GP(n,3)forn=17 are given by:

LR1=LR(x1x2)=V(GP(17,3))-{x10,y10},LR2=LR(x2x3)=V(GP(17,3))-{x11,y11},

LR3=LR(x3x4)=V(GP(17,3))-{x12,y12},LR4=LR(x4x5)=V(GP(17,3))-{x13,y13},

LR5=LR(x5x6)=V(GP(17,3))-{x14,y14},LR6=LR(x6x7)=V(GP(17,3))-{x15,y15},

LR7=LR(x7x8)=V(GP(17,3))-{x16,y16},LR8=LR(x8x9)=V(GP(17,3))-{x17,y17},

LR9=LR(x9x10)=V(GP(17,3))-{x1,y1},LR10=LR(x10x11)=V(GP(17,3))-{x2,y2},

LR11=LR(x11x12)=V(GP(17,3))-{x3,y3},LR12=LR(x12x13)=V(GP(17,3))-{x4,y4},

LR13=LR(x13x14)=V(GP(17,3))-{x5,y5},LR14=LR(x14x15)=V(GP(17,3))-{x6,y6},

LR15=LR(x15x16)=V(GP(17,3))-{x7,y7},LR16=LR(x16x17)=V(GP(17,3))-{x8,y8},

LR17=LR(x17x1)=V(GP(17,3))-{x9,y9},LR18=LR(x1y1)=V(GP(17,3)),

LR19=LR(x2y2)=V(GP(17,3)),LR20=LR(x3y3)=V(GP(17,3)),

LR21=LR(x4y4)=V(GP(17,3)),LR22=LR(x5y5)=V(GP(17,3)),

LR23=LR(x6y6)=V(GP(17,3)),LR24=LR(x7y7)=V(GP(17,3)),

LR25=LR(x8y8)=V(GP(17,3)),LR26=LR(x9y9)=V(GP(17,3)),

LR27=LR(x10y10)=V(GP(17,3)),LR28=LR(x11y11)=V(GP(17,3)),

LR29=LR(x12y12)=V(GP(17,3)),LR30=LR(x13y13)=V(GP(17,3)),

LR31=LR(x14y14)=V(GP(17,3)),LR32=LR(x15y15)=V(GP(17,3)),

LR33=LR(x16y16)=V(GP(17,3)),LR34=LR(x17y17)=V(GP(17,3)),

LR(y1y4)=V(GP(17,3))-{x9,x11,x13,y6,y11,y16}=LR(e1),

LR(y2y5)=V(GP(17,3))-{x10,x12,x14,y7,y12,y17}=LR(e2),

LR(y3y6)=V(GP(17,3))-{x11,x13,x15,y8,y13,y1}=LR(e3),

LR(y4y7)=V(GP(17,3))-{x12,x14,x16,y9,y14,y2}=LR(e4),

LR(y5y8)=V(GP(17,3))-{x13,x15,x17,y10,y15,y3}=LR(e5),

LR(y6y9)=V(GP(17,3))-{x14,x16,x1,y11,y16,y4}=LR(e6),

LR(y7y10)=V(GP(17,3))-{x15,x17,x2,y12,y17,y5}=LR(e7),

LR(y8y11)=V(GP(17,3))-{x16,x1,x3,y13,y1,y6}=LR(e8),

LR(y9y12)=V(GP(17,3))-{x17,x2,x4,y14,y2,y7}=LR(e9),

LR(y10y13)=V(GP(17,3))-{x1,x3,x5,y15,y3,y8}=LR(e10),

LR(y11y14)=V(GP(17,3))-{x2,x4,x6,y16,y4,y9}=LR(e11),

LR(y12y15)=V(GP(17,3))-{x3,x5,x7,y17,y5,y10}=LR(e12),

LR(y13y16)=V(GP(17,3))-{x4,x6,x8,y1,y6,y11}=LR(e13),

LR(y14y17)=V(GP(17,3))-{x5,x7,x9,y2,y7,y12}=LR(e14),

LR(y15y1)=V(GP(17,3))-{x6,x8,x10,y3,y8,y13}=LR(e15),

LR(y16y2)=V(GP(17,3))-{x7,x9,x11,y4,y9,y14}=LR(e16),

LR(y17y3)=V(GP(17,3))-{x8,x10,x12,y5,y10,y15}=LR(e17).

For 1 ≤m≤34 and 1 ≤j≤17 LRN are |LR(ej)| = 28＜|LRm|.Furthermore,V(GP(17,3)),Moreover, 1 ≤j≤17,LR(ej)are pairwise nonempty.There exist a minimal LRFψ:V(GP(17,3))→[0,1] is defined asfor eachandψ(y)=0 for the vertices of GP(17,3)which are not inTherefore,by Theorem 1,

Case 3:For 1 ≤i≤n, 1 ≤j≤nandn≥23,LR(ej)=LR(yiyi+3),LR(xixi+1),LR(xiyi).By Lemmas 3,4,we have(i)|LR(xixi+1)|,LR(xiyi)≥|LR(ej)|=2n-6=α,(ii)The intersection of LRS having minimum cardinality is not empty.Therefore, there exist a mimimal local resolvingψ＇:V(GP(n,3))→[0,1]such that |ψ＇|＜|ψ|, where the minimal LRFψ:V(GP(n,3))→ [0,1] is defined asφ(v)=

Lemma 5:Let GP(n,3)be Generalized Petersen network for,n≡1(mod6)andn≥13.Then,for 1 ≤i≤n-3, 1 ≤j≤n|LR(ej)| = |LR(ej=yiyi+3)| = 2n-6 = |LR(yn-2y1)| = |LR(yn-1y2)| =|LR(yny3)|.Moreover,

Proof:For,n≥13 andn≡3(mod6)the local resolving neighborhood of generalized Petersen network GP(n,3),for 1 ≤i≤n-3,1 ≤j≤n,

with |LR(ej)| = 2n- 6 andand we have

Lemma 6:Let GP(n,3)be generalized Petersen network withn≡3(mod6)andn≥13,then,for 1 ≤i≤n,1 ≤j≤n.(a)|LR(ej)|＜|LR(xixi+1)|and

(b) |LR(ej)|＜|LR(xiyi)|and

Proof:(a)The local resolving neighborhood for 1 ≤i≤n,1 ≤j≤n,p,

with |LR(xixi+1)| = 2n-2＞2n-6 = |LR(ej)|, Therefore,

(b)The local resolving neighborhood for 1 ≤i≤n,1 ≤j≤n,

with|LR(xiyi)|=2n ＞2n-6=|LR(ej)|,Therefore,

Theorem 6:Let GP(n,3)withn≡ 3(mod6)be a generalized Petersen network, where|V(GP(n,3))|=2nandn≥13.Then,

Proof:

The LRNs of GP(n,3)forn=13 are given by:

LR1=LR(x1x2)=V(GP(13,3))-{x8,y8},LR2=LR(x2x3)=V(GP(13,3))-{x9,y9},

LR3=LR(x3x4)=V(GP(13,3))-{x10,y10},LR4=LR(x4x5)=V(GP(13,3))-{x11,y11},

LR5=LR(x5x6)=V(GP(13,3))-{x12,y12},LR6=LR(x6x7)=V(GP(13,3))-{x13,y13},

LR7=LR(x7x8)=V(GP(13,3))-{x1,y1},LR8=LR(x8x9)=V(GP(13,3))-{x2,y2},

LR9=LR(x9x10)=V(GP(13,3))-{x3,y3},LR10=LR(x10x11)=V(GP(13,3))-{x4,y4},

LR11=LR(x11x12)=V(GP(13,3))-{x5,y5},LR12=LR(x12x13)=V(GP(13,3))-{x6,y6},

LR13=LR(x13x1)=V(GP(13,3))-{x7,y7},LR14=LR(x1y1)=V(GP(13,3)),

LR15=LR(x2y2)=V(GP(13,3)),LR16=LR(x3y3)=V(GP(13,3)),

LR17=LR(x4y4)=V(GP(13,3)),LR18=LR(x5y5)=V(GP(13,3)),

LR19=LR(x6y6)=V(GP(13,3)),LR20=LR(x7y7)=V(GP(13,3)),

LR21=LR(x8y8)=V(GP(13,3)),LR22=LR(x9y9)=V(GP(13,3)),

LR23=LR(x10y10)=V(GP(13,3)),LR24=LR(x11y11)=V(GP(13,3)),

LR25=LR(x12y12)=V(GP(13,3)),LR26=LR(x13y13)=V(GP(13,3)),

LR(y1y4)=V(GP(13,3))-{x8,x9,x10,y5,y9,y13}=LR(e1),

LR(y2y5)=V(GP(13,3))-{x9,x10,x11,y6,y10,y1}=LR(e2),

LR(y3y6)=V(GP(13,3))-{x10,x11,x12,y7,y11,y2}=LR(e3),

LR(y4y7)=V(GP(13,3))-{x11,x12,x13,y8,y12,y3}=LR(e4),

LR(y5y8)=V(GP(13,3))-{x12,x13,x1,y9,y13,y4}=LR(e5),

LR(y6y9)=V(GP(13,3))-{x13,x1,x2,y10,y1,y5}=LR(e6),

LR(y7y10)=V(GP(13,3))-{x1,x2,x3,y11,y2,y6}=LR(e7),

LR(y8y11)=V(GP(13,3))-{x2,x3,x4,y12,y3,y7}=LR(e8),

LR(y9y12)=V(GP(13,3))-{x3,x4,x5,y13,y4,y8}=LR(e9),

LR(y10y13)=V(GP(13,3))-{x4,x5,x6,y1,y5,y9}=LR(e10),

LR(y11y1)=V(GP(13,3))-{x5,x6,x7,y2,y6,y10}=LR(e11),

LR(y12y2)=V(GP(13,3))-{x6,x7,x8,y3,y7,y11}=LR(e12),

LR(y13y3)=V(GP(13,3))-{x7,x8,x9,y4,y8,y12}=LR(e13).

For 1 ≤m≤26 and 1 ≤j≤13 LRN are |LR(ej)| = 20＜|LRm|.Furthermore,V(GP(13,3)),andMoreover, 1 ≤j≤13,LR(ej)are pairwise nonempty.There exist a minimal LRFψ:V(GP(13,2))→[0,1] is defined asfor eachandψ(y)=0 for the vertices of GP(13,3)which are not inTherefore, by Theorem 1,Since |V(GP(13,3))| =γ= 26, then by Theorem 3 we have(GP(13,3))implies 1 ≤dimlf(GP(13,3)).As GP(13,3)is not bipartite network,therefore,1 ≤dimlf

Case 2:The LRNs of GP(n,3)forn=19 are given by:

LR1=LR(x1x2)=V(GP(19,3))-{x11,y11},LR2=LR(x2x3)=V(GP(19,3))-{x12,y12},

LR3=LR(x3x4)=V(GP(19,3))-{x13,y13},LR4=LR(x4x5)=V(GP(19,3))-{x14,y14},

LR5=LR(x5x6)=V(GP(19,3))-{x15,y15},LR6=LR(x6x7)=V(GP(19,3))-{x16,y16},

LR7=LR(x7x8)=V(GP(19,3))-{x17,y17},LR8=LR(x8x9)=V(GP(19,3))-{x18,y18},

LR9=LR(x9x10)=V(GP(19,3))-{x19,y19},LR10=LR(x10x11)=V(GP(19,3))-{x1,y1},

LR11=LR(x11x12)=V(GP(19,3))-{x2,y2},LR12=LR(x12x13)=V(GP(19,3))-{x3,y3},

LR13=LR(x13x14)=V(GP(19,3))-{x4,y4},LR14=LR(x14x15)=V(GP(19,3))-{x5,y5},

LR15=LR(x15x16)=V(GP(19,3))-{x6,y6},LR16=LR(x16x17)=V(GP(19,3))-{x7,y7},

LR17=LR(x17x18)=V(GP(19,3))-{x8,y8},LR18=LR(x18x19)=V(GP(19,3))-{x9,y9},

LR19=LR(x19x1)=V(GP(19,3))-{x10,y10},

LR20=LR(x1y1)=V(GP(19,3)),

LR21=LR(x2y2)=V(GP(19,3)),LR22=LR(x3y3)=V(GP(19,3)),

LR23=LR(x4y4)=V(GP(19,3)),LR24=LR(x5y5)=V(GP(19,3)),

LR25=LR(x6y6)=V(GP(19,3)),LR26=LR(x7y7)=V(GP(19,3)),

LR27=LR(x8y8)=V(GP(19,3)),LR28=LR(x9y9)=V(GP(19,3)),

LR29=LR(x10y10)=V(GP(19,3)),LR30=LR(x11y11)=V(GP(19,3)),

LR31=LR(x12y12)=V(GP(19,3)),LR32=LR(x13y13)=V(GP(19,3)),

LR33=LR(x14y14)=V(GP(19,3)),LR34=LR(x15y15)=V(GP(19,3)),

LR35=LR(x16y16)=V(GP(19,3)),LR36=LR(x17y17)=V(GP(19,3)),

LR37=LR(x18y18)=V(GP(19,3)),LR38=LR(x19y19)=V(GP(19,3)),

LR(y1y4)=V(GP(19,3))-{x11,x12,x13,y8,y12,y16}=LR(e1),

LR(y2y5)=V(GP(19,3))-{x12,x13,x14,y9,y13,y17}=LR(e2),

LR(y3y6)=V(GP(19,3))-{x13,x14,x15,y10,y14,y18}=LR(e3),

LR(y4y7)=V(GP(19,3))-{x14,x15,x16,y11,y15,y19}=LR(e4),

LR(y5y8)=V(GP(19,3))-{x15,x16,x17,y12,y16,y1}=LR(e5),

LR(y6y9)=V(GP(19,3))-{x16,x17,x18,y13,y17,y2}=LR(e6),

LR(y7y10)=V(GP(19,3))-{x17,x18,x19,y14,y18,y3}=LR(e7),

LR(y8y11)=V(GP(19,3))-{x18,x19,x1,y15,y19,y4}=LR(e8),

LR(y9y12)=V(GP(19,3))-{x19,x1,x2,y16,y1,y5}=LR(e9),

LR(y10y13)=V(GP(19,3))-{x1,x2,x3,y17,y2,y6}=LR(e10),

LR(y11y14)=V(GP(19,3))-{x2,x3,x4,y18,y3,y7}=LR(e11),

LR(y12y15)=V(GP(19,3))-{x3,x4,x5,y19,y4,y8}=LR(e12),

LR(y13y16)=V(GP(19,3))-{x4,x5,x6,y1,y5,y9}=LR(e13),

LR(y14y17)=V(GP(19,3))-{x5,x6,x7,y2,y6,y10}=LR(e14),

LR(y15y18)=V(GP(19,3))-{x6,x7,x8,y3,y7,y11}=LR(e15),

LR(y16y19)=V(GP(19,3))-{x7,x8,x9,y4,y8,y12}=LR(e16),

LR(y17y1)=V(GP(19,3))-{x8,x9,x10,y5,y9,y13}=LR(e17),

LR(y18y2)=V(GP(19,3))-{x9,x10,x11,y6,y10,y14}=LR(e18),

LR(y19y3)=V(GP(19,3))-{x10,x11,x12,y7,y11,y15}=LR(e19).

For 1 ≤m≤38 and 1 ≤j≤19 LRN are |LR(ej)| = 32＜|LRm|.Furthermore,V(GP(19,3)),Moreover, 1 ≤j≤19,LR(ej)are pairwise nonempty.There exist a minimal LRFψ:V(GP(19,3))→[0,1] is defined asfor eachandψ(y)=0 for the vertices of GP(19,3)which are not inTherefore, by Theorem 1,Since |V(GP(19,3))| =γ= 38, then by Theorem 3 we have(GP(19,3))implies 1 ≤dimlf(GP(19,3)).As GP(19,3)is not bipartite network therefore,1 ≤dimlf (GP(19,3))≤

Case 3:For 1 ≤i≤n, 1 ≤j≤nandn≥25,LR(ej)=LR(yiyi+3),LR(xixi+1),LR(xiyi).By Lemma 5,6,we have(i)|LR(xixi+1)|,LR(xiyi)≥|LR(ej)|=2n-6=α,(ii)The intersection of LRS having minimum cardinality is not empty.Therefore, there exist a mimimal local resolvingψ＇:V(GP(n,3))→[0,1]such that |ψ＇|＜|ψ|, where the minimal LRFψ:V(GP(n,3))→ [0,1] is defined asφ(v)=

Theorem 7.The LFMD of generalized Petersen network GP(n,3)forn≥8 andn≡0(mod2)is 1.

Proof:As generalized Petersen network GP(n,3)forn≥8 andn≡0(mod2)is bipartite network.Therefore,by Theorem 2 we havedimlf(GP(n,3))=1.

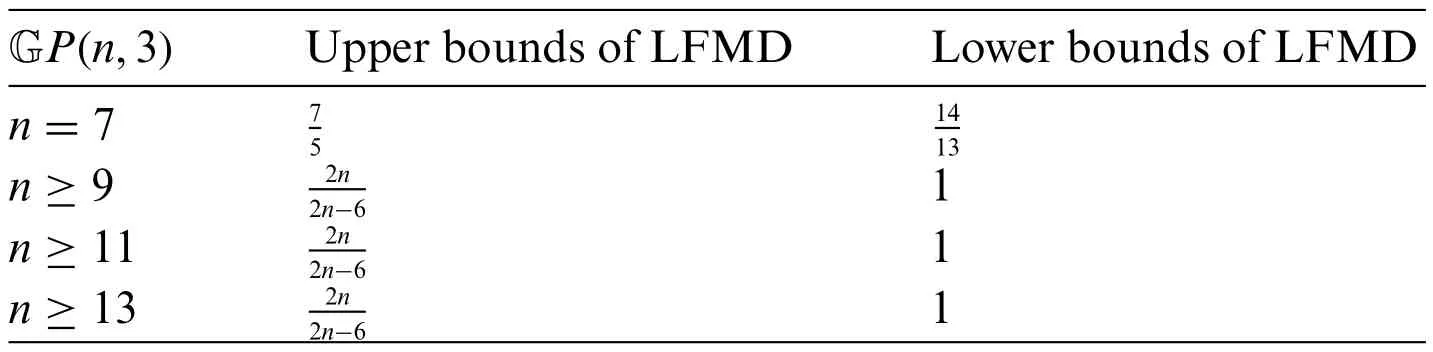
Table 1:Upper and lower bounds of LFMD of generalized petersen GP(n,3)network for,n=7,n ≥9 for n ≡3(mod 6),n ≥11 for n ≡5(mod 6),and n ≥13 for n ≡1(mod 6)

Table 2: Limiting values of LFMD of generalized petersen GP(n,3) network for, n ≥9 for n ≡3(mod 6),n ≥11 for n ≡5(mod 6),and n ≥13 for n ≡1(mod 6)

4 Discussion and Conclusion

In this paper,we have investigated the LFMD generalized Petersen network GP(n,3)forn≥7 with the exact value of lower and upper bounds.We have also checked the bounded and unbounded behavior of networks and found that forn≥8 andn≡0(mod2)GP(n,3)is bipartite Network having LFMD is 1.The details of computed values of LFMD are given in Tables 1 and 2.Even before the aforementioned tables,we illustrate Theorem 5 with the help of a example finding LFMD for the generalized Petersen graph withn= 9.By Fig.1b and Theorem 5 (Case A), it can be observed that the LRN sets of thee edgesy1y4,y2y5,y3y6,y4y7,y5y8,y7y8,y8y2,y9y3have the cardinality of 12 which is minimum among all the other LRNs.Moreover, the union of these LRNs is equal to the order of GP(n,3).The cardinality of the other LRNs with the intersection of this union is larger or equal to 12.By Theorem 1,In addition, the cardinality of LRNs with maximum cardinality is 18 consequently by Theorem 3,dimlfGP(9,3)≥Therefore,

Acknowledgement:The authors pay their sincerest thanks and deepest gratitude towards the referees for their valuable and precious comments that has helped us to soar up the manuscript to present level.

Funding Statement:The first author(Dalal Awadh Alrowaili)was funded by the Deanship of Scientific Research at Jouf University under Grant No.DSR-2021-03-0301.The third author (Muhammad Javaid)is supported by the Higher Education Commission of Pakistan through the National Research Program for Universities Grant No.20-16188/NRPU/R&D/HEC/2021 2021.

Conflicts of Interest:The authors declare that they have no conflicts of interest to report regarding the present study.