Modifications of the Optimal Auxiliary Function Method to Fractional Order Fornberg-Whitham Equations

Hakeem Ullah,Mehreen Fiza,＊,Ilyas Khan,Abd Allah A.Mosa,Saeed Islam and Abdullah Mohammed

1Department of Mathematics,Abdul Wali Khan University,Mardan,23200,Pakistan

2Department of Mathematics,College of Science Al-Zulfi Majmmah University,Al-Majmmah,11952,Saudi Arabia

3Department of Mathematics and Statistics,College of Science,Taif University,Taif,21944,Saudi Arabia

4University Research Centre,Future University in Egypt,New Cairo,11745,Egypt

ABSTRACT In this paper,we present a new modification of the newly developed semi-analytical method named the Optimal Auxilary Function Method (OAFM) for fractional-order equations using the Caputo operator, which is named FOAFM.The mathematical theory of FOAFM is presented and the effectiveness of this method is proven by using it with well-known Fornberg-Whitham Equations(FWE).The FOAFM results are compared with other method results along with their exact solutions with the help of tables and plots to prove the validity of FOAFM.A rapidly convergent series solution is obtained from FOAFM and is validated by comparison with other results.The analysis proves that our method is simply applicable,contains less computational work,and is rapidly convergent to the exact solution at the first iteration.A series solution to the problem is obtained with the help of FOAFM.The validity of FOAFM results is validated by comparing its results with the results available in the literature. It is observed that FOAFM is simply applicable,contains less computational work,and is fastly convergent.The convergence and stability are obtained with the help of optimal constants.FOAFM is very easy in applicability and provides excellent results at the first iteration for complex nonlinear initial/boundary value problems.FOAFM contains the optimal auxiliary constants through which we can control the convergence as FOAFM contains the auxiliary functions D1,D2,D3...in which the optimal constants G1,G2,...and the control convergence parameters exist to play an important role in getting the convergent solution which is obtained rigorously.The computational work in FOAFM is less when compared to other methods and even a low-specification computer can do the computational work easily.

KEYWORDS FOAFM;exact solutions;FWE;Caputo operator;HPM

1 Introduction

Fractional calculus deals with the operation of integer order calculus.Earlier fractional calculus has assumed to have no physical applications, but later scientists proved that fractional calculus has many applications in real-world problems such as sound waves propagation in rigid porous material [1], ultrasonic wave propagation in human bone [2], viscoelastic properties in biological tissues[3],and tracking in automobiles[4].Recently fractional calculus has attracted the attention of researchers due to its vast applications in the field of electromagnetic,physics,viscoelasticity,material science,fluid mechanics,and applied sciences[5-9].The exact solution has an important role in the solution of fractional calculus.Since most of the Partial Differential Equations(PDEs)have no exact solutions, therefore, the scientist strives for various methods like transformation base methods [10-13],Vibrational iteration method VIM[14],Adomian decomposition method(ADM)[15],Homotopy Perturbation Method (HPM) [16], Differential Transform Method (DTM) [17] to treat the PDEs with no exact solutions. This method required a small assumed parameter or the initial guess. The improper selection of these choices affects the accuracy. The idea of homotopy was introduced in the Perturbation Method(PMs)to develop the Homotopy Perturbation Method(HPM)[18-20]and Homotopy Analysis Methods(HAM)[21]to fix the issue of a small parameter.These methods require the initial guess and have larger flexibility to control the convergence region. To overcome the issue of initial guess,Marinca and Heri¸sanu et al.introduced the Optimal Homotopy Asymptotic Method(OHAM)[22-26].This method contains the optimal auxiliary function and does not require the initial guess and is hence extended by Ullah et al.[27-31]to more complex models.Herisanu 2019 introduced the Optimal Auxilary Function Method(OAFM)[32]to handle the nonlinear problem.This method is introduced for less computational work and an accurate solution is obtained at the first iteration.Abbas bandy used the HAM for the nonlinear model[33].Kumar et al.used fractional derivative with Mittag-Leffler-type kernel for the FEW problems[34].Lu used the VIM for the solution of FEW[35]whereas FEW is solved by Group invariant solutions and conservation laws by Heshemi et al. [36].Ramadan et al.[37]used the new iterative method and compared its results with HPM results for the FEW models.Merdan et al.[38]used numerical simulations to handle FEW problems.Wang et al.[39]used the modified fractional homotopy analysis transform method for obtaining the FEW solutions.Abidi et al.[40]used the numerical procedure for the solution of the FEW models.The researchers used the semi-analytical methods and numerical methods equally. The numerical methods required large computational memory and processing time for getting the solutions of nonlinear fractional PDEs.The numerical methods required the linearization and discretization procedure and these procedures sometimes affect the accuracy of the methods that is why the scientists used the analytical method to solve the nonlinear fractional PDEs.The applications of these methods can be seen in[41-53].

The purpose of this paper is to modify the OAFM for fractional-order PDEs.FOAFM has been proven effective and is a reliable method to treat complex fractional-order PDEs.

The paper is organized into six sections.Section 1 is dedicated to the introduction.Basic concept and definitions are given in Section 2.The mathematical theory of FOAFM is given in Section 3,the applications of FOAFM to FWEs are given in Section 4.The results,discussion and conclusion are presented in Sections 5 and 6,respectively.

2 Some Basic Definitions

Definition 1.A real valued functionf(η),η≻0 is in space ifBη,η∈R, for a real numberη≺p,f(η)=ηpf1(η),wheref1(η)∈B(0,∞)and is in space iff n(η)∈Bη,n∈N

Definition 2.The Reiman-Liouville fractional integral operator

Definition 3.The fractional derivative of the function,f(u),in the Caputo sense

Definition 4:Ifn-1 ≺α≤n,n∈N and f∈Bnη,η≥-1,then

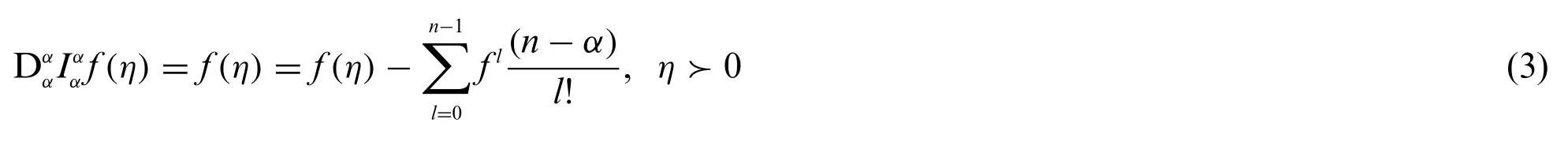
3 Analysis of OAFM for Fractional Order PDEs

Let us see the OAFM to nonlinear ODE

The initial conditions are

Selecting

Using Eq.(6)in Eq.(4),we obtain

The zeroth approximation is determined as

The first approximation is obtained as

since Eqs.(7) and (8) contain the time fractional derivatives, hence by applyingIαthe operator, we obtain

and

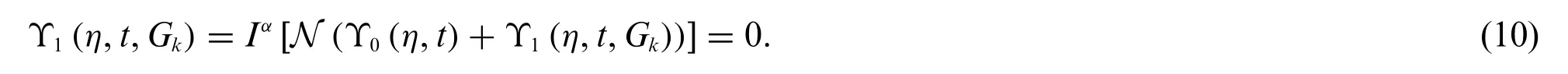
The nonlinear term is expressed as

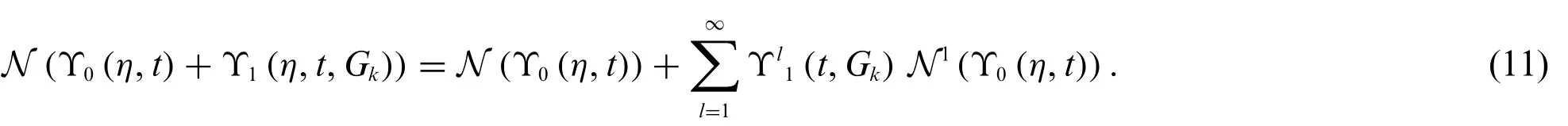
Eq.(11)can be written as

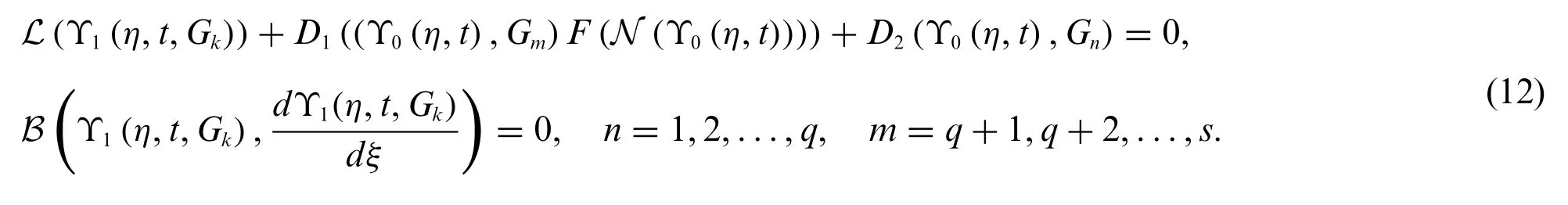
Convergence of the Method:The optimal constants are obtained by using the Method of Least Squares:

whereIis the equation domain.

The unknown constants are established as

Using the values of Es,we find the approximated solution as

4 Implementation of the Method

In this section,we implement the mathematical formulation of OAFM to FWE models.

Problem 1:Consider the FEW of the form

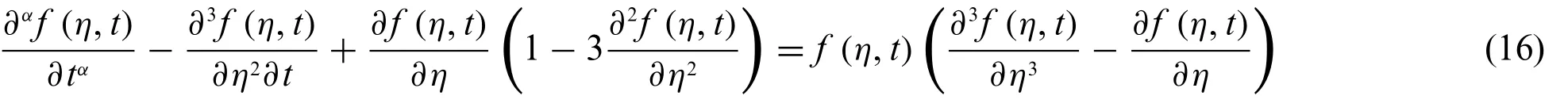
with

wheref (η,t)represents the fluid velocity,η,trepresents the spatial time respectively andαis the order of the fractional.

The exact solution of Eq.(16)is given by[36]

where A is an arbitrary constant.

We consider

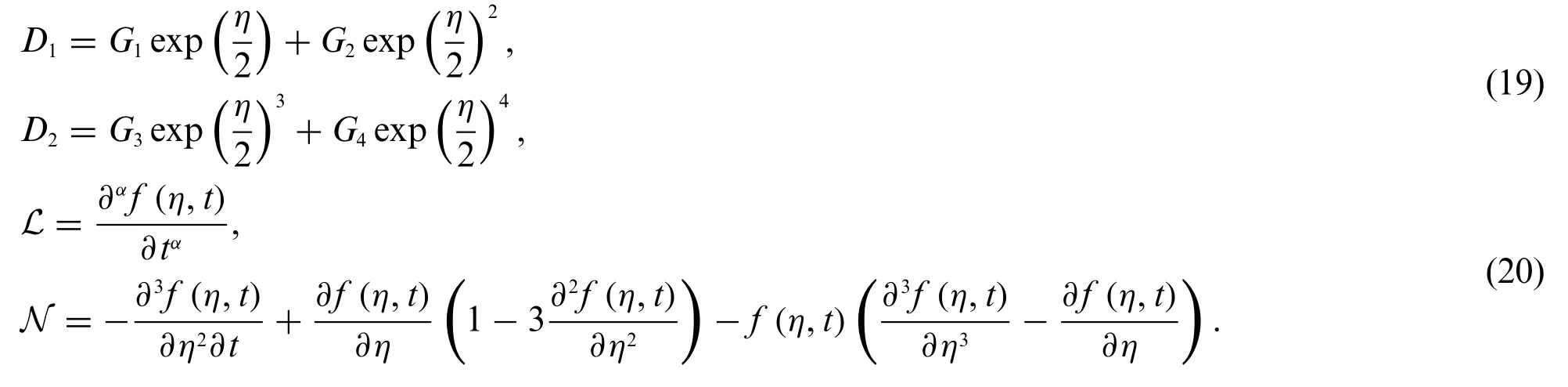
Zeroth Order System:

with initial conditions

Its solution is

First Order System:

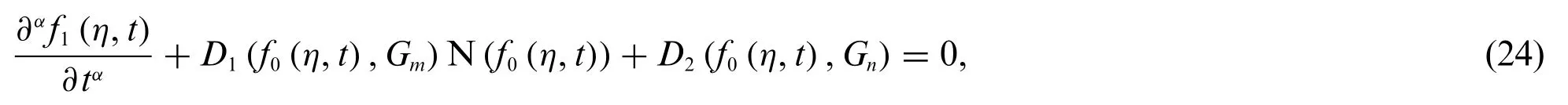
with

Using Eqs.(19)and(23)in Eq.(24),its solution is given by

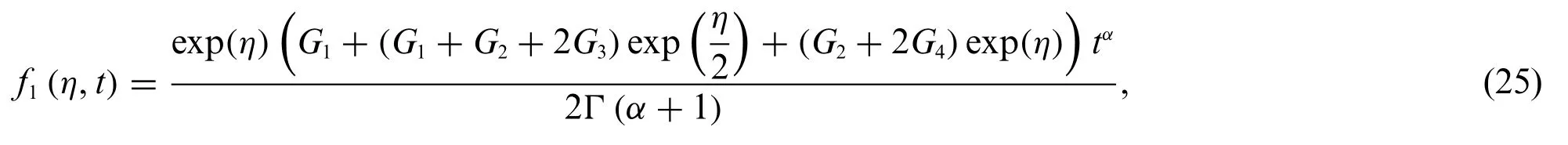
The final solution is given by

Applying the method of least square as discussed in Eqs.(13) and(14),we get the values of the optimal constants

We get

Problem 2:Consider the FEW of the form

with

The exact solution of Eq.(16)is given by[39]

We consider

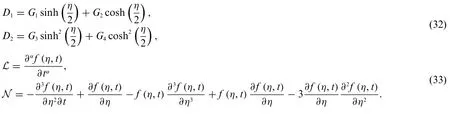
Zeroth Order System:

with initial conditions

Its solution is

First Order System:

Using Eqs.(32)and(36)in Eq.(37),we get

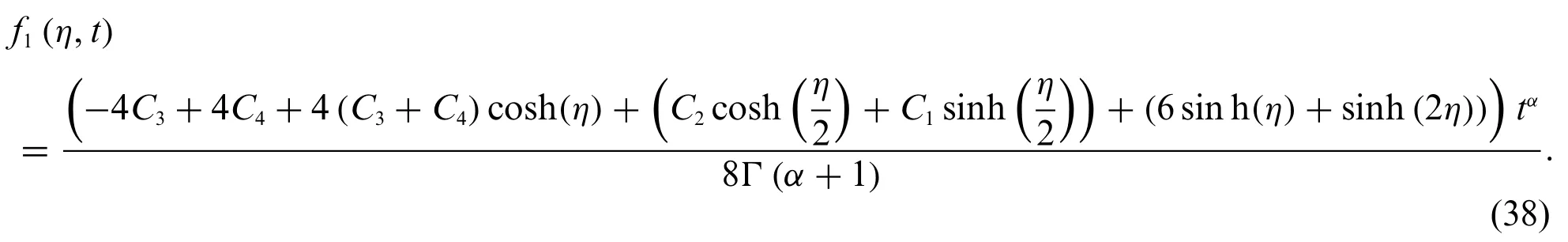
The final solution is given by

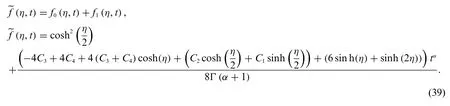
Applying the method of the least square as discussed in Eqs.(13) and(14), we get the values of the optimal constants

5 Results Analysis and Discussion

The mathematical theory of FOAFM provides highly accurate solutions for the fractional order Fornberg-Whitham equation as presented in Section 3.We have used Mathematica 11 for our computational work. The results obtained by FOAFM are compared with other methods available in the literature as given in Tables 1 and 2 for both the problems along with the exact solution revealing that FOAFM is valid and more accurate than other analytical methods as FOAFM provides nearly identical results to exact solutions.The absolute errors AEs for both problems are obtained in comparison with exact solutions for different values of fractional orderαas given in Tables 3 and 4.It is clear from Tables 3 and 4 that when the valueαapproaches to unity,then FOAFM solution rapidly converges to exact a solution which again validates our method.The optimal constants for the problem 1 is achieved by using the method of least square and is given as G1= -5.847118213776651, G2= -14.401838452215966, G3=12.988279917065954, G4=6.390562426483602, whereas the optimal constants of the problem 2 are again achieved by the method of least square and are given as C1= -0.176020345315655812, C2= -0.04833000998550175, C3= -1.0800781345410362, C4=0.17868188928874937.

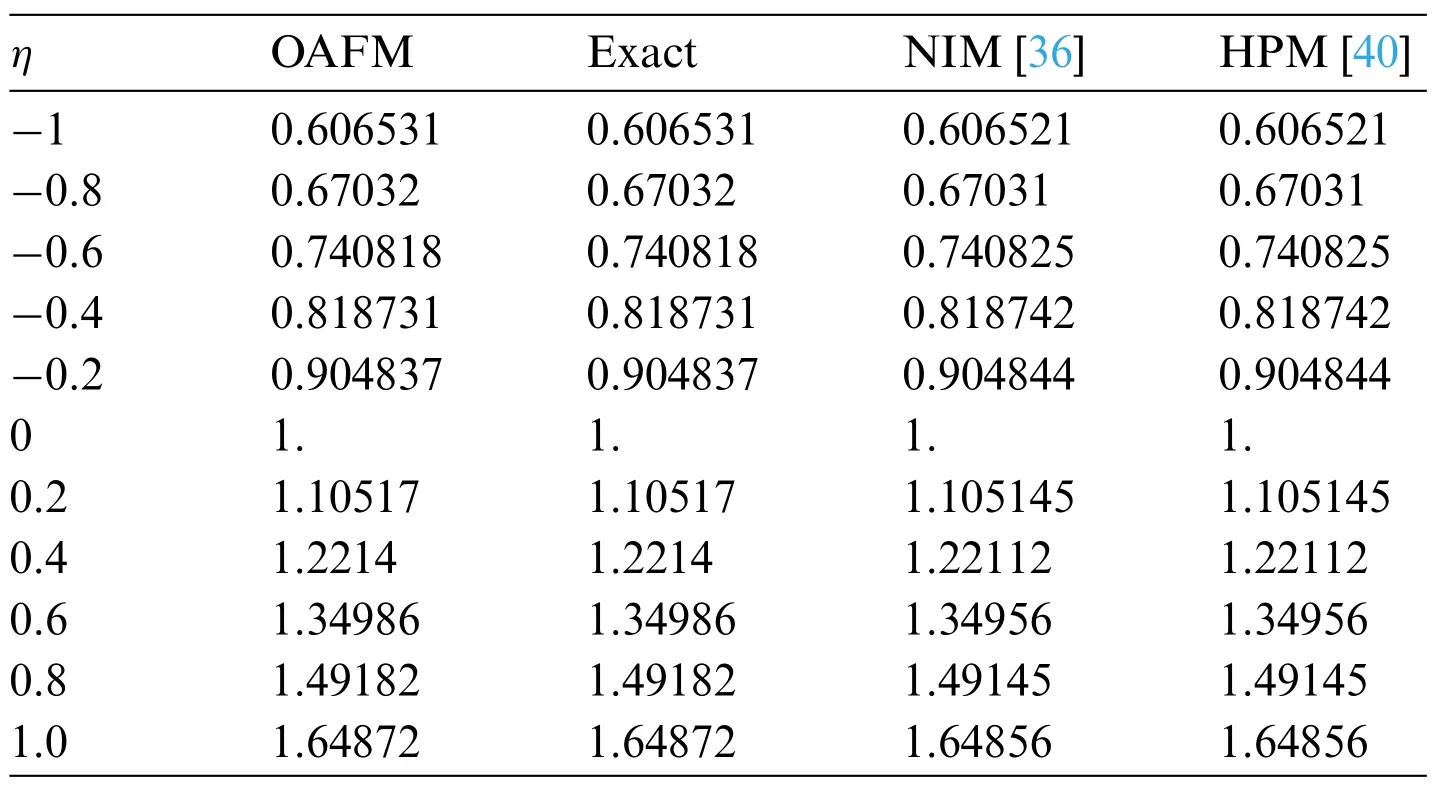
Table 1: Comparison of solutions

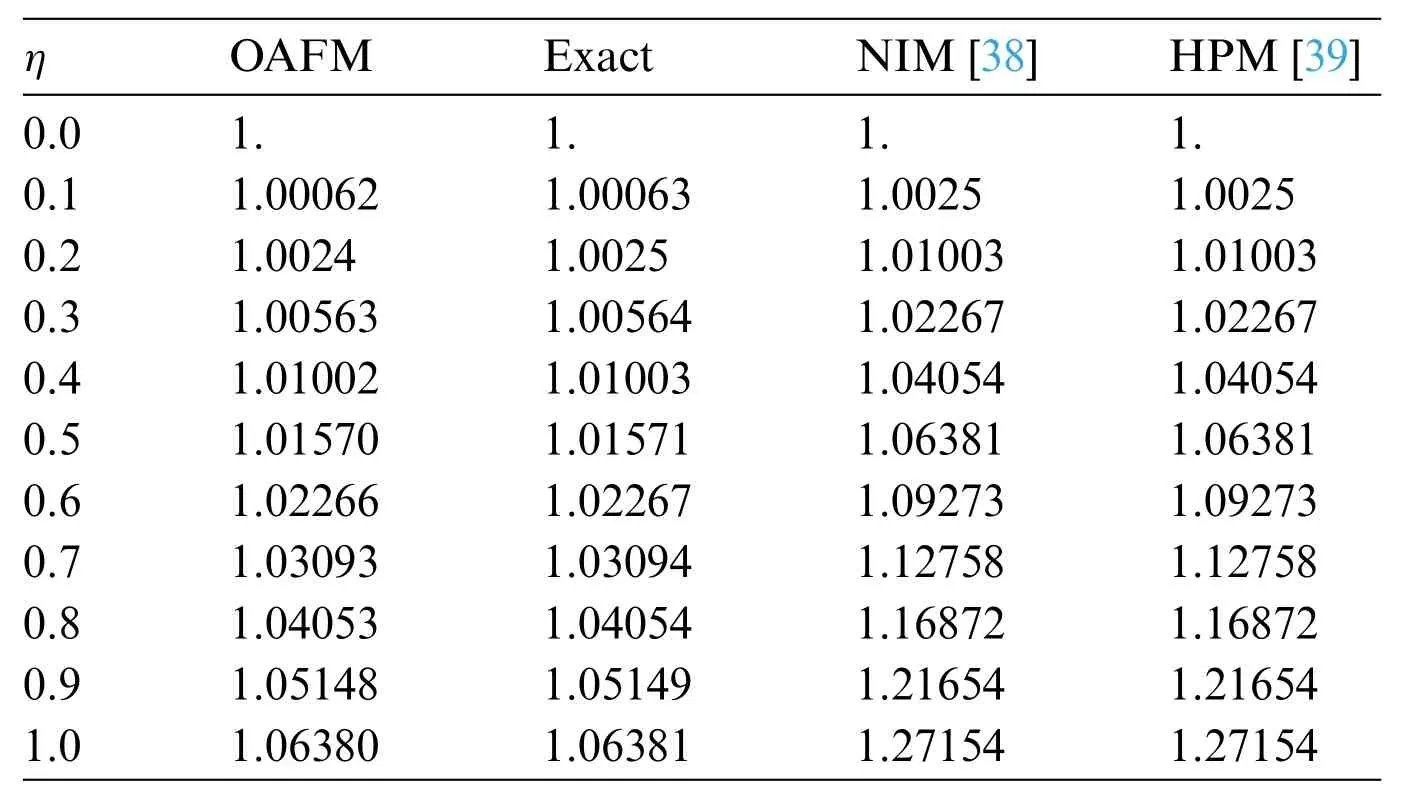
Table 2: Comparison of solutions

Table 3: AE =

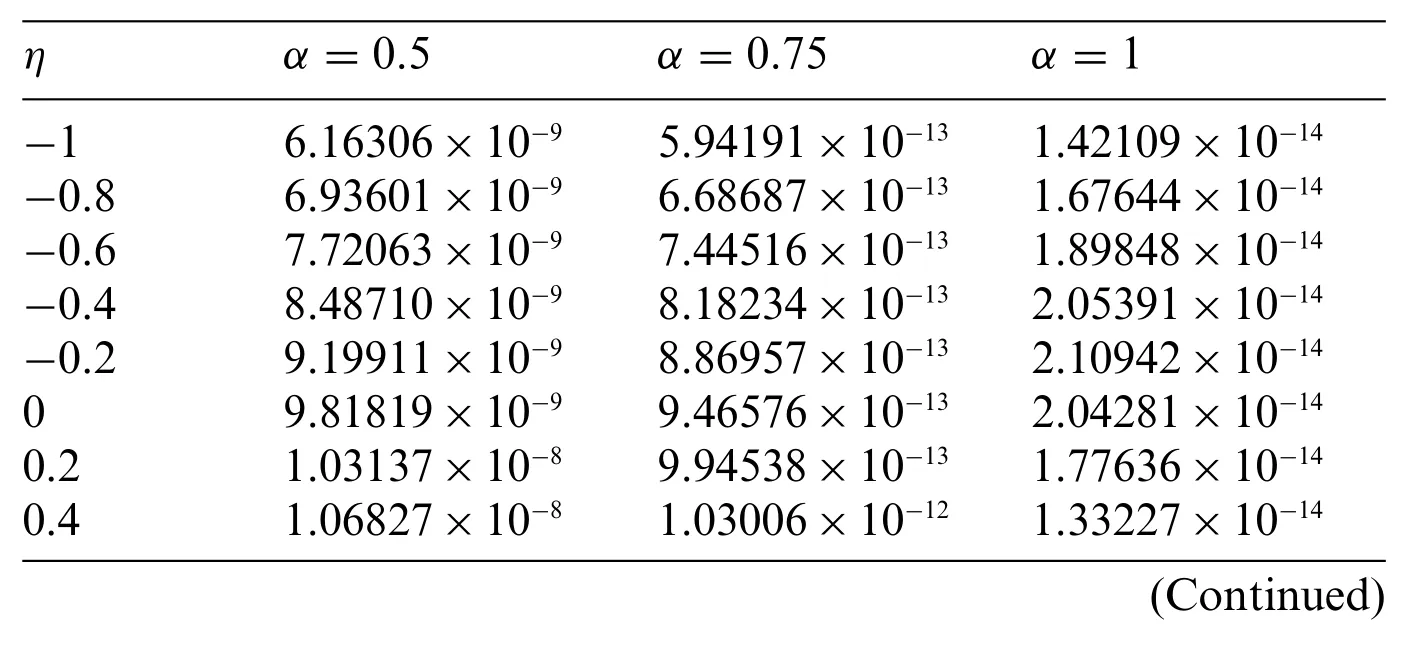
Table 3: AE =

?

?

Table 4:

Table 4:

?

The solution is again validated by comparing the solutions with exact solutions in 3D and 2D forms for problems 1-2 as given in Figs.1-3 and 4-6.The residual plots in 3D and 2D form are given in Figs.7, 8, 9 and 10 for problems 1 and 2, respectively, while the variation of fractional orderαis checked for accuracy in comparison with the exact solution for both the problems in Figs.11 and 12 consecutively.From Tables 3 and 4 and Figs.11 and 12,it is evident that when the value ofαis closer to 1,then the absolute error decreases andα=1 when used in the FOAFM.We get the closest result to the exact solution validating the accuracy of our method.

Figure 1: 3D plot ofη,t)

Figure 2: 3D exact solution of f (η,t)

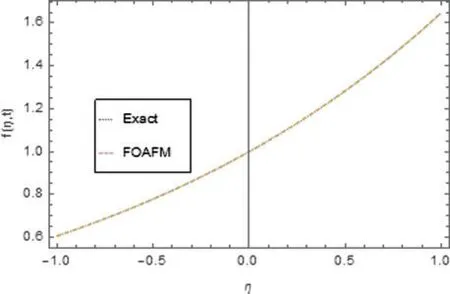
Figure 3: 2D plot of comparison of exact and FOAFM solutions

Figure 4:3D plot of (η,t)

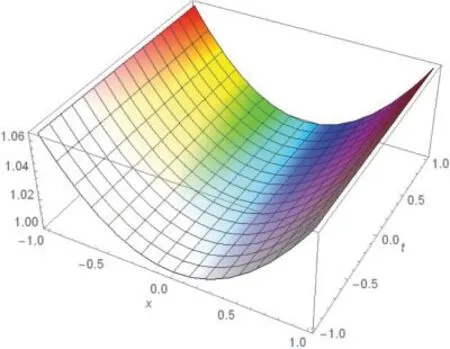
Figure 5: 3D exact solution of f (η,t)

Figure 6: 2D plot of comparison of exact and FOAFM solutions

Figure 7:3D plot of residual

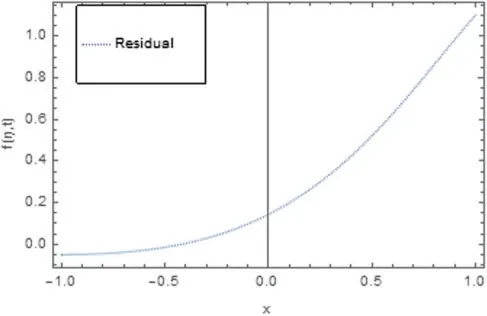
Figure 8:2D plot of residual

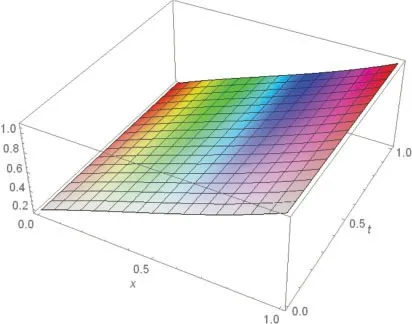
Figure 9: 3D plot of residual

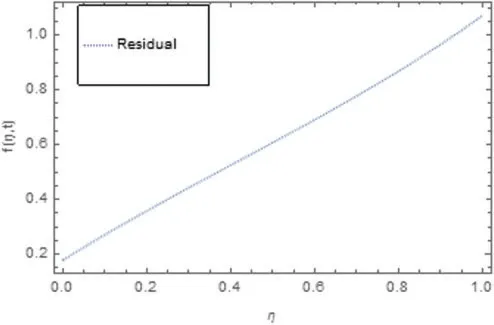
Figure 10: 2D plot of residual

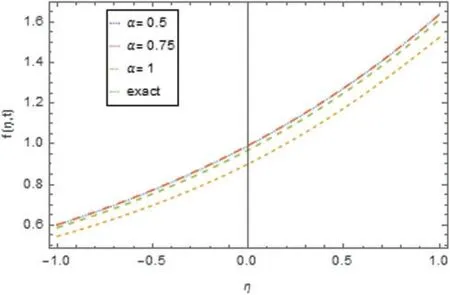
Figure 11: 2D plot of approximate solution for α

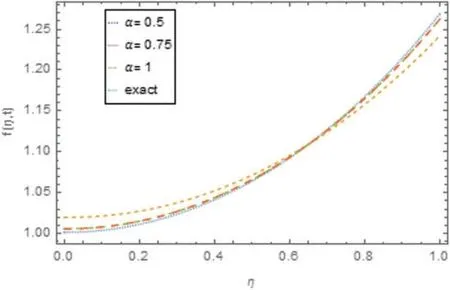
Figure 12: 2D plot of approximate solution for α

6 Conclusion

In this study, a new analytical method is suggested for the solution of the Fornberg-Whitham equation.We obtained the first order series solution for the Fornberg-Whitham equation and achieved the first-order solution with high accuracy.For the accuracy and validity of our method,we compared the FOAFM results with the results available in the literature and the exact results. From the comparison, it is concluded that the suggested method is very accurate and good agreement of our results with the numerical results proves the validity of our method. FOAFM is simply applicable to linear and nonlinear initial and boundary value problems. In comparison with other analytical methods, FOAFM is very easy in applicability and provides us with good results for more complex nonlinear initial/boundary value problems.FOAFM contains the optimal auxiliary constants through which we can control the convergence as FOAFM contains the auxiliary functionsD1,D2,D3...in which the optimal constantsG1,G2,...and the control convergence parameters exist to play an important role in getting the convergent solution that is obtained rigorously.The computational work in FOAFM is less when compared to other methods and even a low specification computer can do the computational work easily. The less computational work and rapid convergent solution at the first iteration enable us to implement this efficient method in our future work for more complex models arising from real-world problems.The numerical method required maximum space and time as compared to FOAFM which is a short method and very rapidly convergent.Numerical methods are required to have large computational work and require the latest computer for computational work.

Funding Statement:The authors received no specific funding for this study.

Conflicts of Interest:The authors declare that they have no conflicts of interest to report regarding the present study.