Entropies of the Y-Junction Type Nanostructures

Ricai Luo,Aisha Javed,Muhammad Azeem,Muhammad Kamran Jamil,Hassan Raza and Muhammad Yasir Ilyas

1School of Mathematics and Physics,Hechi University,Yizhou,456300,China

2Abdus Salam School of Mathematical Sciences,Government College University,Lahore,54000,Pakistan

3Department of Mathematics,Riphah Institute of Computing and Applied Sciences,Riphah International University,Lahore,54000,Pakistan

4School of Mathematical Sciences,College of Science and Technology,Wenzhou-Kean University,Wenzhou,325060,China

5Department of Physics,COMSATS University Islamabad,Lahore,54000,Pakistan

ABSTRACT Recent research on nanostructures has demonstrated their importance and application in a variety of fields.Nanostructures are used directly or indirectly in drug delivery systems,medicine and pharmaceuticals,biological sensors,photodetectors,transistors,optical and electronic devices,and so on.The discovery of carbon nanotubes with Y-shaped junctions is motivated by the development of future advanced electronic devices.Because of their interaction with Y-junctions,electronic switches,amplifiers,and three-terminal transistors are of particular interest.Entropy is a concept that determines the uncertainty of a system or network.Entropy concepts are also used in biology,chemistry,and applied mathematics.Based on the requirements,entropy in the form of a graph can be classified into several types.In 1955, graph-based entropy was introduced.One of the types of entropy is edgeweighted entropy.We examined the abstract form of Y-shaped junctions in this study.Some edge-weight-based entropy formulas for the generic view of Y-shaped junctions were created,and some edge-weighted and topological index-based concepts for Y-shaped junctions were discussed in the present paper.

KEYWORDS Y-shaped junctions;three-terminal junctions;nanostuctures;entropy;carbon nanotube

1 Introduction

The future of multi-terminal networks and electronic appliances is made possible by nanotube junctions or branched nanotubes as building blocks.There are several different types of nanotube junctions used in nanoelectronics,such as junction electrical properties in L,T,Y,and Mei et al.[1,2],mechanical stability [3], conduction mechanism and transport phenomena Kim [4], and thermal rectification[5].Instead of straight nanotubes,nanostructures created nanotube junctions which are expected to outperform plain nanotubes in terms of mechanical properties.An amalgamation of nanotubes is made to obtain nano-structures, as cited by Baughman et al.[6,7], which introduced techniques,methodologies,and concise discussions.

We will discuss Y-type junctions of carbon nanotubes in this work.The name implies that Yjunctions have the shape of a Y-alphabet and are formed by joining three nanotubes.These three nanotubes are joined at a point known as the branching point,which is depicted in Fig.1 and is made up of hexagons and six heptagons.These types of Y-junctions are symmetric if a pattern of hexagons surrounds each heptagon in a symmetric pattern and nanotubes are joined in the same Y-shape.

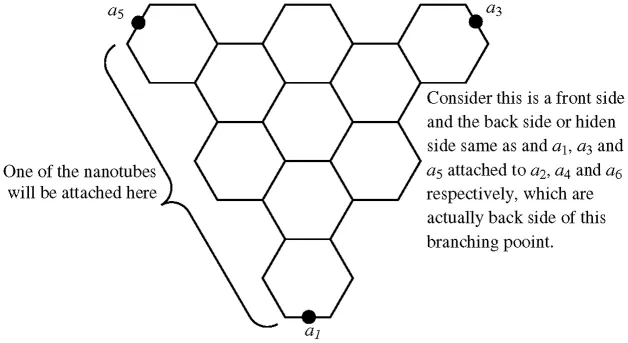
Figure 1:A branching point Y(6,6)

Y-junctions may have additional sub-types depending on the topology of nanotubes.Singlewalled nanotubes, for example, have three geometries: chiral, zig-zag, and armchair.So the created Y-junctions are known as the armchair carbon nanotube Y-junctions.Multi-walled nanotubes have all of these properties.The first nanotube was discovered in 1991 by Iijima[8].Two years later,the first structural topology of a Y-shaped junction was independently discovered by Scuseria [9] and Chernozatonskii[10].In 1995,Zhou et al.[11]observed these junctions experimentally.Biró et al.[12,13]presented the concept of asymmetric and symmetric Y-shaped junctions,their constructions,models,and numerous variants.Bandaru et al.[14,15]cited the applications and properties of novel structure Y-shaped junctions.

The quantitative structure-property and activity relationships are solely concerned with predicting bio-activities and chemical or biological structure properties.In this method,topological indices and physicochemical properties are used to aid prediction.There is a substantial body of literature on topological descriptors of chemical structure that can be found in Ahmad et al.[16-21].There are some basic formulas for predicting the physical properties of a network and system in the following section of this paper.

Definition 1.1Let a graph have edge and vertex sets notation,respectively.It is mandatory that the graph is connected,simple and planar.To compute our major results,we need to define some basics.For this,let a graph be with a vertex set and the second necessary component is edge set.The degree of a vertex set is the cardinality of incident edge with a vertex.There is another way to define edge set which is called edge partition,usually written as the degrees of vertices and an edge set.

Topological descriptors which are very essential to compute our major results of entropy are given in the following definitions.

Definition 1.2Gutman et al.[22,23]introduced the first and second Zagreb index as:

Definition 1.3The atom-bond-connectivity index is introduced by Estrada et al.[18]as:

“The entropy of a probability distribution is known as a measure of the unpredictability of information content or a measure of the uncertainty of a system”by Shannon [24].This viewpoint was founded on the concept of statistically analysing a graph, network, or structure.This idea was applied to the concept of the graph(on the vertex and edge components)in 1955,and it was dubbed graph-based entropy.Entropy is further subdivided into vertex-based,edge-based,and weight-based(on edge or vertex).This concept has numerous real-world applications in biology,ecology,and many other fields,as cited by Dehmer et al.[25]and Ulanowicz[26].For a more in-depth look at entropy and its applications,see Cao et al.[27-32].

The literature on the concept is limited and sparse because it is new and has received little attention.This entropy was also studied on different types of nanotubes found by Imran et al.[33,34].The atomic-bond-connectivity entropy of an edge-weight graph is presented by Kuwn et al.[35].Following that,we will present some basic formulations for edge-weight-based entropy.

The main purpose of the research is to investigate some edge-weight-based entropy of Y-shaped junctions and their variants,as well as some comprehensive applications on the conceptual and applied approach of Y-shaped junctions and their variants.In the following section, we will investigate the structure of Y-shaped junctions and their variants using a basic formulation of edge-weight-based entropy for any simple,loop-less graph.In addition,we will present some key findings on the edgeweight-based entropy of Y-shaped junctions and their variants.Finally, we will come to a decision.There would be some interesting and significant literary references to intrigue readers to investigate Y-shaped junctions and their variants, edge-weight-based entropy, topological index, and entropy applications.

Definition 1.4Chen et al.[36]developed the entropy for an edge weighted graph as:

with given weight for an edge.

Manzoor et al.[37,38] established the following entropies by using the main component of topological index as a weight of an edge.With the same pattern given below are some key mechanisms which are helpful to determine our main results of this work.Most importantly, all the below techniques are according to the mechanism defined in the Eq.(4).

Definition 1.5The entropies formulas of the first and second Zagreb indices are developed by Manzoor et al.[34,38]

Definition 1.6Similarly, the entropy formula of the atom bond connectivity is developed by Manzoor et al.[37]

2 Y-Junction Graphs and Methodology of Presented Work

For the purposes of computation, the Y-junction structures are transformed into vertex-edge graphs, as described by Diudea et al.[39,40].After obtaining the molecular graphs of junctions and their variants,the vertex-degree-based edge types are measured.These vertex-degree-based edge specifications are required tools for computing our main results.

The Y-junctions examined in this research are constructed by the covalent connectivity of three armchair single-walled carbon nanotubes of given size, crossed at an angle of 120 degrees, and ascertained by the chiral vector

Let the parameter be integers.A Y-shaped junction graph is assembled by making an armchair which is called as branching point, and three carbon nanotubes of the same length of-layers of hexagons.It has number of vertices and the edges are in total.It has degree three vertices, and only degree 2 vertices.Each nanotube comprises faces of hexagons.While branching point comprise of faces.In these faces, hexagons, six heptagons and only three faces where nanotubes joined with branching point.

The notation is referred to as a graph of Y-shaped junction and it contained no vertex of one degree.The notation is an extension of graph of Y-shaped junction shown in the Fig.2.This graph appears when one of the three nanotubes has exactly degree one vertices.The total number of vertices and edges are counted accordingly.The graph with notation is another extension of junction graph.It contains edge and vertices.This graph is obtained by having degree one vertices on any two nanotubes.The total degree one vertices attaching to all three nanotubes made another variant Y-junction.This newly developed graph contains edges and vertices.

The structure shown in the Fig.1 is a front view of branching point with three sides where armchair carbon nanotubes with different length will be attached.The structure shown in the Fig.2 is a variant of Y-junction having pendent or one degree vertices, and the notation is used.In the same figure, a place on a single nanotube,is pointed where the extension of one degree and vertices can be attached for different variants of Y-junctions and these variants are denoted.Without any one degree vertex,there will be the original Y-junction of notation.

Given below are some entropy measures for Y-junction and all its the variants.

Figure 2:A variant of Y-junction γ3β(γ,γ)

Theorem 2.1[29]Let γβ(γ,γ)be a structure of Y-junction witht≥2,γ=2t,β≥1,then

Theorem 2.2[41]Let γ1β(γ,γ)be a structure of first extension of Y-junction witht≥2,γ=2t,β≥1,then

Theorem 2.3[41]Let γ2β(γ,γ)be a structure of second extension of Y-junction witht≥2,γ=2t,β≥1,then

Theorem 2.4[41]Let γ3β(γ,γ)be a structure of third extension ofY-junction witht≥2,γ=2t,β≥1,then

Further some interesting topics and literature are given here for the readers attension.Some recent topological indices are discussed by Raza et al.[42].Recent and relevant topics are discussed by Alshehri et al.[43,44], and graph operations and their topological indices are discussed by Raza et al.[45,46].

Theorem 2.5Let γβ(γ,γ)be a structure of Y-junction witht≥2,γ=2t,β≥1, andEM1is the first Zagreb entropy,then is

Proof.The formula of topological descriptor of first Zagreb index is given by the Eq.(1), while the result of this index for the structure of Y-junction is defined in Eq.(8)of Theorem 2.1.Now,to measure the first Zagreb entropy for the structure of Y-junction,we use the Eq.(8)and edge sequence from the Eq.(20)in the formula of the first Zagreb entropy which is given in the Eq.(5).We will have the required result as following:

This concludes the required outcome.

Theorem 2.6Let γβ(γ,γ)be a structureofY-junction witht≥ 2,γ=2t,β≥1 andEM2is the second Zagreb entropy, thenis

Proof.The formula of topological descriptor of the second Zagreb indexM2is given by Eq.(2),while the result of this index for the structure of Y-junction γβ(γ,γ)is defined in Eq.(9) of Theorem 2.1.Now,to measure second Zagreb entropy for the structure of Y-junction γβ(γ,γ),we use Eq.(9)and edge sequence from Eq.(20)in the formula of the second Zagreb entropyEM2which is given in the Eq.(6).We will have the required result as following:

This concludes the required outcome.

Theorem 2.7Let γβ(γ,γ)be a structure of Y-junction witht≥2,γ= 2t,β≥1 andEABCis the atom-bond-connectivity entropy,thenis

Proof.The formula of topological descriptor of atom-bond-connectivity indexABCis given by the Eq.(3),while the result of this index for the structure of Y-junction γβ(γ,γ)is defined in Eq.(10)of Theorem 2.1.To measure atom-bond-connectivity entropy for the structure of Y-junction γβ(γ,γ),we use Eq.(10)and edge sequence from the Eq.(20)in the formula of atom-bond-connectivity entropyEABCwhich is given in the Eq.(7).We will have the required result as following:

This concludes the required outcome.

Theorem 2.8Let γ1β(γ,γ)be a structure of the first extension of Y-junction witht≥2,γ=2t,β≥1 andEM1is the first Zagreb entropy,thenis

Proof.The formula of topological descriptor of the first Zagreb indexM1is given by Eq.(1),while the result of this index for the structure of the first extension of Y-junction γ1β(γ,γ)is defined in Eq.(11)of Theorem 2.2.To measure the first Zagreb entropy for the structure of the first extension of Y-junction γ1β(γ,γ),we use Eq.(11)and edge sequence from Eq.(24)in the formula of the first Zagreb entropyEM1which is given in Eq.(5).We will have the required result as following:

This concludes the required outcome.

Theorem 2.9Let γ1β(γ,γ)be a structure of first extension of Y-junction witht≥2,γ=2t,β≥1and EM2is the second Zagreb entropy,thenis

Proof.The formula of topological descriptor of the second Zagreb indexM2is given by Eq.(2),while the result of this index for the structure of the first extension of Y-junction γ1β(γ,γ)is defined in Eq.(12)of Theorem 2.2.To measure the second Zagreb entropy for the structure of first extension of Y-junction γ1β(γ,γ), we use Eq.(12) and edge sequence from Eq.(24) in the formula of second Zagreb entropyEM2which is given in Eq.(6).We will have the required result as following.

This concludes the required outcome.

Theorem 2.10Let γ1β(γ,γ)be a structure of first extension of Y-junction witht≥2,γ=2t,β≥1 andEABCis the atom-bond-connectivity entropy,thenis

Proof.The formula of topological descriptor of atom-bond-connectivity indexABCis given by Eq.(3),while the result of this index for the structure of the first extension of Y-junction γ1β(γ,γ)is defined in Eq.(13)of Theorem 2.2.To measure atom-bond-connectivity entropy for the structure of the first type of Y-junction γ1β(γ,γ),we use Eq.(13)and edge sequence from Eq.(24)in the formula of atom-bond-connectivity entropyEABCwhich is given in Eq.(7).We will have the required result as following:

This concludes the required outcome.

Theorem 2.11Let γ2β(γ,γ)be a structure of second extension of Y-junction witht≥2,γ=2t,β≥1 andEM1is the first Zagreb entropy,thenis

Proof.The formula of topological descriptor of the first Zagreb indexM1is given by Eq.(1),while the result of this index for the structure of the second extension of Y-junction γ2β(γ,γ)is defined in Eq.(14)of Theorem 2.3.To measure the first Zagreb entropy for the structure of the second extension of Y-junction γ2β(γ,γ),we use Eq.(14)and edge sequence from Eq.(28)in the formula of the first Zagreb entropyEM1which is given in Eq.(5).We will have the required result as following.

This concludes the required outcome.

Theorem 2.12Let γ2β(γ,γ)be a structure of the second extension of Y-junction witht≥2,γ=2t,β≥1 andEM2is the second Zagreb entropy,thenis

Proof.The formula of topological descriptor of the second Zagreb indexM2is given by Eq.(2),while the result of this index for the structure of the second extension of Y-junction γ2β(γ,γ)is defined in Eq.(15) of Theorem 2.3.To measure the second Zagreb entropy for the structure of the second extension of Y-junction γ2β(γ,γ),we use Eq.(15)and edge sequence from Eq.(28)in the formula of the second Zagreb entropyEM2which is given in Eq.(6).We will have the required result as following:

This concludes the required outcome.

Theorem 2.13Let γ2β(γ,γ)be a structure of the second extension of Y-junction witht≥2,γ=2t,

β≥1 andEABCis the atom-bond-connectivity entropy,thenis

Proof.The formula of topological descriptor of atom-bond-connectivity indexABCis given by Eq.(3),while the result of this index for the structure of the second extension of Y-junction γ2β(γ,γ)is defined in Eq.(16)of Theorem 2.3.To measure atom-bond-connectivity entropy for the structure of the first type of Y-junction γ2β(γ,γ),we use Eq.(16)and edge sequence from Eq.(28)in the formula of atom-bond-connectivity entropyEABCwhich is given in Eq.(7).We will have the required result as following:

This concludes the required outcome.

Theorem 2.14Let γ3β(γ,γ)be a structure of the third extension of Y-junction witht≥2,γ=2t,β≥1 andEM1is the first Zagreb entropy,thenis

Proof.The formula of topological descriptor of the first Zagreb indexM1is given by Eq.(1),while the result of this index for the structure of the third extension of Y-junction γ3β(γ,γ)is defined in Eq.(17)of Theorem 2.4.To measure first Zagreb entropy for the structure of the third extension of Y-junction γ3β(γ,γ),we use Eq.(17)and edge sequence from Eq.(32)in the formula of the first Zagreb entropyEM1which is given in Eq.(5).We will have the required result as following:

This concludes the required outcome.

Theorem 2.15Let γ3β(γ,γ)be a structure of the third extension of Y-junction witht≥2,γ=2t,β≥1 andEM2is the second Zagreb entropy,thenis

Proof.The formula of topological descriptor of the second Zagreb indexM2is given by Eq.(2),while the result of this index for the structure of third extension of Y-junction γ3β(γ,γ)is defined in Eq.(18)of Theorem 2.4.To measure the second Zagreb entropy for the structure of the third extension of Y-junction γ3β(γ,γ),we use Eq.(18)and edge sequence from Eq.(32)in the formula of the second Zagreb entropyEM2which is given in Eq.(6).We will have the required result as following.

This concludes the required outcome.

Theorem 2.16Let γ3β(γ,γ)be a structure of the third extension of Y-junction witht≥2,γ=2t,β≥1 andEABCis the atom-bond-connectivity entropy,thenis

Proof.The formula of topological descriptor of atom-bond-connectivity indexABCis given by Eq.(3),while the result of this index for the structure of the third extension of Y-junction γ3β(γ,γ)is defined in Eq.(19)of Theorem 2.4.To measure atom-bond-connectivity entropy for the structure of the first type of Y-junction γ3β(γ,γ),we use Eq.(19)and edge sequence from Eq.(32)in the formula of atom-bond-connectivity entropyEABCwhich is given in Eq.(7).We will have the required result as following:

This concludes the required outcome.

3 Conclusion

Entropy is a concept that determines the uncertainty of a system or network.Entropy concepts are also used in biology,chemistry,and applied mathematics.Based on the requirements,entropy in the form of a graph can be classified into several types.In 1955,graph-based entropy was introduced.One of the types of entropy is edge-weighted entropy.We examined the abstract form of Y-shaped junctions in this study.We created some edge-weight-based entropy formulas for the generic view of Y-shaped junctions.We discussed some edge-weighted and topological index-based concepts for Y-shaped junctions.Entropies of Y-junctions and their variants are studied for the first time in this study.We calculated the atom-bond-connectivity,Zagreb’s first and second entropies for four different types of Y-shaped carbon nanotube junctions.Y-junctions and their structures are elaborated in numerical form using this method.The entire compound is described as a numeric digit.Instead of a complex structure,it will be easy to see as a number.

Data Availability:All the data supporting the results are included in the manuscript.

Funding Statement:This work was supported by the National Science Foundation of China(11961021 and 11561019),Guangxi Natural Science Foundation(2020GXNSFAA159084),and Hechi University Research Fund for Advanced Talents(2019GCC005).

Conflicts of Interest:The authors declare that they have no conflicts of interest to report regarding the present study.