Geometric discord of tripartite quantum systems

Chunhe Xiong(熊春河), Wentao Qi(齐文韬), Maoke Miao(缪茂可), and Minghui Wu(吴明晖)

1Interdisciplinary Center for Quantum Information,School of Physics,Zhejiang University,Hangzhou 310027,China

2School of Computer and Computing Science,Hangzhou City University,Hangzhou 310015,China

3School of Mathematical Sciences,Zhejiang University,Hangzhou 310027,China

4School of information and electrical engineering,Hangzhou City University,Hangzhou 310015,China

Keywords: geometric discord,tripartite quantum systems,quantum state discriminations,frozen discord

1.introduction

Quantum correlation is not only a unique phenomenon but also a valuable resource of the quantum world.The characterization and quantification of quantum correlations lie at the very heart of exploring and exploiting quantum phenomena.As resources, quantum correlations such as entanglement,[1]non-locality,[2,3]discord,[4]and coherence,[5]offer remarkable advantages that make quantum information theory more powerful than classical theory.[6-13]

Quantum discord, proposed firstly by Olliver and Zurek,[14]and Henderson and Vedral,[15]is the quantum correlation that arises from the loss of information caused by quantum measurements.Since then,a lot of efforts have been made to generalize discord to tripartite and larger systems.[16-20]Recently, Radhakrishnanet al.generalized quantum discord to multipartite cases.[21]A feature of their formulation is that the definition of multipartite discord reduces to the standard definition of discord for bipartite correlated subsystems,and this has also led to research of multipartite quantum discord.[22-26]

Quantifying discord is not only the basic problem of quantum correlation theory, but also the premise of using quantum discord.After Datta gave a mathematical description of bipartite quantum states with zero discord,[27]a great deal of work began on the quantitative study of quantum discord from a geometric perspective,that is,this kind of discord is defined as the minimal distance between a state and the set of states with zero discord.[28-31]

In this paper, we consider the quantification of tripartite discord with geometric measure, which has been proven to be a nice measure to quantify entanglement,[32]discord,[29,30]and coherence.[33]For pure states, we obtain the analytic expression of tripartite geometric discord.It is already known that geometric discord of pure state reduces to the geometric entanglement in bipartite systems.However,our results show that it is no longer the case in tripartite systems.

Furthermore, we provide an operational meaning for geometric tripartite discord by linking it to quantum state discrimination, that is, we prove that the geometric discord of tripartite states is equal to the minimum error probability to discriminate a set of pure states with von Neumann measurement.This conclusion generalizes the main result in Ref.[29],where the authors first established the relationship in the bipartite quantum system.Moreover,we obtain the analytic formula of geometric discord for three-qubit Bell diagonal states.

Finally, we consider the dynamics of tripartite geometric discord under local decoherence.It is interesting that the frozen phenomenon exists in the geometric discord of threequbit Bell diagonal states, since it has been shown that the original generalized discord of Bell diagonal states will always decay in this decoherence environment.[22]

The paper is organized as follows.We introduce and discuss the generalized geometric discord for tripartite systems in Section 2.We calculate the geometric discord of three-qubit Bell diagonal states and investigate the dynamic behavior in Section 3.Lastly,we present a conclusion in Section 4.

2.Geometric tripartite discord

2.1.Geometric discord of tripartite system

Let us first start by reviewing the original definition of discord,which is defined as

For tripartite systems, two local measurements will be necessary in order to destroy all the correlations.In general,each local successive measurement is conditionally related to the previous measurement, then the two-partite measurement is written as

wherea,b,andclabel the three subsystems,is a von Neumann projection operator on the subsystema,is a projector on subsystemb,conditioned on the measurement outcome ona.These states with zero discord of tripartite system have the following form:[21]

where{|αj〉a}jis a set of orthogonal basis,and for each fixedk,{|βk|j〉b}kis also a set of orthogonal basis.We call the quantum states with zero discord classically correlated and denote all of the classically correlated states of the tripartite system byCa:b:c.

WithCa:b:c,the geometric discord of tripartite state is defined as

2.2.Pure state case

Next,let us consider the quantification for pure states on the tripartite system.

Lemma 1 For tripartite pure state|ψabc〉, it can be decomposed as

whereµjk=µjµk|j.

With Lemma 1,we can derive the following result.

Theorem 2 For pure state|ψabc〉 with state decomposition as Eq.(3),then the geometric discord is given by

Proof See Appendix A.

In the bipartite system, it is well-known that geometric discord is equivalent to geometric entanglement on pure states.However,we find that it is not true for tripartite systems.

Example 1 Consider the symmetric state of three-qubit systems as follows:[32]

then the maximal entanglement eigenvalueΛmax=2/3 and the entanglement is

On the other hand, since the Schmidt decomposition between partyaand partybcis

we have

We can conclude that there exists a pure state of tripartite systems whose geometric discord is strictly less than geometric entanglement.

2.3.Mixed state case

Now, let us consider the mixed-state case.For bipartite state,Spehner and Orszag linked the Bures distance of discord to quantum state discrimination.[29]Let us consider the case of tripartite systems.

Theorem 3 For mixed stateρ ∈D(Habc), the geometric discord is equivalent to the minimal error to discriminate a set of quantum states with von Neumann measurement,that is,

Proof Firstly, the classically correlated stateσcc ∈Ca:b:chas the following form:

therefore,for anyρ,the fidelity between them is

Furthermore,one has

By using the Cauchy-Schwartz inequality, for fixedijone has

Note that Eq.(7)will be equality if the vectors|ψk|ij〉are orthogonal for differentij’s and each of them can be decomposed into the sum of product states.First, we show that the vectors|ψk|ij〉are orthogonal provided that the|Φijk〉are chosen appropriately.Actually,for an arbitrary orthonormal basis|Φijk〉ofHabc,let us consider the Hermitian matrixS(ij)with coefficients given by the scalar productsS(ij)k,l=〈ψk|ij|ψl|ij〉.SinceS(ij)is a Hermitian matrix,one can find a unitary matrixV(ij)such that (V(ij))†S(ij)V(ij)is orthogonal in the firstrijrows,whererijis the rank ofS(i j).Let| ˜Φijk〉=∑lV(ij)l,k|Φijl〉.Then| ˜Φijk〉is an orthonormal basis ofHand ∑k| ˜Φi jk〉〈 ˜Φijk|=Πij.Moreover,the vectors|˜ψk|ij〉=∑lV(ij)l,k|ψl|ij〉form an orthogonal set.

As a result,one finds

where the probabilityqij=〈αiβj|i|trc[ρ]|αiβj|i〉and the corresponding state

Furthermore,the geometric discord is given by

Noting that,ifρis invertible,then{ρij}ijwill be linearly independent.[29]In sequence,the result in Ref.[37]shows that the POVM maximizing the success probabilityPs(ρij,qij)is a von Neumann measurement with projectors,which implies

3.Three qubit Bell diagonal states and dynamics behavior

3.1.Three qubit Bell diagonal states

Let us calculate the geometric discord of the three-qubit Bell diagonal state,that is,

whose matrix form in computation basis is of the form(B2).

Let us first consider the geometric discord between subsystemaandbc.Based on the relationship between bipartite state discrimination and bipartite geometric discord,[29]one has

whereΛ=p1ρ1-p2ρ2.By using the minimax principle,[38]the maximum of tr(Π1Λ) over all projectorsΠ1of rank 4 is equal to the sum of the 4 highest eigenvaluesλ1≥···≥λ4of the Hermitian matrixΛ.

With the optimal probability of quantum state discrimination of two states,we can obtain

wherecmax:= max{|c1|,|c2|,|c3|}.Furthermore, since the closest classical correlated state of the above equation is actually inCa:b:c(see Appendix B),it holds

for each three-qubit Bell diagonal stateρabc.

3.2.Dynamic of geometric discord under local channels

For the initial state of Eq.(B1),the time evolution of the total system under the phase flip channel is given by

Fig.1.The case of c1=0.3,c2=0.4,c3=0.8,and γ =0.1.

4.Conclusion

In summary, we have obtained the analytic formula for geometric discord of tripartite systems and found that the geometric discord of pure state is not equal to the geometric entanglement for symmetric state of three-qubit systems,which implies that quantum discord and entanglement have a more complicated relationship in multipartite systems.

Furthermore, we provide an operational meaning for geometric discord of tripartite systems by linking it to quantum state discrimination, and calculate the geometric discord for generalized Bell diagonal states of three-qubit systems.

Lastly,we investigate the dynamic behavior of geometric discord of three-qubit systems under local decoherence and show that the frozen phenomenon exists for geometric discord of three-qubit systems.

Acknowledgments

Project supported by the National Natural Science Foundation of China(Grant No.12201555)and China Postdoctoral Science Foundation(Grant No.2021M702864).

Appendix A:Proof of Theorem 2

Proof Denote the classically correlated state

wherepjkl ≥0 and ∑pjkl=1.Moreover,|αj〉is an orthogonal basis ofHa,and for fixedj,|βk|j〉is an orthogonal basis ofHb,and|αjβk|jγl|jk〉is an orthogonal basis ofHcfor eachjk.Then,the square of fidelity between|ψabc〉andσccis

where the inequality follows from the fact that ∑pjkl=1.Actually,denote=max{µk|j}for eachj,then

where the last two inequalities follow the Cauchy-Schwartz inequality.Denote the Schmidt vector of eachby, then the closest classical correlated state is a pure state

Appendix B: Geometric discord of three-qubit Bell diagonal states

Let us calculate the geometric discord of the three-qubit Bell diagonal state,that is,

whose matrix form in computation basis is

As a result, if||c||=1,ρabcreduces to a pure state, and the geometric discord is given by Eq.(4).If||c||/=1,then we haveρabc ＞0 and

Let us first consider the geometric discord between subsystemsaandbc.Denote|α1(2)〉〈α1(2)|=(1/2)(I±uσ),then

whereξ=c1+ic2,η=u1+iu2.The eigenvalues of bothΛuandΓuare the same,that is,

Therefore,the fidelity betweenρabcand classically correlated states on the bipartite systemaandbcis

From Appendix C,one has

wherecmaxhas the maximal absolute value of{c1,c2,c3}.As a result,the geometric discord betweenaandbcis

(i) Assumecmax=c3, then the optimal measurement is{|0〉〈0|,|1〉〈1|}.Furthermore, the eigenspaces ofΓuare spanned by the following projections:

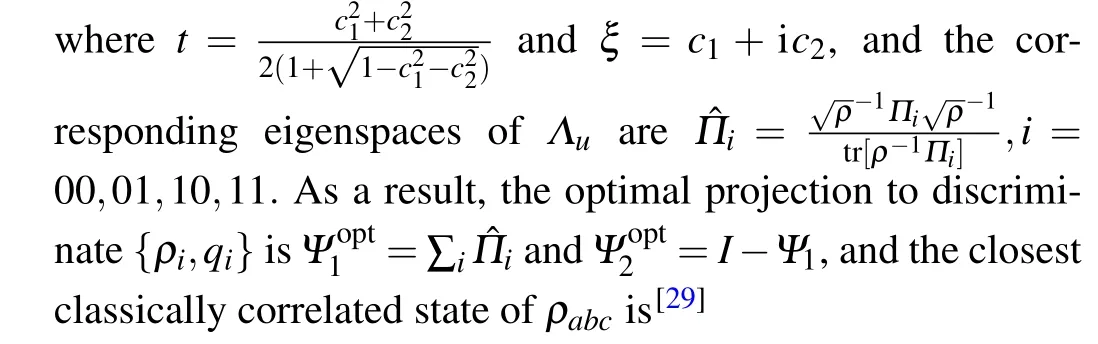
wherei,j=00,01,10,11.Sinceσ3)is a diagonal state, then the closest correlated stateρccis also a diagonal state,which meansρcc ∈Ca:b:c.In other words,for this kind of state,

(ii) Ifcmax=c1, it can be verified that the unitary matrixU=(1/2)(-I+iσ)satisfiesU†σ1U=σ3,U†σ2U=σ1,U†σ3U=σ2,therefore,

(iii) Ifcmax=c2, it can be verified that the unitary matrixU=(1/2)(I+iσ) satisfiesU†σ1U=σ2,U†σ2U=σ3,U†σ3U=σ1,therefore,

(iv) If the maximalcmaxis not unique, for example,|c1|=|c2|＞|c3|, then there are infinite optimal projections with|α0〉 = (1/2)(I+sc1σ1+(1-s)c2σ2),s ∈[0,1].As a result, the closest classically correlated state is not unique.Based on the above discussion,it also holds

Appendix C:Proof of the inequality

Letf=f1+f2+f3+f4with

the equality holds forf1=f2=f3=f4,which means that